If X={1,2,3,....,10}andA={1,2,3,4,5}. Then, the number of subsets B of X such that A−B={4} is
a)25b)24c)25−1d)1Solution in Malayalam
25
24
25−1
1
The correct Answer is:A
Step by step video, text & image solution for If X= {1,2,3,....,10} and A={1,2,3,4,5}. Then, the number of subsets B of X such that A-B ={4} is a)2^5b)2^4c)2^(5) -1d)1 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
The number of solutions of √3x2+x+5=x−3 is (A) 0 (B) 1 (C) 2 (D) 4
View SolutionIf x+2 is a factor of x2+ax+2b and a+b=4, then (a) a=1, b=3 (b) a=3, b=1 (c) a=−1, b=5 (d) a=5, b=−1
View SolutionLet A=[123−5] and B=[1002] and X be a matrix such that A=BX , then X is equal to (a) 12[243−5] (b) 12[−2435] (c) [243−5] (d) none of these
View SolutionLet X={1,2,3,…,10} and A={1,2,3,4,5} . The number of subsets of B of X such that A−B={4}, is ___________
View SolutionIf (log)10[12x+x−1]=x[(log)105−1] , then x= 4 (b) 3 (c) 2 (d) 1
View SolutionThe number of solutions of √3x2+x+5=x−3 is (A) 0 (B) 1 (C) 2 (D) 4
View Solutionmatch the columns
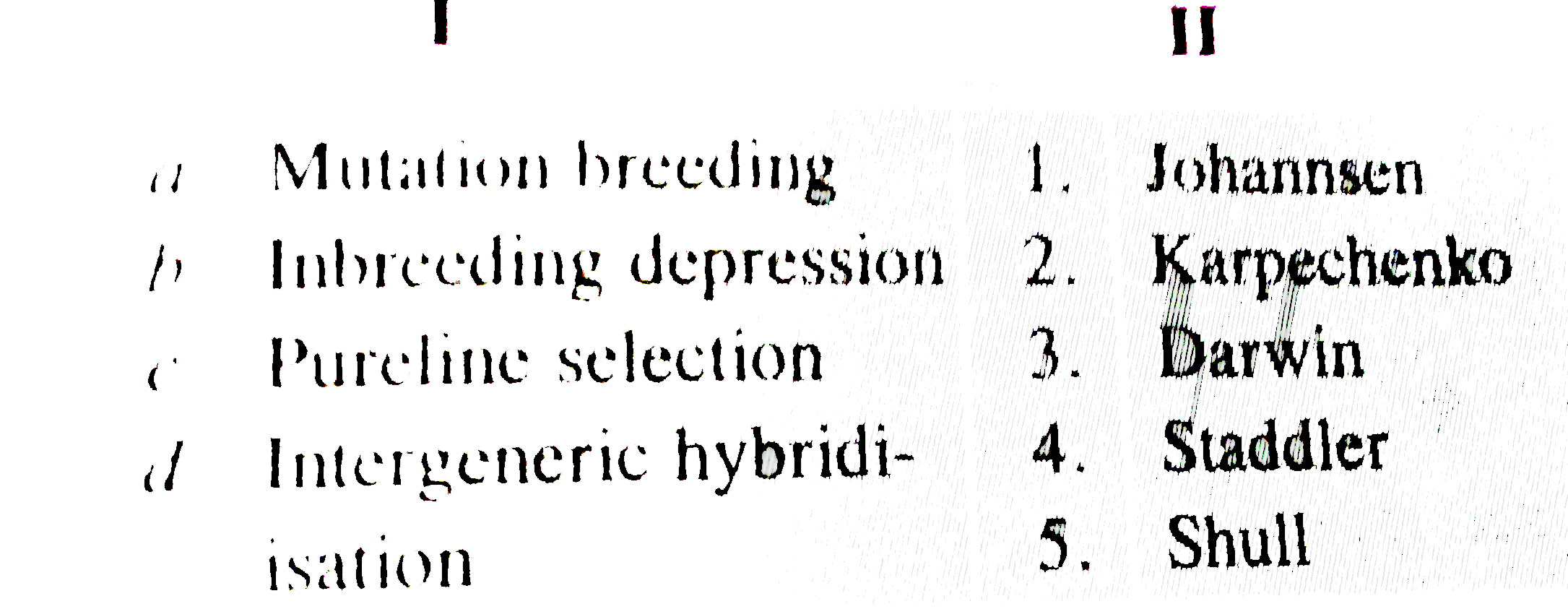
(a) a -4, b-2,c-5,d-1
(b) a-4,b-5,c-2,d-1
(c) a-4,b-5,c-1,d-2
(d) a-5, b-4,c-1,d-2
View Solution
