The shadow of a tower is found to be 60 m shorter when the sun's altitude changes from 30∘ to 60∘ . The height of the tower from the ground is approximately equal to : a)62 m b) 30√3 m c)101 m d)75 m Solution in Malayalam
62 m
301 m
101 m
75 m
Step by step video, text & image solution for The shadow of a tower is found to be 60 m shorter when the sun's altitude changes from 30 ^(@) " to " 60^(@) . The height of the tower from the ground is approximately equal to : a)62 m b) 30sqrt3 m c)101 m d)75 m by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
If the shadow of a tower is 30 m when the sun's altitude is 30∘ what is the length of the shadow when the sun's altiutude is 60∘?
View SolutionThe height of a tower is 15 m. The length of its shadow is 5√3 m when Sun's altitude is 30∘.
Is it True or False
View SolutionAt a particular time , when the sun's altitude is 30∘ , the length of the shadow of a vertical tower is 45 m . Calculate :
(i) the height of the tower ,
(ii) the length of the shadow of the same tower, when the sun's altitude is :
(a) 45∘ , (b) 60∘
View SolutionThe shadow of a tower standing on a level ground is found to be 40 m longer when the Sun's altitude is 30∘ than when it is 60∘. Find the height of the tower
View SolutionThe shadow of a tower standing on a level plane is found to be 50 m longer when when sun's elevation is 30∘ than when it is 60∘. Find the height of the tower.
View SolutionThe shadow of a tower standing on a level ground is found to be 40 m longer when sun's altitude is 30∘ then when it was 60∘. Find the height of the tower.
View SolutionThe shadow of a tower standing in a level plane is found to be 18 m shorter when the sun's altitude changes from 30∘ to 45∘. Show that the height of the tower is 9(1+√3)m.
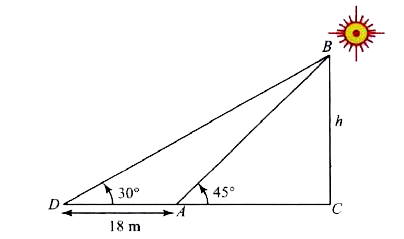
View SolutionThe shadow of a tower is found to be 60 ft. longer when the sun's altitude has become 60∘ from 30∘. The height of the tower from the ground is
View Solution
