A Carnot engine operating between temperatures T1 and T2 has efficiency 0.2. When T2 is reduced by 50 K, its efficiency increases to 0.4. Then, T1andT2 are respectivelySolution in Malayalam
200 K, 150K
250 K, 200 K
300 K, 250 K
300 K, 200 K
The correct Answer is:B
By Carnot.s ideal engine
η=1−T2T1
where ηi=0.2,η2=0.4
For the first condition
η(1)=1−T2T1
0.2=1−T2T1
or 0.2=T1−T2T1 ...(i)
For the second condition
η2=1−T2−50T1
0.4=T1−(T2−50)T1
0.4=T1−T2+50T1
=0.4=T1−T2T1+50T1
0.4=0.2+50T1 [From Eq. (i)]
0.4−0.2=50T1
T1=500.2
T1=250K
Putting the value of T1 in Eq. (i) we get
0.2=T1−T2T1
T2=T1=0.2T1
T2=250−0.2×250
T2=250−50
T2=200K
So, T1=250K,T2=200K
Similar Questions
A Carnot engine has an efficiency of 1/6. When the temperature of the sink is reduced by 62∘C, its efficiency is doubled. The temperature of the source and the sink are, respectively.
03:49View SolutionThree Carnot engines operate in series between a heat source at a temperature T1 and a heat sink at temperature T4 (see figure). There are two other reservoirs at temperature T2andT3, as shown, with T1>T2>T3>T4. The three engines are equally efficient if :
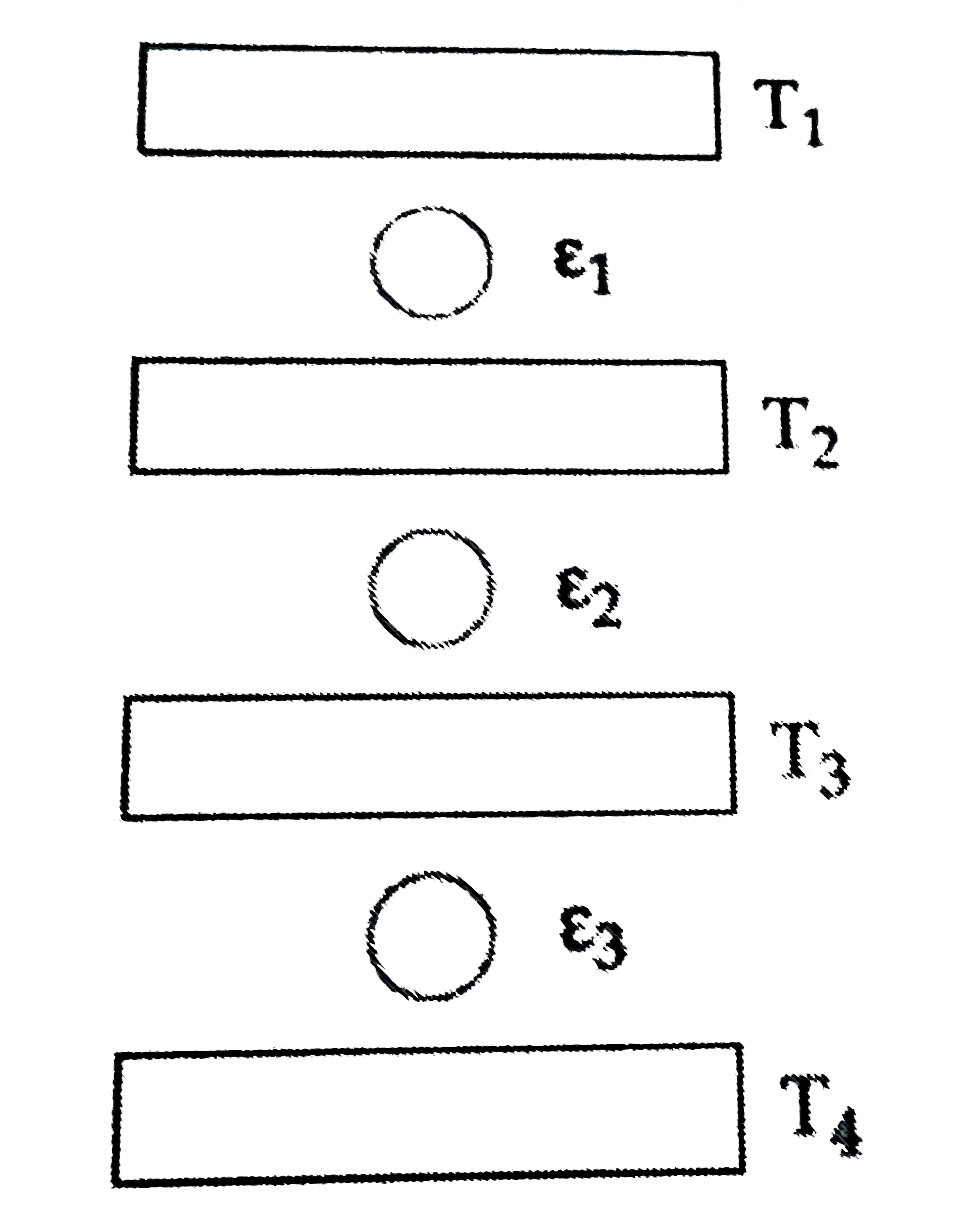 06:01View Solution
06:01View SolutionAn engine has an efficiency of 16. When the temperature of sink is reduced by 62∘C, its efficiency is doubled. Temperature of the source is
05:03View SolutionA carnot engine operating between temperatures T_1 and T_2 has efficiency 1/6 When T, is lowered by 62 K, its efficiency increases to 1/3 . Then T_1 and T_2 are, respectively:
03:03View SolutionAn ideal heat engine working between temperature T_(1) and T_(2) has an efficiency eta , the new efficiency if both the source and sink temperature are doubled, will be
02:08View SolutionThe efficiency of Carnot heat engine is 0.5 when the temperature of the source is T_(1) and that of sink is T_(2) . The efficiency of another Carnot's heat engine is also 0.5 . The temperature of source and sink of the second engine are respectively
02:29View SolutionEfficiency of an engine is eta1 at T1 =200 degree C and T2= 0 degree C and eta2 at T1 = 0 degree C and T2 = -200 degreeC find the ratio of eta1 / eta2
02:56View SolutionA carnot engine has an efficiency 0.4. When the temperature of the source is increased by 20^(@)C and the sink is reduced by 20^(@)C, its efficiency is found to increase to 0.5. Calculate the temperature of source and sink.
03:08View Solution

