Go back to Pascal’s triangle. Line 1 : 1 = 110 Line 2 : 1 1 = 111 Line 3 : 1 2 1 = 112 Make a conjecture about Line 4 and Line 5. Does your conjecture hold? Does your conjecture hold for Line 6 too?Solution in Punjabi
Step by step video & image solution for Go back to Pascal’s triangle. Line 1 : 1 = 11^0 Line 2 : 1 1 = 11^1 Line 3 : 1 2 1 = 11^2 Make a conjecture about Line 4 and Line 5. Does your conjecture hold? Does your conjecture hold for Line 6 too? by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
State whether the following statement is always true, always false or ambiguous. Justify your answers. There are 8 days in a week.
View SolutionA coin is tossed 1000 times with the following frequencies: Head : 455, Tail : 545 Compute the probability for each event.
View SolutionShow that each angle of a rectangle is a right angle.
View SolutionThe prejection of the line segment joining the poing (1,−1,3) and (2,−4,11) on the line joining the points (−1,2,3) and (3,−2,10), is ……
View SolutionEquations of the line through (4,−5,6) and perpendicular to the lines x−111=y+6−3=z−50and x−15=y−22=z−811 are
View SolutionGo back to Pascal's triangle.
Line 1:1=11∘
Line 2:1,1=111
Line 3:1,2,1=112
Make a conjecture about Line 4 and Line 5. Does your conjecture hold ? Does your conjecture hold for Line 6 too ?
View SolutionLook at the following pattern :
12=1
112=121
1112=12321
11112=1234321
111112=123454321
Make a conjecture about each of the following :
1111112=
11111112=
Check if your conjecture is true.
View Solutionरेखा x−13=y+64=z+12 को रखने वाले उस समतल का समीकरण ज्ञात कीजिए जो रेखा x−22=y−1−3=z+45 के समान्तर है।
View SolutionNote the Pascal triangle: line 1: 1 = 11^@ Row 2: 11 = 11^1 Row 3: 121 = 11^2 Create hypotheses for line 4 and base line 5. Check whether these hypotheses are sustainable. Similarly to line 6, test your hypothesis.
View SolutionGo back to Pascal's triangle . Line -1 : 1=11^(0) Line -2 : 11 = 11^(1) Line-3 : 121 = 11^(2) Make a conjecture about Line-4 and Line-5 . Does your conjecture hold ? Does your conjecture hold for Line -6 too ?
View SolutionLook at the following pattern: 1^(2) = 1 11^(2) =121 111^2 = 12321 1111^(2)=1234321 11111^2 = 123454321 Make a conjecture about about each of the following : 111111^2=.............. 1111111^2=........... Check if your conjecture is true .
View SolutionGo back to Pascal's triangle . Line -1 : 1=11^(0) Line -2 : 11 = 11^(1) Line-3 : 121 = 11^(2) Make a conjecture about Line-4 and Line-5 . Does your conjecture hold ? Does your conjecture hold for Line -6 too ?
View SolutionLook at the following pattern: 1^(2) = 1 11^(2) =121 111^2 = 12321 1111^(2)=1234321 11111^2 = 123454321 Make a conjecture about about each of the following : 111111^2=.............. 1111111^2=........... Check if your conjecture is true .
View SolutionLook at the following pattern:
12=1
112=121
1112=12321
11112=1234321
111112=123454321
Make a conjecture about about each of the following :
1111112=..............
11111112=...........
Check if your conjecture is true .
View SolutionGo back to Pascal's triangle .
Line -1 : 1=110
Line -2 : 11=111
Line-3 : 121=112
Make a conjecture about Line-4 and Line-5 .
Does your conjecture hold ? Does your conjecture hold for Line -6 too ?
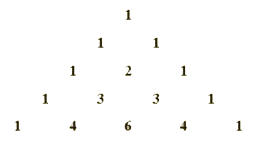
View SolutionLook at the following pattern:
12=1
112=121
1112=12321
11112=1234321
111112=123454321
Make a conjecture about about each of the following :
1111112=..............
11111112=...........
Check if your conjecture is true .
View Solution
