Evaluate 3.¯2−0.¯¯¯¯16
The correct Answer is:N/a
To evaluate 3.¯¯¯2−0.¯¯¯¯¯¯16, we can follow these steps:
Step 1: Convert the repeating decimals into fractions.
1. For 3.¯¯¯2:
Let x=3.¯¯¯2.
Then, x=3.2222....
To eliminate the repeating part, multiply both sides by 10:
10x=32.2222...
Now, subtract the first equation from this new equation:
10x−x=32.2222...−3.2222...
This simplifies to:
9x=29
Therefore,
x=299
2. For 0.¯¯¯¯¯¯16:
Let y=0.¯¯¯¯¯¯16.
Then, y=0.161616....
Multiply both sides by 100:
100y=16.161616...
Now, subtract the first equation from this new equation:
100y−y=16.161616...−0.161616...
This simplifies to:
99y=16
Therefore,
y=1699
Step 2: Perform the subtraction.
Now we need to subtract the two fractions:
3.¯¯¯2−0.¯¯¯¯¯¯16=299−1699
To subtract these fractions, we need a common denominator. The least common multiple of 9 and 99 is 99.
Convert 299 to have a denominator of 99:
299=29×119×11=31999
Now we can perform the subtraction:
31999−1699=319−1699=30399
Step 3: Simplify the fraction.
Now we simplify 30399:
303÷3=101and99÷3=33
So,
30399=10133
Step 4: Convert back to decimal form.
To convert 10133 back to decimal form, we can perform the division:
101÷33=3.060606...
This is equivalent to 3.¯¯¯¯¯¯06.
Final Answer:
Thus, the final answer is:
3.¯¯¯¯¯¯06
---
Let x=3.¯¯¯2.
Then, x=3.2222....
10x=32.2222...
Now, subtract the first equation from this new equation:
10x−x=32.2222...−3.2222...
This simplifies to:
9x=29
Therefore,
x=299
Let y=0.¯¯¯¯¯¯16.
Then, y=0.161616....
100y=16.161616...
Now, subtract the first equation from this new equation:
100y−y=16.161616...−0.161616...
This simplifies to:
99y=16
Therefore,
y=1699
3.¯¯¯2−0.¯¯¯¯¯¯16=299−1699
299=29×119×11=31999
31999−1699=319−1699=30399
303÷3=101and99÷3=33
So,
30399=10133
101÷33=3.060606...
This is equivalent to 3.¯¯¯¯¯¯06.
Thus, the final answer is:
3.¯¯¯¯¯¯06
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - NUMBER SYSTEMEXERCISE - Problems From NCERT/exemplar7videos
NUMBER SYSTEM
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - NUMBER SYSTEMEXERCISE - Exercise 1a5videos
NUMBER SYSTEM
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINES AND ANGLESEXERCISE - Revision Exercise (long Answer Questions )6videos
LINES AND ANGLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - POLYNOMIALSEXERCISE - Revision Exercise (short Answer Questions)16videos
POLYNOMIALS
Similar Questions
Knowledge Check
- Question 1 - Select One
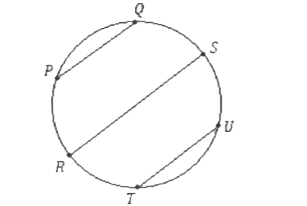
In the circle above with diameter d, chords ¯¯¯¯¯¯PQand¯¯¯¯¯¯TU are parallel to diameter ¯¯¯¯¯RS. If ¯¯¯¯¯¯PQand¯¯¯¯¯¯TU are each 34 of the length of ¯¯¯¯¯RS, what is the distance between chords ¯¯¯¯¯¯PQand¯¯¯¯¯¯TU in terms of d?Ad√78Bd√74Cπd4D3πd4
Evaluate :
2.¯7+1.¯3View SolutionEvaluate : (a) 0.9¯7(b)0.2345
View SolutionExpress each of the following recurring decimals into the rational number :
(i)0.¯5 (ii)2.¯4 (iii)1.¯¯¯¯12 (iv)2.7¯¯¯¯39 (v)0.¯¯¯¯¯¯516 (vi)3.7¯¯¯¯¯¯148View SolutionExpress each of the following as a fraction in simplest form : (i) 0.¯5 (ii) 1.¯4 (iii) 0.¯¯¯¯15
View SolutionWhich of the following is irrational?
(a)0.14
(b) 0.14¯¯¯¯16
(c)0.¯¯¯¯¯¯¯¯1416
(d) 0.1014001400014..View SolutionExpress each of the following recurring decimals into the rational number :
(i)0.¯7 (ii)0.¯6 (iii)1.¯3 (iv)3.¯8View SolutionExpress each of the following recurring decimals into the rational number :
(i)0.¯¯¯¯32 (ii)0.¯¯¯¯56 (iii)3.¯¯¯¯18 (iv)10.¯¯¯¯13View Solution

