3(1−x)<2(x+4)
The correct Answer is:N/a
To solve the inequality 3(1−x)<2(x+4), we will follow these steps:
Step 1: Distribute the terms in the inequality
We start by distributing the constants on both sides of the inequality.
3(1−x)<2(x+4)
This expands to:
3−3x<2x+8
Step 2: Rearrange the inequality
Next, we will move all terms involving x to one side and constant terms to the other side.
Add 3x to both sides:
3<2x+3x+8
This simplifies to:
3<5x+8
Now, subtract 8 from both sides:
3−8<5x
This simplifies to:
−5<5x
Step 3: Divide by 5
Now, we will divide both sides by 5 to isolate x:
−55<x
This simplifies to:
−1<x
Step 4: Write the final solution
We can rewrite the inequality as:
x>−1
In interval notation, this means:
x∈(−1,∞)
Final Answer:
The solution to the inequality 3(1−x)<2(x+4) is x>−1 or in interval notation, x∈(−1,∞).
---
We start by distributing the constants on both sides of the inequality.
Next, we will move all terms involving x to one side and constant terms to the other side.
Now, we will divide both sides by 5 to isolate x:
We can rewrite the inequality as:
The solution to the inequality 3(1−x)<2(x+4) is x>−1 or in interval notation, x∈(−1,∞).
Topper's Solved these Questions
- LINEAR INEQUALITIESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR INEQUALITIESEXERCISE - MISCELLANEOUS EXERCISE
14 Videos
- LINEAR INEQUALITIESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR INEQUALITIESEXERCISE - EXERCISE
76 Videos
- LIMITS AND DERIVATIVESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LIMITS AND DERIVATIVESEXERCISE - Miscellaneous Exercise
30 Videos
- MATHEMATICAL REASONING BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - MATHEMATICAL REASONING EXERCISE - Misellaneous exercise
7 Videos
Similar Questions
Knowledge Check
If 2x−3<x+1<4x+7,x∈R then the solution set of x on the number line is:
A B
B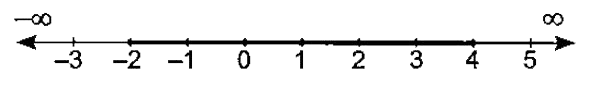 C
C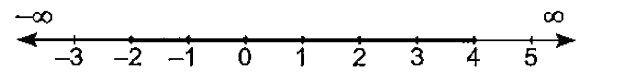 D
D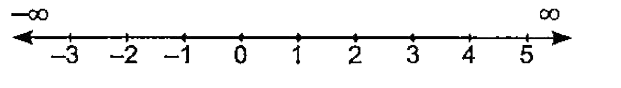
The equation x4−2x3−3x2+4x−1=0 has four distinct real roots x1,x2,x3,x4 such that x1<x2<x3<x4 and product of two roots is unity, then : x1x2+x1x3+x2x4+x3x4=
View SolutionFind the value of a,b if f(x)=⎧⎪ ⎪ ⎪ ⎪ ⎪⎨⎪ ⎪ ⎪ ⎪ ⎪⎩ae1/|x+2|−12−e1/|x+2|;−3<x<−2b;x=−2sin(x4−16x5+32);−2<x<0 is continuous at x=−2.
View SolutionThe equation x4−2x3−3x2+4x−1=0 has four distinct real roots x1,x2,x3,x4 such that x1<x2<x3<x4 and product of two roots is unity, then : x32+x34=
View SolutionSolve : 5x4>4x−13and4x+3<2x+13
View SolutionA function f is defined on the set of real numbers as follows:
f(x)=⎧⎪⎨⎪⎩x+11≤x<22x−12≤x<43x−104≤x<6
(a) Find the domain of the function.
(b) Find the range of the function.
View SolutionIf f(x)=x2−3x+4x2+3x+4, then complete solution of 0<f(x)<1, is :
View SolutionIf the median of the scores 1,2,x,4,5(where 1<2<x<4<5) is 3, then the mean of the scores is
View Solution
