The plane xa+yb+zc=1 meets the coordinate axes at A,B and C respectively. Find the equation of the sphere OABC.
x2+y2+z2+az+by+cz=0
x2+y2+z2+2az+2by+2cz=0
x2+y2+z2−ax−by−cz=0
None of these
The correct Answer is:C
To find the equation of the sphere OABC, where O is the origin and A, B, and C are the points where the plane xa+yb+zc=1 meets the coordinate axes, we will follow these steps:
Step 1: Find the coordinates of points A, B, and C
The plane intersects the coordinate axes at points A, B, and C.
- To find point A (where the plane intersects the x-axis), set y=0 and z=0:
xa+0+0=1⟹x=a⟹A(a,0,0)
- To find point B (where the plane intersects the y-axis), set x=0 and z=0:
0+yb+0=1⟹y=b⟹B(0,b,0)
- To find point C (where the plane intersects the z-axis), set x=0 and y=0:
0+0+zc=1⟹z=c⟹C(0,0,c)
Step 2: Find the center of the sphere
The center of the sphere OABC is the midpoint of the line segment joining the origin O(0, 0, 0) and the centroid of triangle ABC. The coordinates of the centroid G of triangle ABC can be calculated as:
G(a+0+03,0+b+03,0+0+c3)=(a3,b3,c3)
Thus, the center of the sphere is at the midpoint between O and G:
Center=⎛⎝0+a32,0+b32,0+c32⎞⎠=(a6,b6,c6)
Step 3: Calculate the radius of the sphere
The radius of the sphere is the distance from the center to any of the points A, B, or C. We can calculate the distance from the center to point A:
Radius=√(a−a6)2+(0−b6)2+(0−c6)2
Calculating this gives:
=√(5a6)2+(−b6)2+(−c6)2=√25a236+b236+c236=√25a2+b2+c236=√25a2+b2+c26
Step 4: Write the equation of the sphere
The equation of a sphere with center (h,k,l) and radius r is given by:
(x−h)2+(y−k)2+(z−l)2=r2
Substituting h=a6, k=b6, l=c6, and r=√25a2+b2+c26:
(x−a6)2+(y−b6)2+(z−c6)2=(√25a2+b2+c26)2
This simplifies to:
(x−a6)2+(y−b6)2+(z−c6)2=25a2+b2+c236
Final Equation of the Sphere
Thus, the equation of the sphere OABC is:
(x−a6)2+(y−b6)2+(z−c6)2=25a2+b2+c236
The plane intersects the coordinate axes at points A, B, and C.
xa+0+0=1⟹x=a⟹A(a,0,0)
0+yb+0=1⟹y=b⟹B(0,b,0)
0+0+zc=1⟹z=c⟹C(0,0,c)
The center of the sphere OABC is the midpoint of the line segment joining the origin O(0, 0, 0) and the centroid of triangle ABC. The coordinates of the centroid G of triangle ABC can be calculated as:
G(a+0+03,0+b+03,0+0+c3)=(a3,b3,c3)
Thus, the center of the sphere is at the midpoint between O and G:
Center=⎛⎝0+a32,0+b32,0+c32⎞⎠=(a6,b6,c6)
The radius of the sphere is the distance from the center to any of the points A, B, or C. We can calculate the distance from the center to point A:
Radius=√(a−a6)2+(0−b6)2+(0−c6)2
Calculating this gives:
=√(5a6)2+(−b6)2+(−c6)2=√25a236+b236+c236=√25a2+b2+c236=√25a2+b2+c26
The equation of a sphere with center (h,k,l) and radius r is given by:
(x−h)2+(y−k)2+(z−l)2=r2
Substituting h=a6, k=b6, l=c6, and r=√25a2+b2+c26:
(x−a6)2+(y−b6)2+(z−c6)2=(√25a2+b2+c26)2
This simplifies to:
(x−a6)2+(y−b6)2+(z−c6)2=25a2+b2+c236
Thus, the equation of the sphere OABC is:
(x−a6)2+(y−b6)2+(z−c6)2=25a2+b2+c236
Topper's Solved these Questions
- THREE-DIMENSIONAL GEOMETRY BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise 11.2
17 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise 11.3
14 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise 11 G
10 Videos
- RELATIONS AND FUNCTIONS BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Miscellaneous Exercise
19 Videos
- VECTORSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - VECTORSEXERCISE - Miscellaneous Exercise
19 Videos
Similar Questions
The plane xa+yb+zc=1 meets the co-ordinate axes in the point A, B, C respectively. Find the
area △ABC.
View SolutionA tangent and a normal to a curve at any point P meet the x and y axes at A, B and C, D respectively. Find the equation of the curve passing through (1,0) if the centre of circle through O.C,PandB lies on the line y = x (where O is the origin).
View Solution
Knowledge Check
A plane meets the coordinate axes at A, B and C respectively such that the centroid of triangle ABC is (p, q, r). The equation of the plane is
Axp+yq+zr=1Bxp+yp+zr=13Cpz+qy+rz=3Dxp+yq+zr=3
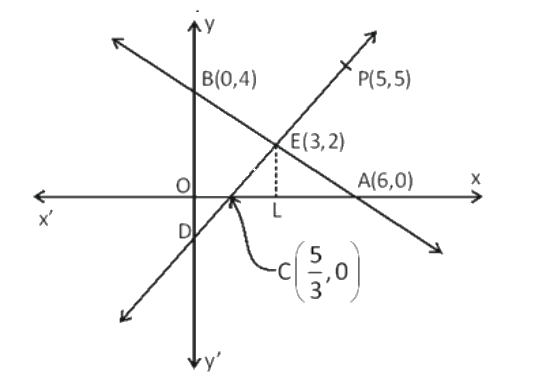
The line 2x+3y=12 meets the coordinates axes at A and B respectively. The line through (5, 5) perpendicular to AB meets the coordinate axes and the line AB at C, D and E respectively. If O is the origin, then the area (in sq. units) of the figure OCEB is equal to
View SolutionA plane meets the coordinate axes at A,BandC respectively such that the centroid of triangle ABC is (1,−2,3). Find the equation of the plane.
View SolutionA plane cuts the co-ordinate axes at A, B and C respectively. If the centroid of ΔABC is (2,−3,4), find the equation of the plane.
View SolutionIf the plane x2+y3+z6=1 cuts the axes of coordinates at points, A,B, and C, then find the area of the triangle ABC. a. 18 sq unit b. 36 sq unit c. 3√14 sq unit d. 2√14 sq unit
View SolutionThe plane x2+y3+z4=1 cuts the co-ordinate axes in A,B,C: then the area of the ΔABC is
View SolutionThe line xa+yb=1 meets the axis of and y at A and B respectively and C is middle point of AB then
View SolutionIf the plane x2+y3+z4=1 cuts the coordinate axes in A,B,C, then the area of triangle ABC is
View Solution
