Points $A (- 1, y)$ and $B (5, 7)$ lie on a circle with centre $O (2, - 3 y) .$ Find the values of $y .$ Hence, find the radius of the circle.

Video Solution

Text Solution

Verified by Experts

Here, $O A = O B$ (radii of a circle)
$\Rightarrow O A^{2} = O B^{2}$
$\Rightarrow {(2 + 1)}^{2} + {(- 3 y - y)}^{2} = {(2 - 5)}^{2} + {(- 3 y - 7)}^{2}$
$\Rightarrow 9 + 16 y^{2} = 9 + 9 y^{2} + 42 y + 49$

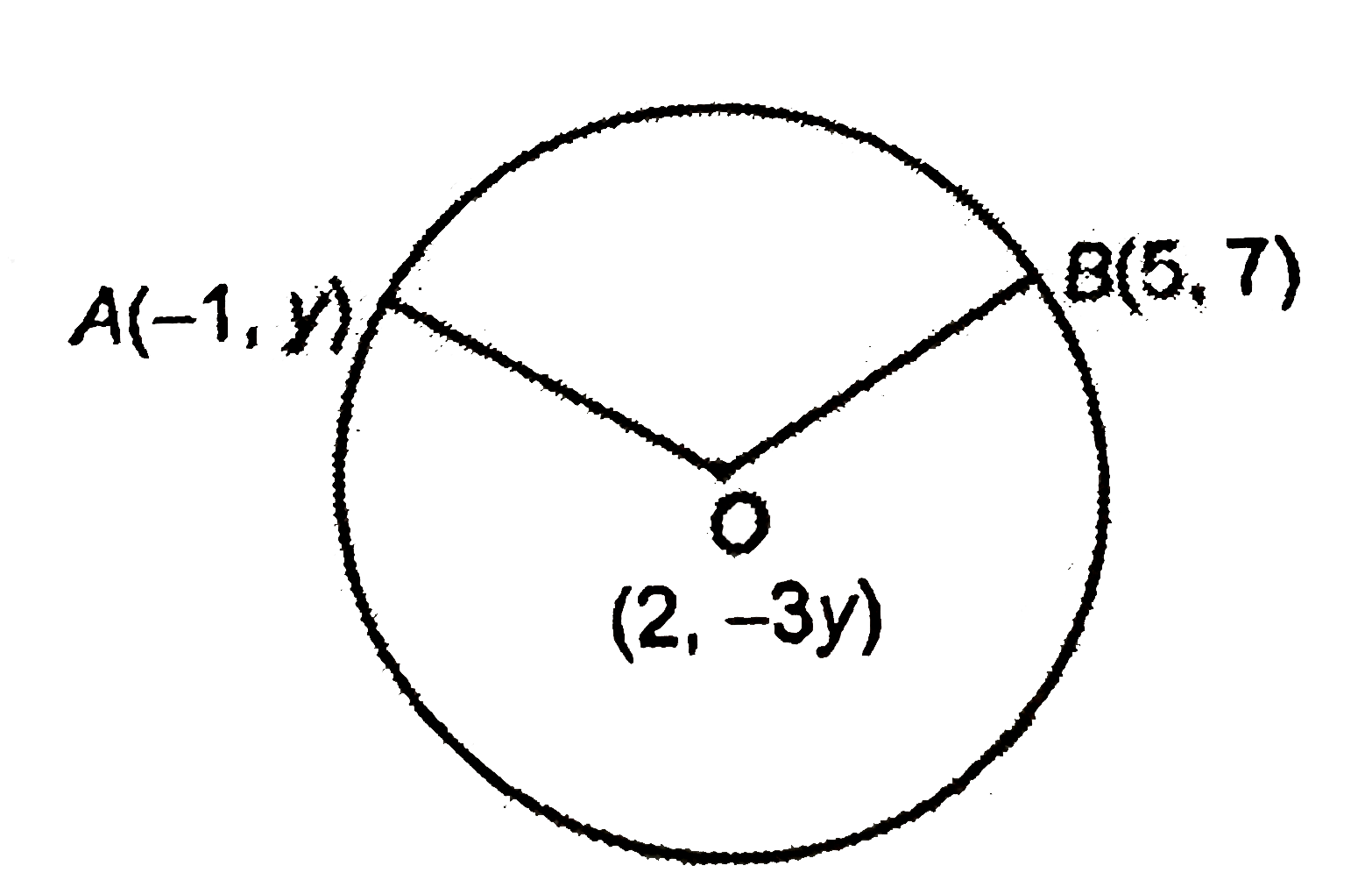
$\Rightarrow 7 y^{2} - 42 y - 49 = 0$
$\Rightarrow y^{2} - 6 y - 7 = 0$
$\Rightarrow y^{2} - 7 y + y - 7 = 0$
$\Rightarrow y (y - 7) + 1 (y - 7) = 0$
$\Rightarrow y - 7 = 0 or y + 1 = 0$
$\Rightarrow y = 7 or y = - 1$
Now, the co-ordinates of centre O are either (2, -21) or (2, 3) when centre is O(2, -21), then
$radius = O B$
$= \sqrt{{(2 - 5)}^{2} + {(- 21 - 7)}^{2}}$
$= \sqrt{9 + 784} = \sqrt{793}$ units
When centre is O(2, 3), then
$radius = O B$
$= \sqrt{{(2 - 5)}^{2} + {(3 - 7)}^{2}}$
$= \sqrt{9 + 16} = \sqrt{25} = 5$ units

|

Updated on:21/07/2023

Class 10 MATHS COORDINATE GEOMETRY

Topper's Solved these Questions

Similar Questions

NAGEEN PRAKASHAN ENGLISH-CO-ORDINATE GEOMETRY-Miscellaneous Examples

Home
Ask Doubt
Profile