Find the coordinates of the points which divide the line segment joining A(−2,2)andB(2,8) into four equal parts.
Let P, Q and R be the poins on line segment AB such that
AP=PQ=QR=RB
Let AP=PQ=QR=RB=k
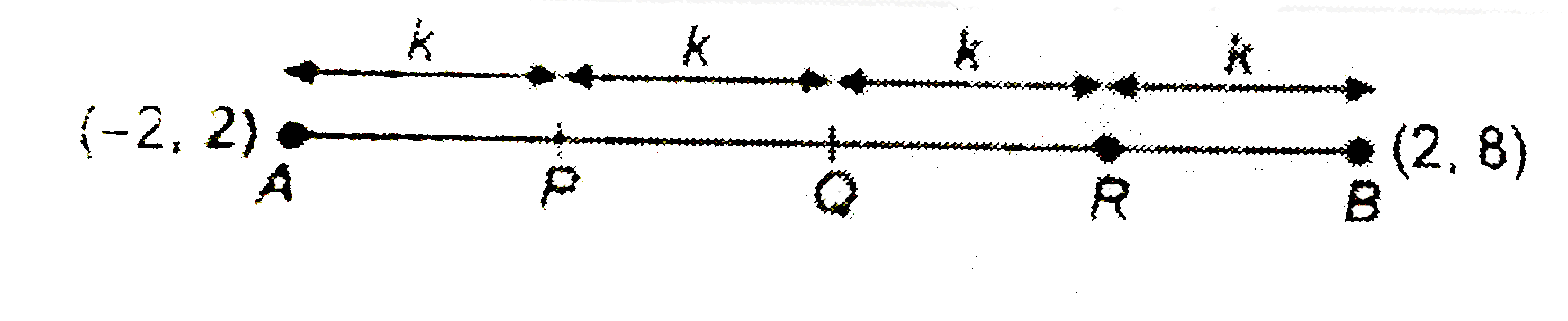
Now, APPB=k3k=13
Therefore, P divides AB internally in the ration 1 : 3.
∵ Internally ratio=(m1x2+m2x1m1+m2,m1y2+m2y1m1+m2)
P=(1×2+3(−2)1+3,1×8+3×21+3)=(2−64,8+64)=(−44,144)=(−1,72)
Again, ARRB=3kk=31
Therefore, R divides AB internally in the ratio 3 : 1.
∴ R=(3×2+1×(−2)3+1,3×8+1×23+1)
=(6−24,24+24)=(44,264)=(1,132)
Also, AQQB=2k2k=11
∴ Q is the mid-point of AB.
∴ Q=(−2+22,2+82)[∵mid-point=(x1+x22,y1+y22)]
i.e., Q=(02,102)=(0,5)
So, required points are (−1,72),(0,5)and(1,132).
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Exercise 7a21videos
CO-ORDINATE GEOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Exercise 7b18videos
CO-ORDINATE GEOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Miscellaneous Examples8videos
CO-ORDINATE GEOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CIRCLESEXERCISE - Long Answer Questions2videos
CIRCLES
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Exercise 11 B10videos
CONSTRUCTIONS
Similar Questions
Find the points which divide the line segment joining (8,12) and (12,8 ) into four equal parts.
View SolutionFind the coordinates of the point which trisect the line segment joining A(2,1,−3) and B(5,−8,3).
View SolutionFind the coordinates of the points which divide the line segment joining the points (−4, 0) and (0, 6) in four equal parts.
View SolutionFind the coordinates of the points which trisect the line segment joining (0, -3, 2) and (1, 3, 3).
View SolutionFind the coordinates of the points which trisect the line segment joining (2, 1, 0) and (5, 8, -3).
View SolutionFind the coordinates of the point which divides the line segment joining (2,4) and (6,8) in the ratio1:3 internally and externally.
View SolutionFind the coordinates of points which trisect the line segment joining (1,−2)and(−3,4).
View SolutionFind the coordinates of the points of the line segment joining (1, 3, 5) and (-2, 1, 2) in four
equal parts.
[Hint : Ratio may be 3 : 1, 1 : 1 or 1 : 3.]View SolutionFind the coordinates of the point which divides the line segment joining (−1, 3) and (4, −7) internally in the ratio 3:4 .
View SolutionFind the coordinates of the point which divides the line segment joining the point, (−2,3,5) and (1,−4,6) in the ratio.
2 : 3 internally,View Solution

