The angle of depression between a flying kite and 'a' point on the ground is 60 m. If the string makes 30∘.Find the height of the kite from the ground.
The correct Answer is:30 m
To solve the problem, we need to find the height of the kite from the ground using the given information. Here’s the step-by-step solution:
Step 1: Understand the Problem
We have a kite flying at a height above the ground. The angle of depression from the kite to a point on the ground is given, and the length of the string (which is the hypotenuse of the triangle formed) is also provided.
Step 2: Draw a Diagram
Let’s denote:
- Point A: The point on the ground directly below the kite.
- Point B: The position of the kite.
- Point C: The point on the ground where the angle of depression is measured.
In triangle ABC:
- AB is the length of the string (hypotenuse) = 60 m.
- Angle BAC = 30° (the angle the string makes with the horizontal).
- BC is the height of the kite from the ground, which we need to find.
Step 3: Use Trigonometric Ratios
In triangle ABC, we can use the sine function, which relates the angle to the opposite side (height of the kite) and the hypotenuse (length of the string).
The sine function is defined as:
sin(θ)=OppositeHypotenuse
For our triangle:
sin(30°)=BCAB
Step 4: Substitute the Known Values
We know:
- sin(30°)=12
- AB=60m
Substituting these values into the equation gives:
12=BC60
Step 5: Solve for BC
To find BC (the height of the kite), we can rearrange the equation:
BC=60×12
BC=30m
Final Answer
The height of the kite from the ground is 30 meters.
---
We have a kite flying at a height above the ground. The angle of depression from the kite to a point on the ground is given, and the length of the string (which is the hypotenuse of the triangle formed) is also provided.
Let’s denote:
- Point A: The point on the ground directly below the kite.
- Point B: The position of the kite.
- Point C: The point on the ground where the angle of depression is measured.
- AB is the length of the string (hypotenuse) = 60 m.
- Angle BAC = 30° (the angle the string makes with the horizontal).
- BC is the height of the kite from the ground, which we need to find.
In triangle ABC, we can use the sine function, which relates the angle to the opposite side (height of the kite) and the hypotenuse (length of the string).
sin(θ)=OppositeHypotenuse
sin(30°)=BCAB
We know:
- sin(30°)=12
- AB=60m
12=BC60
To find BC (the height of the kite), we can rearrange the equation:
BC=60×12
BC=30m
The height of the kite from the ground is 30 meters.
Topper's Solved these Questions
- SOME APPLICATIONS OF TRIGONOMETRYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Revision Exercise (short Answer Questions)
5 Videos
- SOME APPLICATIONS OF TRIGONOMETRYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Revision Exercise (long Answer Questions)
1 Videos
- SOME APPLICATIONS OF TRIGONOMETRYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - Long Answer Questions
5 Videos
- REAL NUMBERSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - REAL NUMBERSEXERCISE - Revision Exercise Long Answer Questions
5 Videos
- STATISTICS BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions
4 Videos
Similar Questions
The length of a string between a kite and a point on the ground is 90 metres. If the string makes an angle θ with the ground level such that tanθ=158, how high is the kite? Assume that there is no slack in the string.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower is 30∘ . Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View SolutionAn aeroplane flying at a height 300 metre above the ground passes vertically above another plane at an instant when the angles of elevation of the two planes from the same point on the ground are 60∘ and 45∘ respectively. Then the height of the lower plane from the ground in metres is
View Solution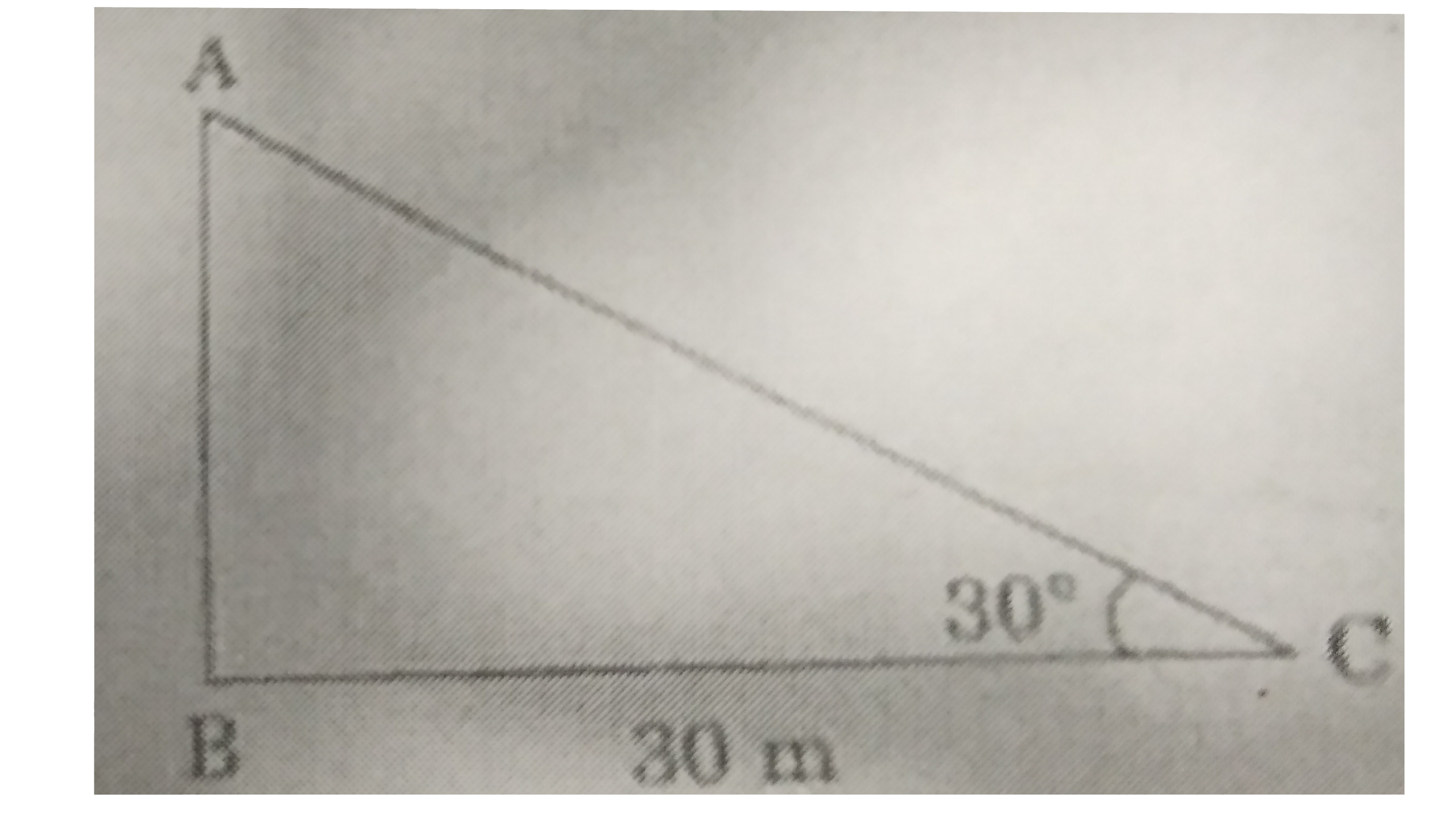
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View SolutionIn the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
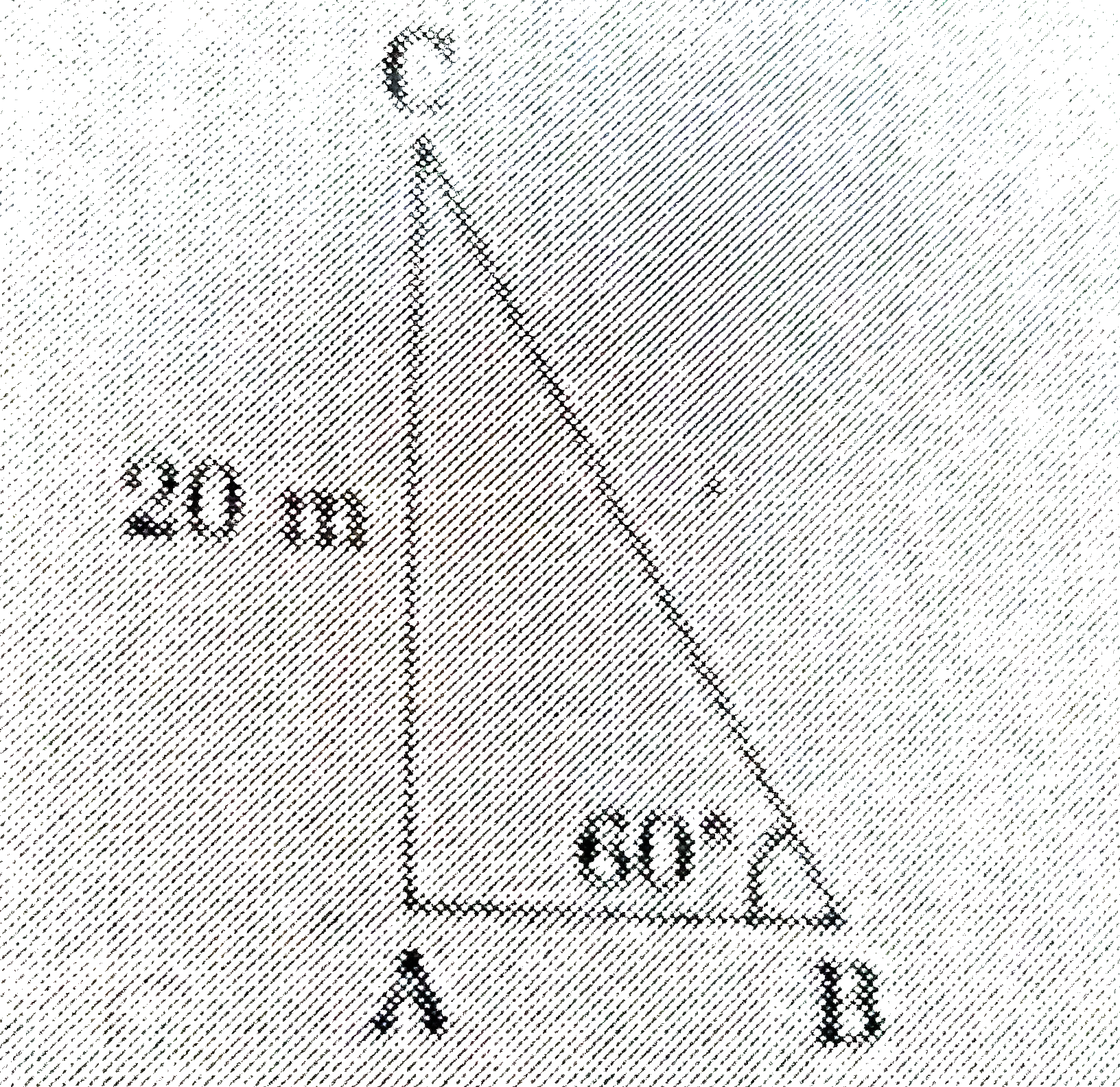
View Solution
