Question

Factorise : $x^5+y^5$

Explanation

Let `p(x,y)=x^(5)+y^(5)`
Put =-y
`therefore p(-y,y)=(-y)^(5)+y^(5)=-y^(5)+y^(5)=0`
Since remainder =0
`therefore (x+y)` is factor of p(x,y).
`therefore p(x,y)=x^(5)+y^(5)=x^(4)(x+y)-x^(4)y+y^(5)=x^(4)(x+y)-x^(3)y(x+y)+x^(3)y^(2)(x+y)+x^(3)y^(2)+y^(4)`
`=x^(4)(x+y)-x^(3)y(x+y)+x^(2)y^(2)(x+y)-x^(2)y^(3)+y^(5)`
`=x^(4)(x+y)-x^(3)y(x+y)+x^(2)y^(2)(x+y)-xy^(3)(x+y)+xy^(4)+y^(5)`
`=x^(4)(x+y)-x^(3)y(x+y)+x^(2)y^(2)(x+y)-xy^(3)(x+y)+y^(4)(x+y)-y^(5)+y^(5)`
`=(x+y)*x^(4)-x^(3)y+x^(2)y^(2)-xy^(4)+y^(4))`
Instead of the above method, we can do the above factors by actual division as :
`x^(4)-x^(3)y+x^(2)y^(2)-xy^(3)+y^(4)`

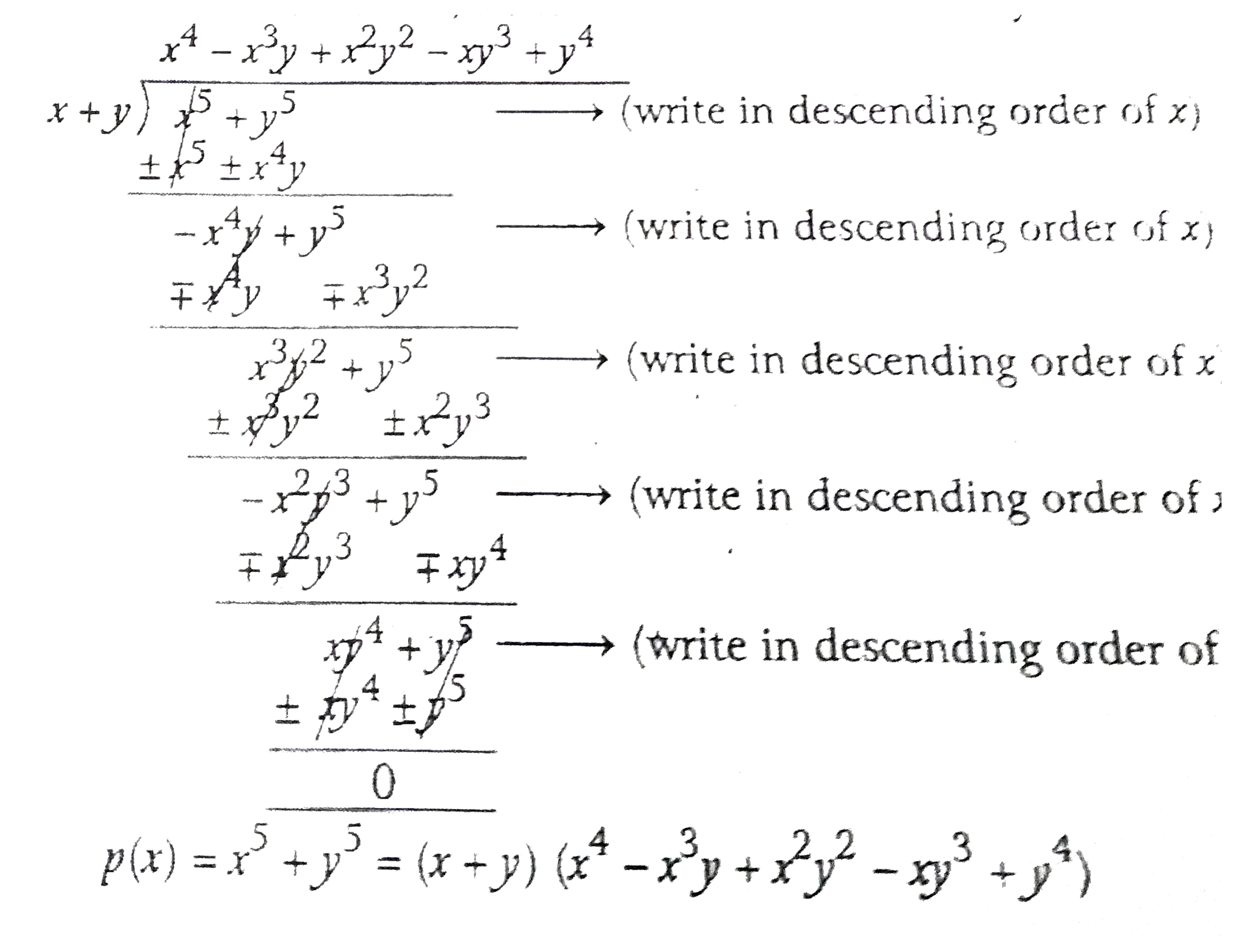
`therefore p(x)=x^(5)+y^(5)=(x+y)(x^(4)-x^(3)y+x^(2)y^(2)-xy^(3)+y^(4))`