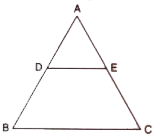
In the adjoining figure BC||DE. Area of △=25cm2 .area of trapezium BCED=24cm2 , DE= 14 cm, calculate length of BC.
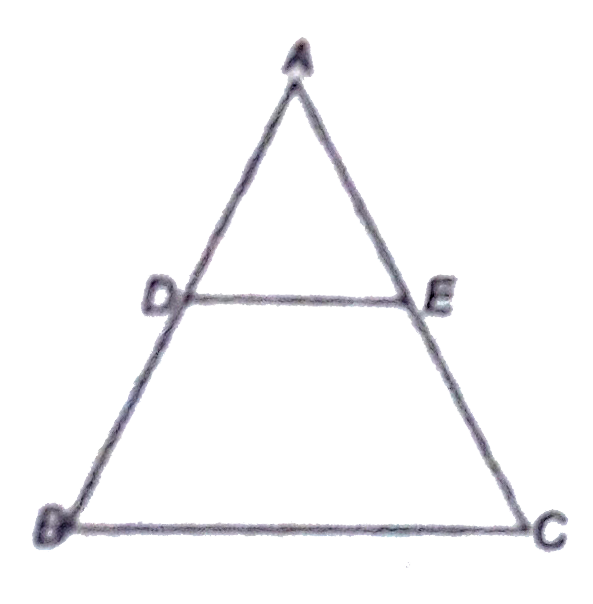
The correct Answer is:70cm
Step by step video & image solution for In the adjoining figure BC||DE. Area of triangle= 25 cm^(2) .area of trapezium BCED= 24cm^(2) , DE= 14 cm, calculate length of BC. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLES Book:NAGEEN PRAKASHAN ENGLISHChapter:TRIANGLES Exercise:Exercise 6d
Explore 27 Videos
- TRIANGLES Book:NAGEEN PRAKASHAN ENGLISHChapter:TRIANGLES Exercise:Revision Exercise very shot Questions
Explore 1 Video
- TRIANGLES Book:NAGEEN PRAKASHAN ENGLISHChapter:TRIANGLES Exercise:Exercise 6b
Explore 29 Videos
- STATISTICS Book:NAGEEN PRAKASHAN ENGLISHChapter:STATISTICS Exercise:Revision Exercise Long Answer Questions
Explore 4 Videos
- VOLUME AND SURFACE AREA OF SOLIDSBook:NAGEEN PRAKASHAN ENGLISHChapter:VOLUME AND SURFACE AREA OF SOLIDSExercise:Revisions Exercise Long Answer Questions
Explore 5 Videos
Similar Questions
In the given figure, BC is parallel to DE. Area of triangle ABC=25cm2, Area of trapezium BCED=24cm2andDE=14cm. Calculate the length of BC.
Also, find the area of triangle BCD.
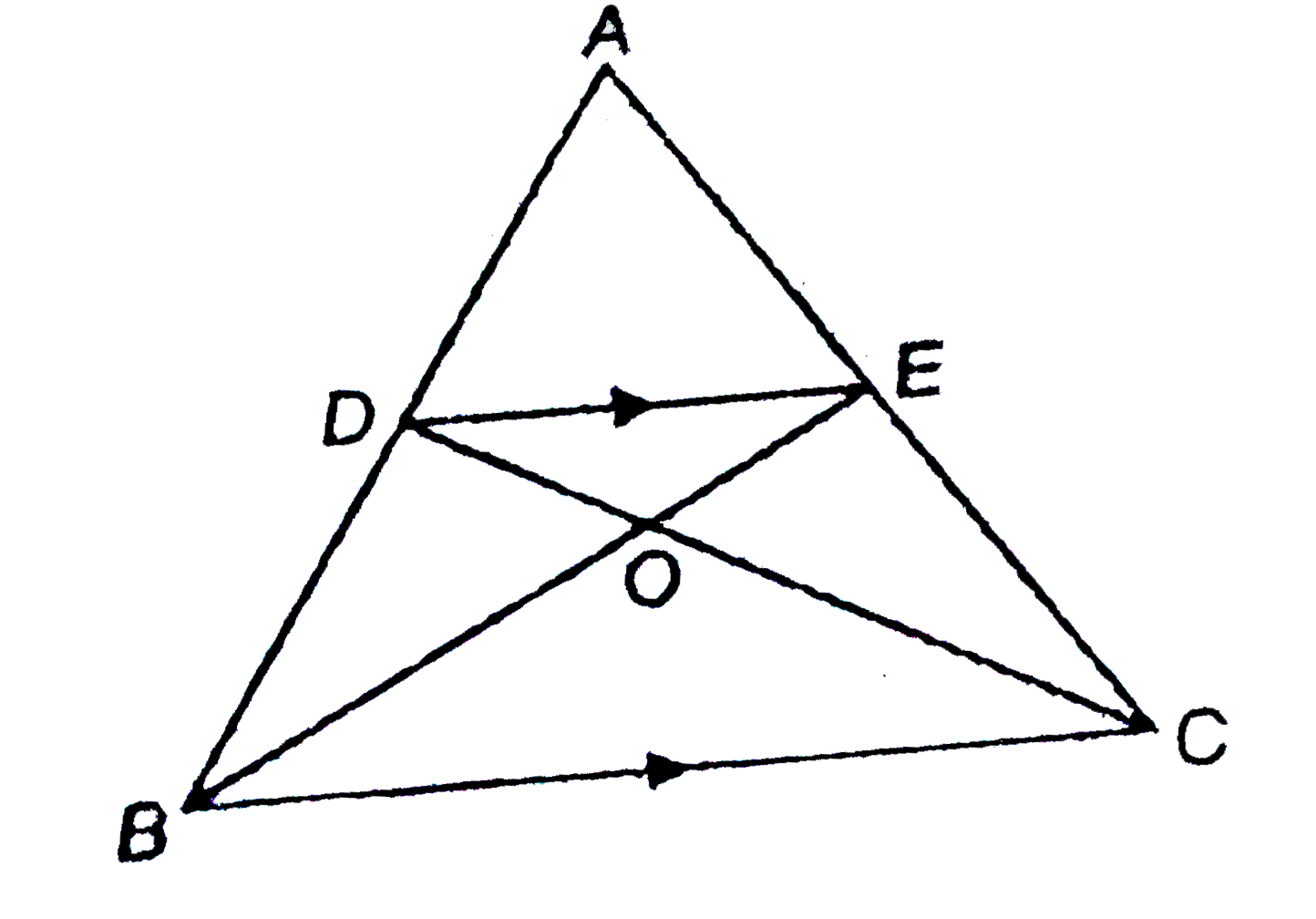
View SolutionIn the adjoining figure, DE∣∣BC. Prove that area (ΔACD)= area (ΔABE)
View SolutionIn triangle ABC, given below, AB = 8 cm, BC = 6 cm and AC = 3 cm. Calculate the length of OC

View SolutionThe area of a triangle with base 4 cm and height 6 cm is 24 cm2.
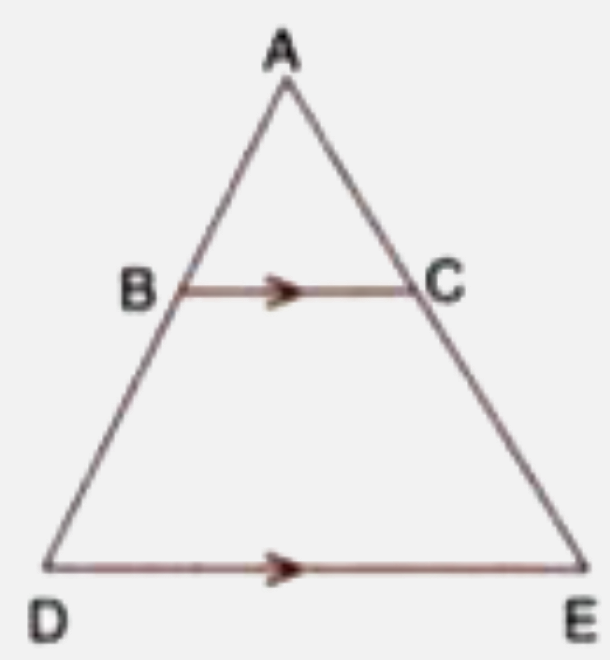
View SolutionIn the adjoining figure, DE//BC and D divides AB in the ratio 2 : 3. Find DE, if BC = 7.5 cm.
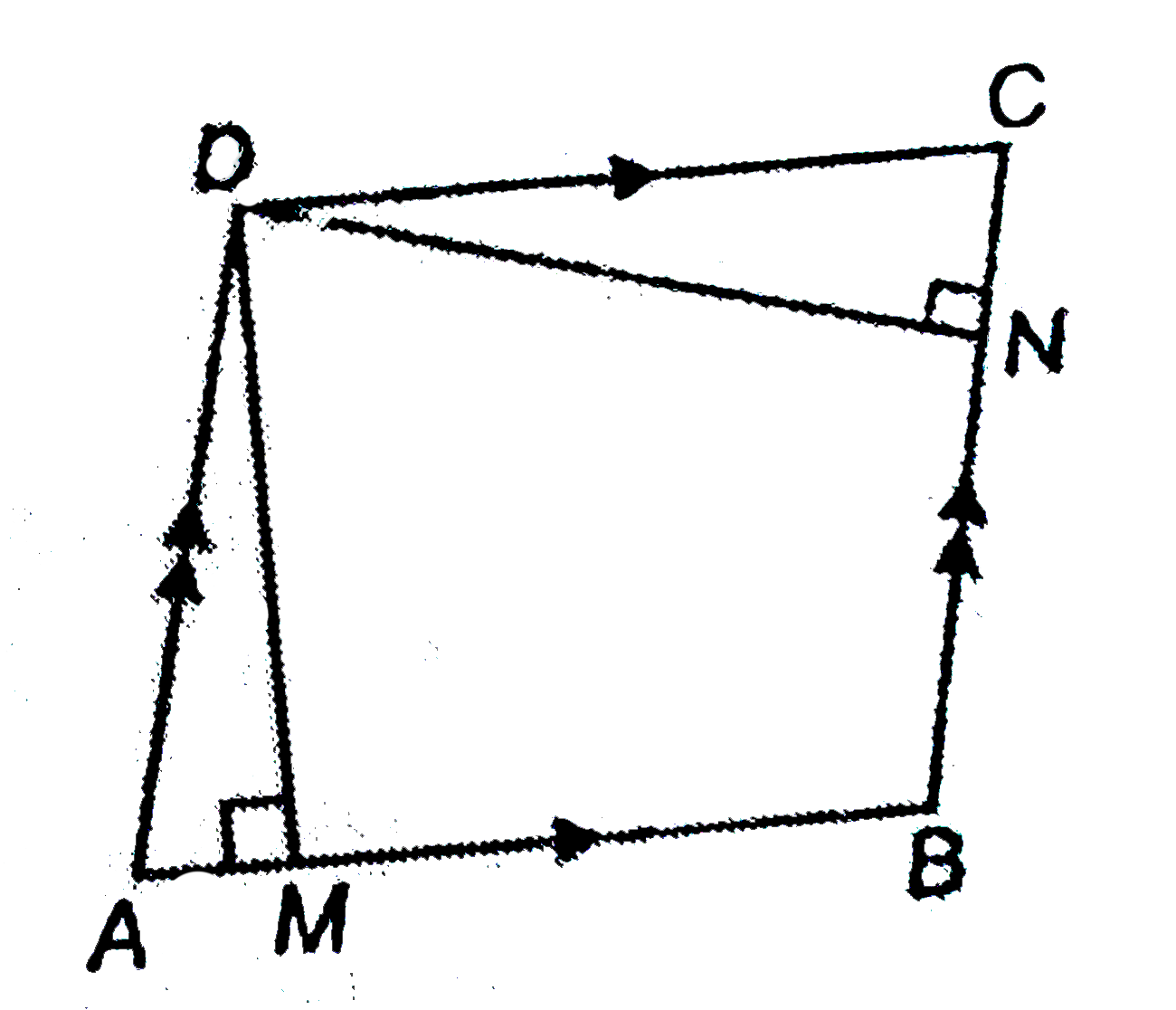
View SolutionIn the adjoining figure, AB=8cm,DM=6cmandBC=6cm. Find the length of DN
View SolutionIn the adjoining figure, ΔABC is right angled at C and M is the mid-point of hypotenuse AB, If AC = 32 cm and BC = 60 cm, then find the length of CM.

View SolutionIn the adjoining figure, DE//BC and D divides AB in the ratio 2 : 3. Find :
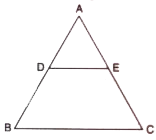
AEEC
View SolutionIn the adjoining figure, DE//BC and D divides AB in the ratio 2 : 3. Find :
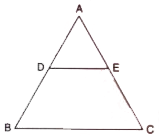
AEAC
View SolutionIn the given figure, AB and DE are perpendiculars to BC. If AB = 9 cm, DE = 3 cm and AC = 24 cm, calculate AD.

View Solution