A person walking at 4 m/s finds rain drops falling slantwise in to his face with a speed of 4m/s at an angle of $30^{\circ}$ with the vertical. Show that the actual speed of the rain drops is 4m/s.

Text Solution

Verified by Experts

Velocity of the person $(V_{p}) = 4 m / s$
$v = 4 m / s, θ = 30^{\circ}$
Relative velocity $= v_{r} - v_{p}$
$v = \sqrt{v_{r}^{2} + v_{p}^{2} - 2 v_{r} v_{p} \cos θ}$
$4 = \sqrt{v_{r}^{2} + 4^{2} - 2 \times v_{r} \times 4 \times {\cos 60}^{\circ}}$
$4^{2} = v_{r}^{2} + 4^{2} - 2 \times v_{r} \times 4 \times \frac{1}{2}$

$v_{r}^{2} - 4 v_{r} = 0$
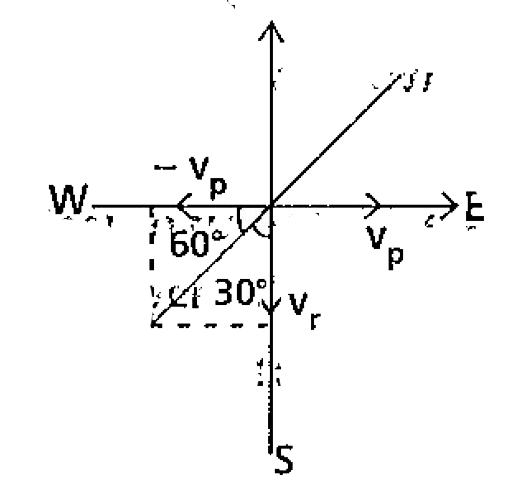
Velocity of rain drop $(v_{r}) = 4 m / s$

|

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Similar Questions

Knowledge Check

Question 1 - Select One
A man walking with 3 m/s feels the rain falling vertically with a speed of 4 m/s. Find the angle which the rainfall makes with the vertical according to a stationary observer.
A $37^{\circ}$
B $53^{\circ}$
C ${tan}^{- 1} (\frac{3}{5})$
D ${tan}^{- 1} (\frac{5}{3})$
Question 2 - Select One
A man running on the horizontal road at $8 k m h^{- 1}$ find the rain appears to be falling vertically. He incresases his speed to $12 k m h^{- 1}$ and find that the drops make angle $30^{2}$ with the vertical. Fin dthe speed and direction of the rain with respedt to the road.
A $4 \sqrt{7}$ km/h
B $9 \sqrt{7}$ km/h
C $12 \sqrt{7}$ km/h
D $15 \sqrt{7}$ km/h
Question 3 - Select One
A man running with velocity $3 m / s$ finds that raindrops are hitting him vertically with a speed of $4 m / s$ .
Raindrops are falling at an angle $θ$ with the vertical. Value of $θ$ is
A $37^{\circ}$
B $53^{\circ}$
C $45^{\circ}$
D $60^{\circ}$

Recommended Questions

Home
Ask Doubt
Profile