A 20μF capacitor is charged to a potential difference of 1000 V. The terminals of this charged capacitor are then connected to those of an uncharged 5μF capacitor. Calculate the final potential difference across each capacitor.
Given, C1=20μF=20×10−6F,V1=1000V,
C2=5μF=5×10−6F,V2=0
After joining a common potential is developed
i.e. C=C1V1+C2V2C1+C2
=20×10−6×1000+5×10−6×020×10−6+5×10−6
=20×10−6×10325×10−6=200×10225=800V
∴ Final potential difference across each capacitor is 800 V.
Similar Questions
Knowledge Check
A 10μF capacitor is charged to a potential difference of 1000V. The terminals of the charged capacitor are disconnected from the power supply and connected to the terminals of an uncharged 6μF capacitor. What is the final potential difference across each capacitor ?
A167VB100VC625VD250VA capacitor or capacitance C1 is charge to a potential V and then connected in parallel to an uncharged capacitor of capacitance C2. The fianl potential difference across each capacitor will be
AC1VC1+C2BC2VC1+C2C1+C2C1D1−C2C1
Five capacitors are connected across a battery of 5V as shown in figure (o). Calculate the potential difference across the plates of the 5μF capacitor.
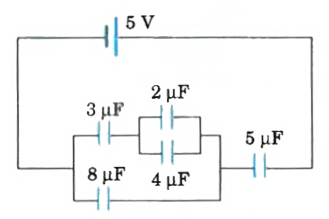
View SolutionA 10μF capacitor is charged to a potential difference of 50V and is connected to another uncharged capacitor in parallel. Now the common potential difference becomes 20 volt. The capacitance of second capacitor is
View SolutionA μF capacitor C1 is charged to V0=120V. The charging battery is then removed and the capacitor is connected in parallel to an uncharged +4μF capacitor C2.
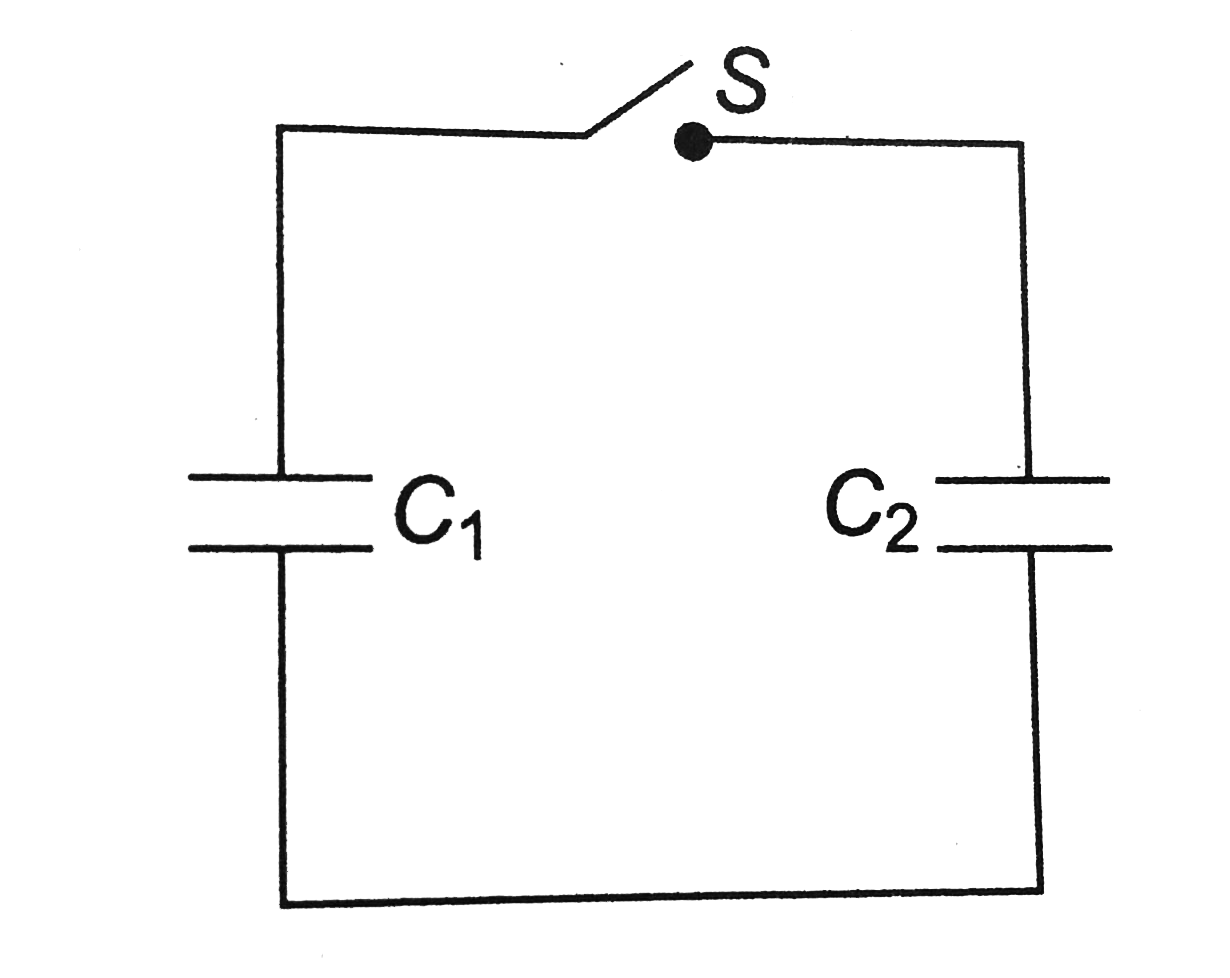
(a) what is the potential difference V across the combination?
(b) what is the stored energy before and after the switch S is closed?
View SolutionA capacitor of capacitance 5 μF is charged to a potential difference of 10 volts and another capacitor of capacitance 9 μF is charged to a potential difference of 8 volts. Now these two capacitors are connected in such a manner that the positive plate of one is connected to the positive plate of other. Calculate the common potential difference across the two capacitors.
View SolutionA 10μF capacitor is charged to a potential difference of 50V and is connected to another uncharged capacitor in parallel. Now the common potential difference becomes 20 volt. The capacitance of second capacitor is
View SolutionA 10μF capacitor is charged to a potential difference of 50V and is connected to another uncharged capacitor in parallel. Now the common potential difference becomes 20 volt. The capacitance of second capacitor is
View Solution

