Why do we use resistor in electrical circuit?
Derive expression for equivalent resistance when three resistors are arranged in
(i) series combination,
(ii) parallel combination.
Text Solution
Resistors are used in electrical and electronic circuits for the controlled flow of current.
(i) Resistors in series
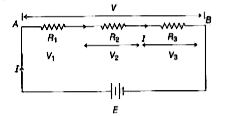
Consider three resistors having resistances R1,R2andR3 are connected in series. Let V be the potential difference applied across A and B using the battery and the same current I be passing through each resistance. If V1,V2andV3 are the potential difference across R1,R2andR3 respectively, then
V1=IR1,V2=IR2
and V3=IR3 (Ohm.s law)
But, V=V1+V2+V3=IR1+IR2+IR3
V=I(R1+R2+R3)
If Rs is the equivalent resistance of the given series combination of resistances.
Then, V=IRs
∴Rs=VI=II(R1+R2+R3)
⇒R3=R1+R2+R3
Thus, the equivalent resistance of a number of resistors connected in series is equal to the sum of individual resistances.
(ii) N/A
Thus, the reciprocal of equivalent resistance of a number of resistors connected in parallel is equal to the sum of the reciprocals of the individual resistances.
|
Similar Questions
Knowledge Check
Equivalent resistance R, of the parallel combination when two resistors Rand R, are connected in parallel is equal to
ARp=R1+R2R1R2BRp=R1R2R1−R2CRp=R1−R2R1+R2DRp=R1+R2R1+R2Consider the combination of resistor,

The equivalent resistance between a and b isAR6B2R3CR3D3RThere are mainly two types of combinations of resistors in a circuit, series and parallel. In the given table, Column I shows voltages of different arrangements of resistors, Column II shows different arrangement of resistors and Column III shows currents of different arrangements of resistors.
What are the conditions when resistors are in mixed combination, that is series and parallel combination respectively?
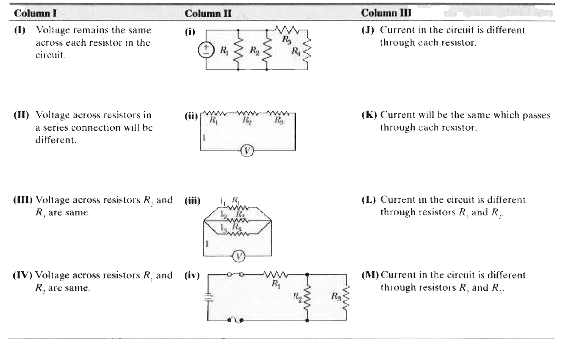 A(III) (i) (L)B(I) (i) (J)C(IV) (i) (L)D(II) (iii) (M)
A(III) (i) (L)B(I) (i) (J)C(IV) (i) (L)D(II) (iii) (M)
Combination of Resistors|Series Combination|Parallel Combination
View SolutionWhy is resistance more in series combination of resistors ?
View SolutionDerive the expression for equivalent spring constant of
(i) parallel combination of n−springs
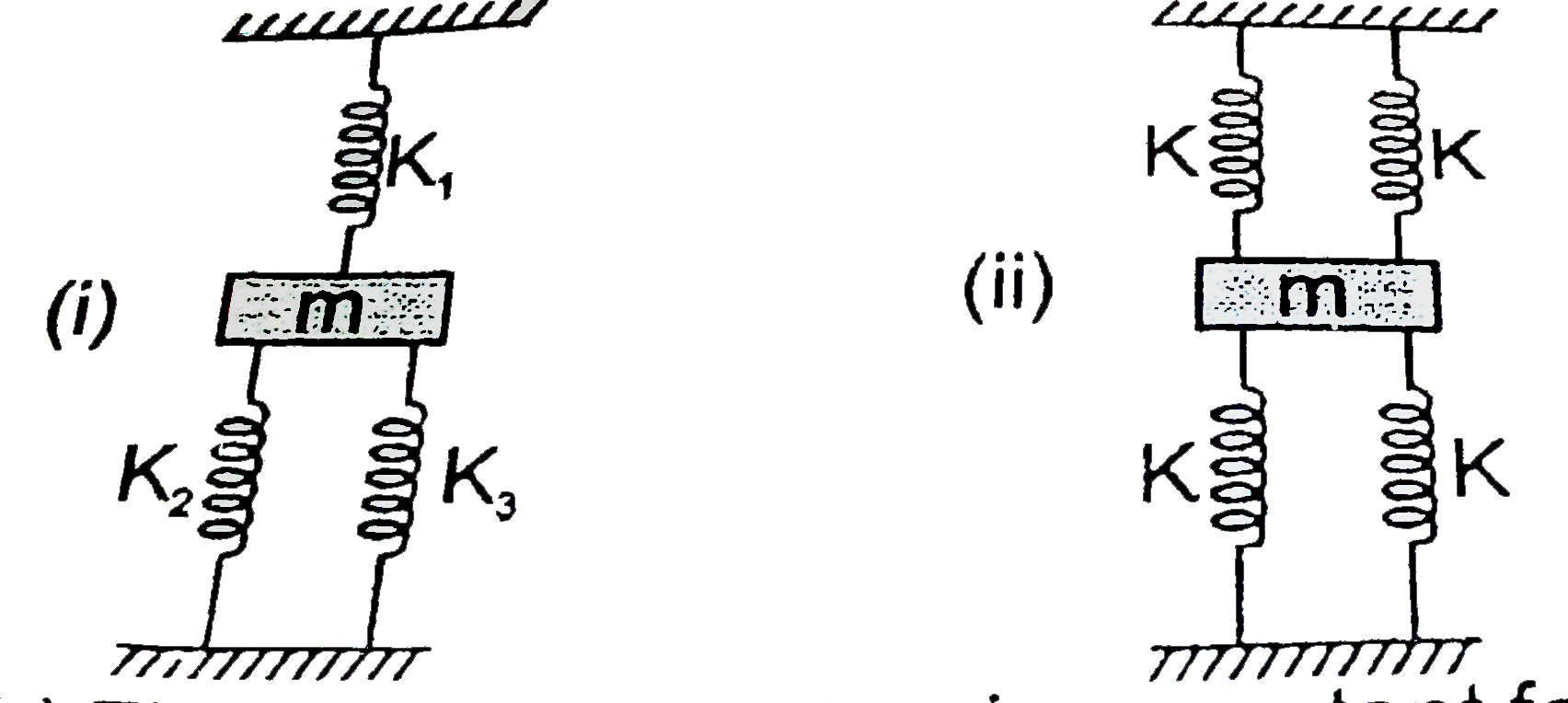
(ii) series combination of n−springs
View SolutionWhat is Ohm's law ? How is it represented graphically ? Derive an expression for the resultant resistance of a series combination of resistors.
View SolutionConsider the combination of resistor,

The equivalent resistance between a and b is
View Solution
