An AC circuit contains a resistance in series. Draw the circuit. What is the phase of the current in the inductance with respect to applied emf ?

Text Solution

Verified by Experts

Circuit diagram

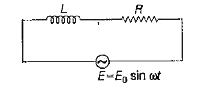
Phasor diagram of the circuit is

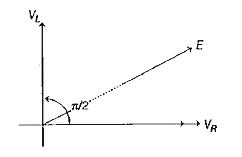
Applied emf is phasor (vector) sum of $V_{L} and V_{R}$ .
$∴ E = \sqrt{V_{L}^{2} + V_{R}^{2}}$
= $= \sqrt{I^{2} X_{L}^{2} + I^{2} R^{2}}$
$= I \sqrt{X_{L}^{2} + R^{2}} = 1 Z$
where, $Z = \sqrt{X_{L}^{2} + R^{2}}$
$∴$ Circuit current, $I = \frac{E}{\sqrt{X_{L}^{2} + R^{2}}}$
where $X_{L} = L ω$ .
From phasor diagram it is clear that voltage and current of inductor has a phase different of $\frac{π}{2}$ with leading voltage.

Updated on:21/07/2023

In a simple A.C. circuit containing resistance, the current
Alags behind the e.m.f. by $π / 2$
Bis in phase with applied e.m.f.
Cleads the applied e.m.f. by $π / 2$
Dnone of these
An a.c. circuit contains a resistance of $3 Ω$ and inductive reactance of $4 Ω$ . The cosine of the phase angle between the current and potential difference in this circuit is
A $\frac{2}{5}$
B $\frac{3}{5}$
C $\frac{3}{2}$
D $\frac{4}{5}$
In an AC circuit, a resistance R is connected in series with an inductance L. If the phase angle between the voltage and the current is pi//4 then the value of ihe inductive reactance is
A $R / 4$
B $R / 2$
CR
D $R / s$