An AC source of emf $ε = ε_{0} sin ω t$ is connected in series with a pure resistance R only. Derive an expression for the instantaneous power of this circuit ans show that it is pulsating in nature.

Text Solution

Verified by Experts

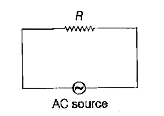
In the above figure, a resistor of resistance R is connected in series to an AC source of emf with instantaneous value $e = E_{0} sin ω t$ .
Suppose, E is the potential drop across resistance R, then
E = IR
emf = instantaneous value of potential drop.
$∴$ Eqs. (i) and (ii), we have
IR = E
$\Rightarrow I = \frac{E}{R} = \frac{E_{0} sin ω t}{R}$
rArr $I = I_{0} sin ω t$
$∴$ Instantaneous power is given by
$P_{\in s t} = E . I$
$\Rightarrow P_{\in s t} = E_{0} sin ω t . I_{0} sin ω t$
$\Rightarrow P_{\in s t} = E_{0} I_{0} {sin}^{2} ω t$
As it can be seen in Eq. (iv) that instantaneous power expression includes ${sin}^{2} ω t$ (an ever function) which itself shows, that, instantaneous power is pulsating in nature.

An AC source producing emf $V = V_{0}$ "["sin omega t+sin 2omegat"]" is connected in series with a capacitor and a resistor. The current found in the circuit is

A $i_{1} - i_{2}$
B $i_{1} < i_{2}$
C $i_{1} > i_{2}$
D $i_{1}$ may be less than, equal to or greater than $i_{2}$
A series combination of $R, L C$ is connected to an a.c source. If the resistance is $3 Ω$ and the reactance is $4 Ω$ , the power factor of the circuit is
A0.4
B0.6
C0.8
D $1.0$
An element with $e m f ε$ and interval resistance $r$ is connected across an external resistance $R$ . The maximum power in external circuit is $9 W$ . The current flowing through the circuit in these conditions is $3 A$ . Then which of the following is // are correct ?
A $ε = 6 V$
B $r = R$
C $r = 1 Ω$
D $r = 3 Ω$