Given , SI unit and SI unit, calculate the speed of electromagnetic waves in air
Given , SI unit
and SI unit
Using formula,
or
or
Similar Questions
The permittivity and permeability of free space are ε0=8.85×10−12C2N−1m−2andμ0=4π×10−7TmA−1, respectively. Find the velocity of the electromagnetic wave.
View SolutionThe permittivity and permeability of free space are ε0=8.85×10−12C2N−1m−2andμ0=4π×10−7TmA−1, respectively. Find the velocity of the electromagnetic wave.
View Solution
Knowledge Check
The electric field portion of an electromagnetic wave is given by ( all variables in SI units) E=10−4sin(6×105t−0.01x) The. Frequency (f) and the speed (v) of electromagnetic wave are
Af=30π kHz and v=1.5×107ms−1Bf=90π kHz and v=6.0×107ms−1Cf=300π kHz and v=6.0×107ms−1Df=600π kHz and v=7.5×107ms−1The electric field portion of an electromagnetic wave is given by ( all variables in SI units) E=10−4sin(6×105t−0.01x) The. Frequency (f) and the speed (v) of electromagnetic wave are
Af=30πkHzandv=1.5×107ms−1Bf=90πkHzandv=6.0×107ms−1Cf=300πkHzandv=6.0×107ms−1Df=600πkHzandv=7.5×107ms−1The graph given below shows the variation of electric field E (in MV/m) with time t (in μs) in a parallel plate capacitor
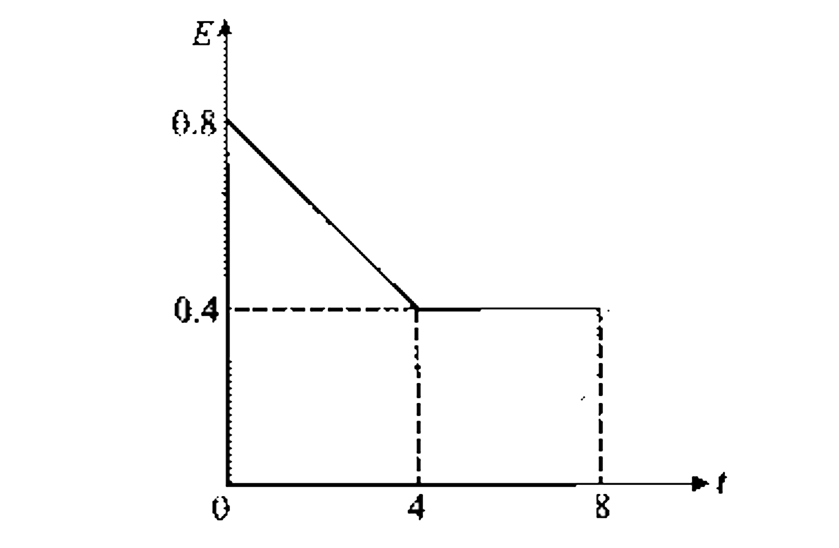
Consider the statements:
(1) The displacement current through a 1m2 region perpendicular to the during the time interval t=0 to t=4μs is 0.0885A (given ε0=8.85×10−12 SI unit)
(2) The displacement current through 1m2 region perpendicular to the field during the time interval t=4μs to 8μs is zero.
Which of the statements given above is/are correct:A1 onlyB2 onlyCboth 1 and 2Dneither 1 nor 2
The electric field portion of an electromagnetic wave is given by ( all variables in SI units) E=10−4sin(6×105t−0.01x) The. Frequency (f) and the speed (v) of electromagnetic wave are
View SolutionThe magnetic field in a plane electromagnetic wave is given by: By=12×10−8sin(1.20×107z+3.60×1015t)T. Calculate the
Energy density associated with the Electromagnetic wave.
View SolutionThe magnetic field in a plane electromagnetic wave is given by: By=12×10−8sin(1.20×107z+3.60×1015t)T. Calculate the
Speed. of the wave
View SolutionThe magnetic field of an electromagnetic wave is given by
3×10−7sin(103x+6.28×1012t). The wave length of the electromagnetic wave is
View SolutionThe magnetic field in a plane electromagnetic wave is given by
By=2×10−7sin(0.5×103x+1.5×1011t).
This electromagnetic wave is
View Solution
