A simple pendulum has time period T1 The point of suspension is now moved upward according to the relation y=kt2(k=1m/s2) where y is vertical displacement, the time period now becomes T2. The ratio of (T1T2)2 is : (g=10m/s2)
45
65
560
1
The correct Answer is:B
To solve the problem, we need to find the ratio of the squares of the time periods T1 and T2 of a simple pendulum when the point of suspension is moved upward with a specific acceleration. Let's break down the solution step by step.
Step 1: Understand the Time Period of a Simple Pendulum
The time period of a simple pendulum is given by the formula:
T=2π√Lg
where L is the length of the pendulum and g is the acceleration due to gravity.
Step 2: Determine the Acceleration
The point of suspension is moved upward according to the relation:
y=kt2
where k=1m/s2. The acceleration a is the second derivative of displacement with respect to time:
a=d2ydt2=d2(kt2)dt2=2k=2×1=2m/s2
Step 3: Modify the Time Period Formula for T2
When the point of suspension is moving upward with an acceleration a, the effective acceleration acting on the pendulum becomes g−a. Therefore, the new time period T2 is given by:
T2=2π√Lg−a
Step 4: Write the Time Periods in Terms of L and g
Now we have:
T1=2π√Lg
T2=2π√Lg−2
Step 5: Find the Ratio of the Time Periods
Now we need to find the ratio (T1T2)2:
T1T2=2π√Lg2π√Lg−2=√Lg√Lg−2
This simplifies to:
T1T2=√g−2g
Squaring both sides gives:
(T1T2)2=g−2g
Step 6: Substitute the Value of g
Given g=10m/s2:
(T1T2)2=10−210=810=45
Final Answer
Thus, the ratio (T1T2)2 is:
45
The time period of a simple pendulum is given by the formula:
T=2π√Lg
where L is the length of the pendulum and g is the acceleration due to gravity.
The point of suspension is moved upward according to the relation:
y=kt2
where k=1m/s2. The acceleration a is the second derivative of displacement with respect to time:
a=d2ydt2=d2(kt2)dt2=2k=2×1=2m/s2
When the point of suspension is moving upward with an acceleration a, the effective acceleration acting on the pendulum becomes g−a. Therefore, the new time period T2 is given by:
T2=2π√Lg−a
Now we have:
T1=2π√Lg
T2=2π√Lg−2
Now we need to find the ratio (T1T2)2:
T1T2=2π√Lg2π√Lg−2=√Lg√Lg−2
This simplifies to:
T1T2=√g−2g
Squaring both sides gives:
(T1T2)2=g−2g
Given g=10m/s2:
(T1T2)2=10−210=810=45
Thus, the ratio (T1T2)2 is:
45
Similar Questions
A simple pendulum has time period T_1. The point of suspension is now moved upward according to the relatiori. y = kt2 (k = 2 m/s2) where y is the vertical displacement. The time period now becomes T2 then the ratio of T21/T22 (g = 10 m/s2)
View SolutionA simple pendulum has time period 2s. The point of suspension is now moved upward accoding to relation y=(6t−3.75t2)m where t is in second and y is the vertical displacement in upward direction. The new time period of simple pendulum will be
View Solution
Knowledge Check
A simple pendulum has time period (T_1). The point of suspension is now moved upward according to the relation y=Kt2,(K=1m/s2) where (y) is the vertical displacement. The time period now becomes (T_2). The ratio of T21T22 is (g=10m/s2).
A6/5B5/6C1D4/5
In the arrangement as shown, tension T2 is (g=10m/s2)
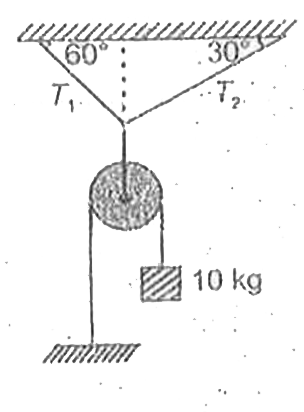
View SolutionA bob of a simple pendulum of mass 40 mg with a positive charge 4×10−6C is oscilliating with time period T1. An electric field of intensity 3.6×104 N/c is applied vertically upwards now time period is T2. The value of T2T1 is (g=10ms2)
View SolutionA simple pendulum has a time period T1 when on the earth's surface and T2 when taken to a height R above the earth's surface, where R is the radius of the earth. The value of T2T1 is
View SolutionA pendulum has time period T in air when it is made to oscillate in water it acquired a time period T=√2T The density of the pendulum bob is equal to (density) of water =1)
View SolutionA pendulum has time period T in air when it is made to oscillate in water it acquired a time period T=√2T The density of the pendulum bob is equal to (density) of water =1)
View SolutionA simple pendulum has time period T = 2s in air. If the whole arrangement is placed in a non viscous liquid whose density is 1/2 times the density of bob. The time period in the liquid will be
View SolutionWhen the string of a conical pendulum makes an angle of 45∘ with the vertical , its time is T1 when the string makes an angle of 60∘ with the vertical , its time period is T2 then T21/T22is
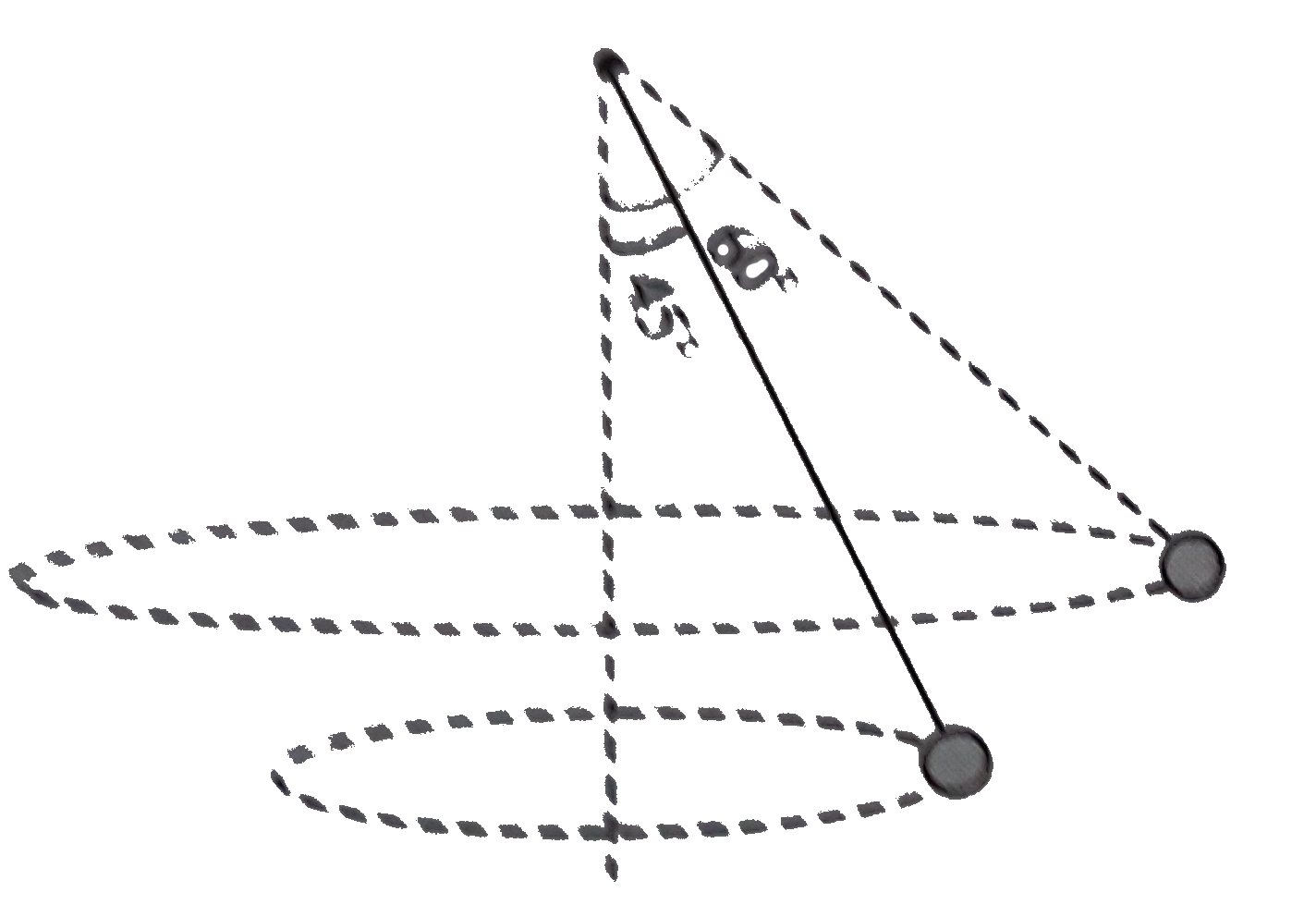
View Solution
