A steel ball strikes a fixed smooth steel plate placed on a horizontal surface atan angle θ with the vertical. If the coefficient of restitution is e, the angle at which the rebound will take place is:
θ
tan−1[tanθe]
etanθ
tan−1[etanθ]
The correct Answer is:B
To find the angle at which the steel ball rebounds after striking a fixed smooth steel plate, we can follow these steps:
Step 1: Understanding the Problem
The steel ball strikes the plate at an angle θ with the vertical. The coefficient of restitution e determines how much of the vertical component of the velocity is retained after the collision.
Step 2: Break Down the Velocity Components
Let the initial velocity of the steel ball be u. We can break this velocity into two components:
- The horizontal component ux=usinθ
- The vertical component uy=ucosθ
Step 3: Analyze the Collision
Since the plate is smooth and fixed, the horizontal component of the velocity ux remains unchanged after the collision:
- vx=ux=usinθ
The vertical component of the velocity changes due to the collision. According to the coefficient of restitution e:
- vy=−e⋅uy=−e⋅(ucosθ)
Step 4: Find the Rebound Angle
The rebound angle α can be found using the tangent function, which relates the vertical and horizontal components of the velocity after the collision:
tanα=vyvx
Substituting the values we found:
tanα=−e⋅(ucosθ)usinθ
Step 5: Simplify the Expression
The u cancels out:
tanα=−e⋅cosθsinθ
This can be rewritten as:
tanα=−e⋅cotθ
Step 6: Find the Angle α
To find α, we take the arctangent:
α=tan−1(−e⋅cotθ)
Final Result
Thus, the angle at which the rebound will take place is:
α=tan−1(tanθe)
The steel ball strikes the plate at an angle θ with the vertical. The coefficient of restitution e determines how much of the vertical component of the velocity is retained after the collision.
Let the initial velocity of the steel ball be u. We can break this velocity into two components:
- The horizontal component ux=usinθ
- The vertical component uy=ucosθ
Since the plate is smooth and fixed, the horizontal component of the velocity ux remains unchanged after the collision:
- vx=ux=usinθ
- vy=−e⋅uy=−e⋅(ucosθ)
The rebound angle α can be found using the tangent function, which relates the vertical and horizontal components of the velocity after the collision:
tanα=vyvx
tanα=−e⋅(ucosθ)usinθ
The u cancels out:
tanα=−e⋅cosθsinθ
This can be rewritten as:
tanα=−e⋅cotθ
To find α, we take the arctangent:
α=tan−1(−e⋅cotθ)
Thus, the angle at which the rebound will take place is:
α=tan−1(tanθe)
Similar Questions
A force P acts on a smooth block of mass m placed on a horizontal floor at an angle θ with with horizontal on the block

View SolutionA particle strikes a smooth horizontal surface at an angle of 45∘ with a velocity of 100m/s and rebounds. If the coefficient of restitution between the floor and the particle is 0.57 then the angle which the velocity of the particle after it rebounds will make with the floor is
View Solution
Knowledge Check
A ball is dropped from a height h on the ground If the coefficient of restitution is e, the height to which the ball goes up after it rebounds for the nth time is
Ahe2nBhenCe2nhDhe2nA ball is dropped on to a horizontal plate from a height h = 9 m above it. If the coefficient of restitution is e=1/2, the total distance travelled before the ball comes to rest is
A10 mB15 mC20 mD25 m
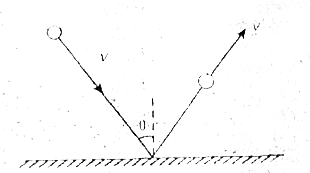
A ball moving with speed v collides with a horizontal smooth surface at an angle θ with normal to surface as shown in figure. If coefficient of restitution of collision is e, then find v'
View SolutionA ball is dropped from height 'H' onto a horizontal surface. If the coefficient of restitution is 'e' then the total time after which it comes to rest is
View SolutionA ball is dropped from height 'H' onto a horizontal surface. If the coefficient of restitution is 'e' then the total time after which it comes to rest is
View SolutionA ball is projected in a direction inclined to the vertical and bounces on a smooth horizontal plane. The range of one rebound is R. If the coefficient of restitution is e, then range of the next rebound is
View SolutionA rod of mass m is released on smooth horizontal surface making angle θ with horizontal. Then which of the following statement is correct ?
View SolutionA particle strikes a smooth horizontal surface at an angle θ>0 and it rebounds at an angle ϕ as shown
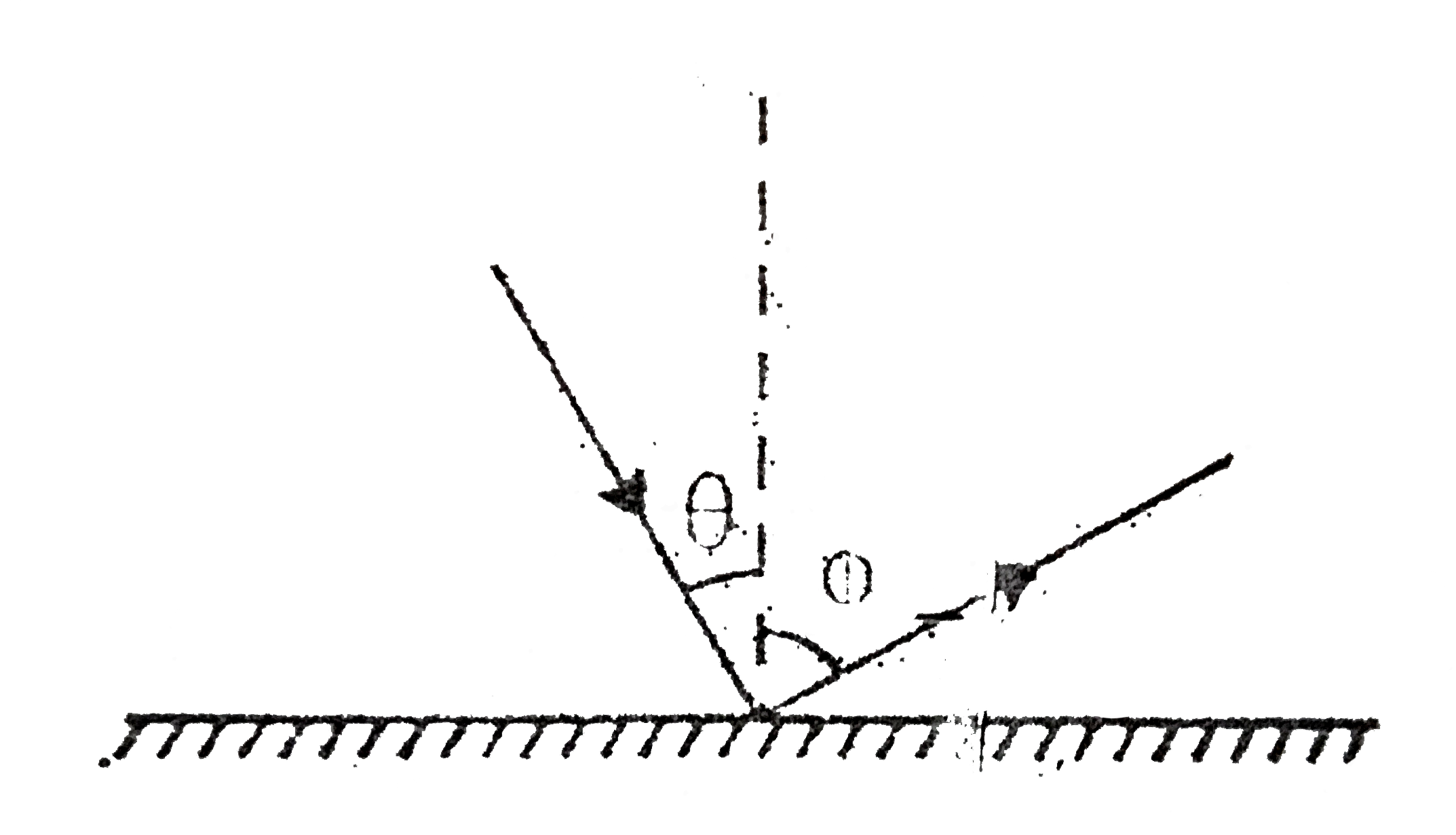
View Solution
