A pendulum clock ( fitted with a small heavy bob that is connected with a metal rod ) is 3 seconds fast each day at a temperature of 15∘C and 2 seconds slow at a temperature of 30∘C. Find the temperature ( in ∘C ) at which will show the correct time.
The correct Answer is:24.00∘C
To solve the problem of finding the temperature at which the pendulum clock shows the correct time, we can follow these steps:
Step 1: Understand the problem
The pendulum clock is 3 seconds fast at 15°C and 2 seconds slow at 30°C. We need to find a temperature T between 15°C and 30°C where the clock shows the correct time.
Step 2: Set up the equations
We can denote the fractional loss of time as:
- At 15°C: The clock is fast by 3 seconds per day.
- At 30°C: The clock is slow by 2 seconds per day.
The total time in a day is 86400 seconds (24 hours).
We can express the time error in terms of the temperature:
1. For 15°C:
3 seconds86400 seconds=12α(T−15)
2. For 30°C:
−2 seconds86400 seconds=12α(30−T)
Step 3: Simplify the equations
From the first equation:
386400=12α(T−15)
Multiplying both sides by 86400:
3=12α(T−15)⋅86400
This simplifies to:
α(T−15)=686400=114400
(Equation 1)
From the second equation:
−286400=12α(30−T)
Multiplying both sides by 86400:
−2=12α(30−T)⋅86400
This simplifies to:
α(30−T)=−486400=−121600
(Equation 2)
Step 4: Solve the equations
Now we have two equations:
1. α(T−15)=114400
2. α(30−T)=−121600
Dividing Equation 1 by Equation 2:
T−1530−T=114400−121600=−2160014400=−32
Cross-multiplying gives:
2(T−15)=−3(30−T)
Expanding:
2T−30=−90+3T
Rearranging:
T=60
T−3T=−90+30
−T=−60⟹T=24
Step 5: Conclusion
The temperature at which the pendulum clock shows the correct time is T=24°C.
The pendulum clock is 3 seconds fast at 15°C and 2 seconds slow at 30°C. We need to find a temperature T between 15°C and 30°C where the clock shows the correct time.
We can denote the fractional loss of time as:
- At 15°C: The clock is fast by 3 seconds per day.
- At 30°C: The clock is slow by 2 seconds per day.
1. For 15°C:
3 seconds86400 seconds=12α(T−15)
2. For 30°C:
−2 seconds86400 seconds=12α(30−T)
From the first equation:
386400=12α(T−15)
Multiplying both sides by 86400:
3=12α(T−15)⋅86400
This simplifies to:
α(T−15)=686400=114400
(Equation 1)
−286400=12α(30−T)
Multiplying both sides by 86400:
−2=12α(30−T)⋅86400
This simplifies to:
α(30−T)=−486400=−121600
(Equation 2)
Now we have two equations:
1. α(T−15)=114400
2. α(30−T)=−121600
T−1530−T=114400−121600=−2160014400=−32
2(T−15)=−3(30−T)
Expanding:
2T−30=−90+3T
Rearranging:
T=60
T−3T=−90+30
−T=−60⟹T=24
The temperature at which the pendulum clock shows the correct time is T=24°C.
Similar Questions
A pendulum clock is 5 sec. Slow at a temperature 30∘C and 10 sec. fast at a temperature of 15∘C, At what temperature does it give the correct time-
View SolutionA clock with a metallic pendulum gains 5 s each day at a temperature of 15∘C and loses 10 s each day at a temperature of 30∘C. Find the coefficient of thermal expansion of the pendulum metal.
View SolutionA clock with a metallic pendulum gains 6 seconds each day when the temperature is 20∘C and loses 6 second when the temperature is 40∘C. Find the coefficient of linear expansions of the metal.
View SolutionA clock with a metallic pendulum 4 second fast each day at a temperature of 20°C. The koi efficient of linear expansion of given metal is 2.77×10−5°C−1. The temperature at which clock gives correct reading is nearly
View SolutionA metallic sphere cools from 50∘C to 40∘C in 300 seconds. If the room temperature is 20∘C then its temperature in next 5 minutes will be -
View SolutionIf a liquid takes 30 seconds in cooling of 80∘C to 70∘C and 70 seconds in cooling 60∘C to 50∘C, then find the room temperature.
View SolutionSix identical conducting rods are connected as shown in figure. In steady state temperature of point ϕ1 is fixed at 100∘C and temperature of ϕ6 at −80∘C

Q. What is the temperature of ϕ3?
View SolutionSix identical conducting rods are connected as shown in figure. In steady state temperature of point ϕ1 is fixed at 100∘C and temperature of ϕ6 at −80∘C
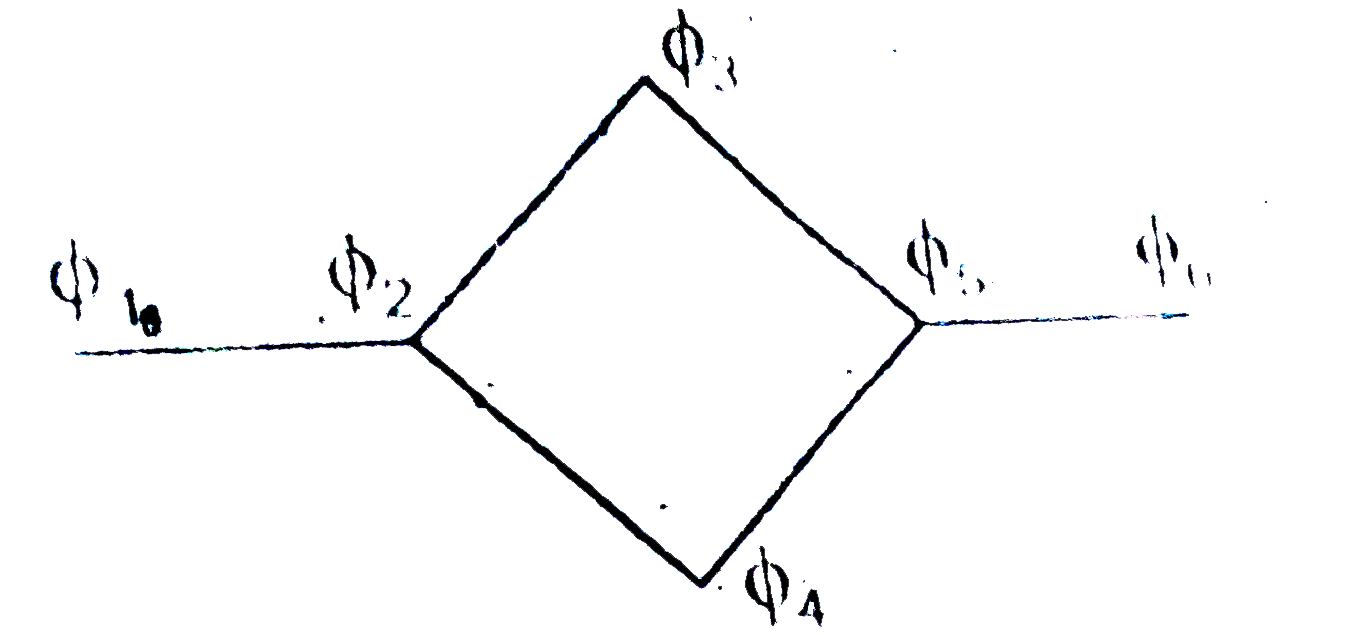
Q. What is the temperature of ϕ2?
View SolutionA cup of tea cools from 80∘C to 60∘C in 40 seconds. The ambient temperature is 30∘C. In cooling from 60∘C to 50∘C, it will take time:
View SolutionThe two rods show in figure have identical geometrical dimension.. They are in contact with two heat baths at temperature 100∘C . And 0∘C . The temperature of the junction is 70∘C . Find the temperature of the junction if the rods are interchanged.

View Solution
