0.01 M solution of KCl and CaCl2 are separately prepared in water. The freezing point of KCl is found to be −2∘C. What is the freezing point of CaCl2 aq. Solution if it is completely ionized?
−3∘C
+3∘C
−2∘C
−4∘C
To solve the problem, we need to determine the freezing point of a 0.01 M solution of CaCl₂ based on the freezing point depression observed for a 0.01 M solution of KCl.
1. Understanding Freezing Point Depression:
The freezing point depression (ΔTf) is the decrease in the freezing point of a solvent (water in this case) when a solute is dissolved in it. It is given by the formula:
ΔTf=i⋅Kf⋅m
where:
- i = van 't Hoff factor (number of particles the solute dissociates into)
- Kf = freezing point depression constant (for water, Kf=1.86°C⋅kg/mol)
- m = molality of the solution
2. Finding the van 't Hoff Factor for KCl:
KCl dissociates into two ions:
KCl→K++Cl−
Thus, the van 't Hoff factor i for KCl is:
iKCl=2
3. Calculating ΔTf for KCl:
Given that the freezing point of KCl solution is −2°C, we can say:
ΔTf,KCl=−2°C
4. Finding the van 't Hoff Factor for CaCl₂:
CaCl₂ dissociates into three ions:
CaCl2→Ca2++2Cl−
Thus, the van 't Hoff factor i for CaCl₂ is:
iCaCl2=3
5. Setting Up the Proportionality:
Since ΔTf is directly proportional to i, we can set up the following relationship:
ΔTf,KClΔTf,CaCl2=iKCliCaCl2
6. Substituting Known Values:
Substituting the known values into the equation:
−2°CΔTf,CaCl2=23
7. Cross-Multiplying to Solve for ΔTf of CaCl₂:
Cross-multiplying gives:
−2°C⋅3=2⋅ΔTf,CaCl2
−6°C=2⋅ΔTf,CaCl2
ΔTf,CaCl2=−3°C
8. Calculating the Freezing Point of CaCl₂ Solution:
The freezing point of the CaCl₂ solution is calculated by subtracting ΔTf from the freezing point of pure water (0°C):
Freezing Point=0°C−(−3°C)=0°C+3°C=3°C
Final Answer:
The freezing point of the CaCl₂ aqueous solution is 3°C.
The freezing point depression (ΔTf) is the decrease in the freezing point of a solvent (water in this case) when a solute is dissolved in it. It is given by the formula:
ΔTf=i⋅Kf⋅m
where:
- i = van 't Hoff factor (number of particles the solute dissociates into)
- Kf = freezing point depression constant (for water, Kf=1.86°C⋅kg/mol)
- m = molality of the solution
KCl dissociates into two ions:
KCl→K++Cl−
Thus, the van 't Hoff factor i for KCl is:
iKCl=2
Given that the freezing point of KCl solution is −2°C, we can say:
ΔTf,KCl=−2°C
CaCl₂ dissociates into three ions:
CaCl2→Ca2++2Cl−
Thus, the van 't Hoff factor i for CaCl₂ is:
iCaCl2=3
Since ΔTf is directly proportional to i, we can set up the following relationship:
ΔTf,KClΔTf,CaCl2=iKCliCaCl2
Substituting the known values into the equation:
−2°CΔTf,CaCl2=23
Cross-multiplying gives:
−2°C⋅3=2⋅ΔTf,CaCl2
−6°C=2⋅ΔTf,CaCl2
ΔTf,CaCl2=−3°C
The freezing point of the CaCl₂ solution is calculated by subtracting ΔTf from the freezing point of pure water (0°C):
Freezing Point=0°C−(−3°C)=0°C+3°C=3°C
The freezing point of the CaCl₂ aqueous solution is 3°C.
Similar Questions
Knowledge Check
The lowest freezing point of 0.1 M aqueous solution is of:
AK2SO4BNaClCUreaDGlucoseA solution of urea in water has a boiling point of 100.18∘C . Calculate the freezing point of the solution.
A−0.4539∘CB−0.6539∘CC−0.4859∘CD−0.5539∘C
The freezing point of water is depressed by 0.37∘C in a 0.01 molar NaCI solution. The freezing point of 0.02 molar solution is depressed by
View SolutionA 1.24 M aqueous solution of KI has a density of 1.15gcm−3. What is the freezing point of the solution if the KI is completely dissociated in the solution?
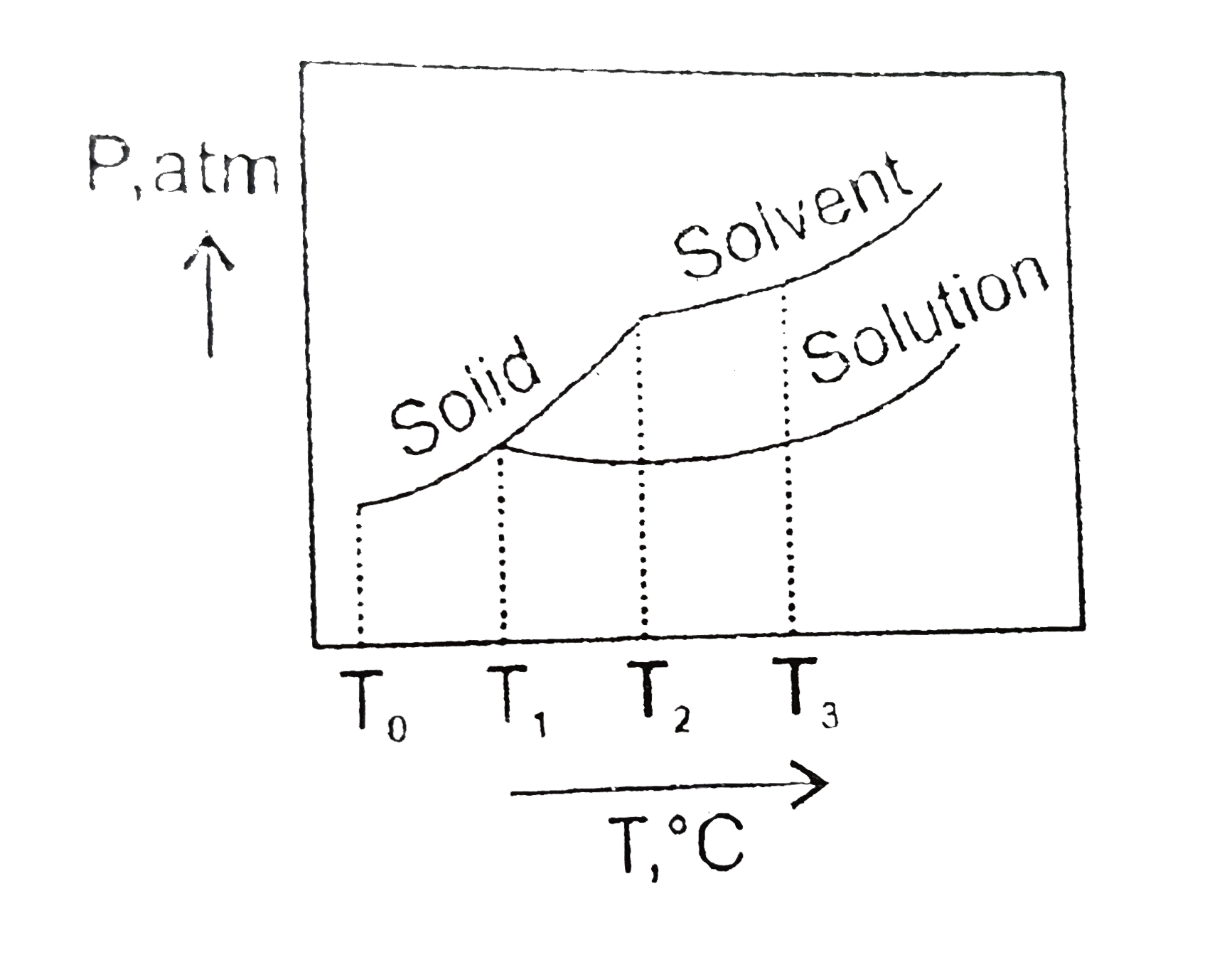
View SolutionWhat is the normal freezing point of the solution represented by the phase diagram ?
View Solution0.1 M solution of which of the following compounds will have the lowest freezing point ?
View SolutionThe molal depression constant for water is 1.86∘C. The freezing point of a 0.05−molal solution of a non-electrolyte in water is
View SolutionThe boiling point of 0.1MKCl solution is ………..than 100∘C.
View Solution
