Question

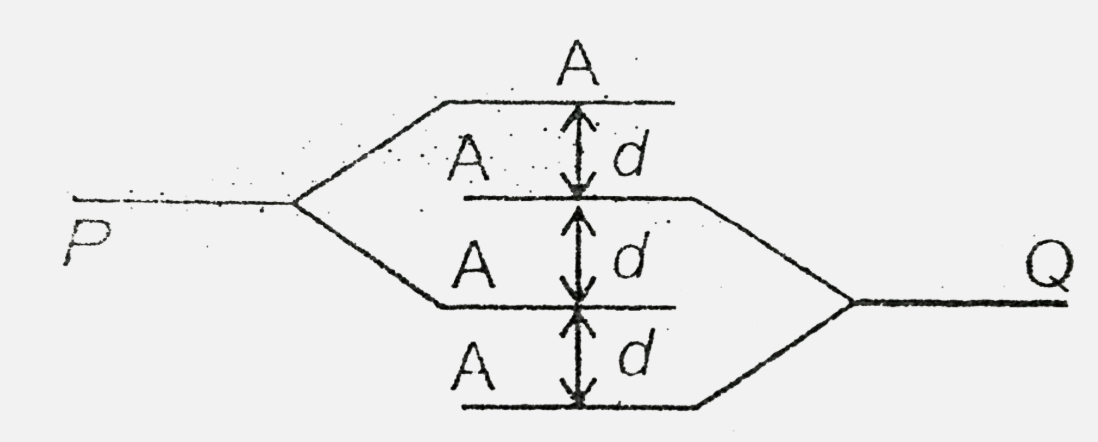
Positive charge q is given to each plate of a parallel plate air capacitor having area of each plate A and seperation between them, d. Then find
(i) Capacitance of the system. (ii) Charges appearing on each surface of plates
(iii) Electric field between the plates (iv) Potential diffrence between the plates
(v) Energy stored between hte plates

Options

A. since both the plates are identically charged, therefore, capacitance becomes equal to zero
B. energy stored in the space between the capacitor plates is equal to `(q^(2))/(epsi_(0)A^(2))`.
C. no charge appears on inner surface of the plates
D. potential difference between the plates is equal to `(2qd)/(epsi_(0)A)`

Answer

C

Explanation

To solve the problem step by step, we will find the required parameters for the parallel plate capacitor with the given conditions.

Step 1: Find the Capacitance of the System
The formula for the capacitance \( C \) of a parallel plate capacitor is given by:
\( C = \frac{\varepsilon_0 A}{d} \)
where:
- \( \varepsilon_0 \) is the permittivity of free space (approximately \( 8.85 \times 10^{-12} \, \text{F/m} \)),
- \( A \) is the area of each plate,
- \( d \) is the separation between the plates.

Step 2: Charges Appearing on Each Surface of Plates
Since a positive charge \( Q \) is given to each plate, the charges on the plates will distribute as follows:
- The outer surfaces of both plates will have a charge of \( +Q \).
- The inner surfaces of both plates will have a charge of \( 0 \) because the charges are static and there is no movement of charge within the capacitor.

Step 3: Electric Field Between the Plates
The electric field \( E \) between the plates of a parallel plate capacitor is given by:
\( E = \frac{\sigma}{\varepsilon_0} \)
where \( \sigma \) is the surface charge density given by:
\( \sigma = \frac{Q}{A} \)
Thus, substituting \( \sigma \) into the electric field equation:
\( E = \frac{Q}{A \varepsilon_0} \)

Step 4: Potential Difference Between the Plates
The potential difference \( V \) between the plates is given by:
\( V = E \cdot d \)
Substituting the expression for \( E \):
\( V = \left(\frac{Q}{A \varepsilon_0}\right) \cdot d = \frac{Qd}{A \varepsilon_0} \)

Step 5: Energy Stored Between the Plates
The energy \( U \) stored in the capacitor is given by:
\( U = \frac{1}{2} C V \)
Substituting the expressions for \( C \) and \( V \):
\( U = \frac{1}{2} \left(\frac{\varepsilon_0 A}{d}\right) \left(\frac{Qd}{A \varepsilon_0}\right) = \frac{1}{2} \frac{Q^2}{\varepsilon_0 A} \)

Summary of Results
1. Capacitance: \( C = \frac{\varepsilon_0 A}{d} \)
2. Charges on Plates: Outer surfaces: \( +Q \); Inner surfaces: \( 0 \)
3. Electric Field: \( E = \frac{Q}{A \varepsilon_0} \)
4. Potential Difference: \( V = \frac{Qd}{A \varepsilon_0} \)
5. Energy Stored: \( U = \frac{1}{2} \frac{Q^2}{\varepsilon_0 A} \)