Assuming that potential energy of spring is zero when it is stretched by x02, its potential energy when it is compressed by x0 is
38kx20
−34kx20
34kx20
18kx20
The correct Answer is:A
To solve the problem, we need to find the potential energy of a spring when it is compressed by x0, given that the potential energy is zero when it is stretched by x02.
1. Understanding the Potential Energy of a Spring:
The potential energy (PE) stored in a spring is given by the formula:
U=12kx2
where k is the spring constant and x is the displacement from the mean position.
2. Condition 1 - Potential Energy at x02:
We are told that the potential energy is zero when the spring is stretched by x02. Let's denote this position as point A.
UA=0whenx=x02
3. Setting the Mean Position:
Since the potential energy is defined to be zero at point A, we can assume that point A is the new mean position for our calculations.
4. Condition 2 - Potential Energy when Compressed by x0:
Now, we need to find the potential energy when the spring is compressed by x0. Let's denote this position as point B.
UB=12kx2wherex=−x0
(negative because it is compressed).
5. Calculating Potential Energy at Point B:
Substituting x=−x0 into the potential energy formula:
UB=12k(−x0)2=12kx20
6. Finding the Change in Potential Energy:
Since we defined the potential energy at point A (stretched by x02) to be zero, we need to calculate the potential energy at point A:
UA=12k(x02)2=12kx204=18kx20
7. Final Calculation of Potential Energy at Point B:
The potential energy at point B (compressed by x0) is:
UB=12kx20
Thus, the potential energy when compressed by x0 is:
UB−UA=12kx20−18kx20
Finding a common denominator:
UB−UA=48kx20−18kx20=38kx20
Conclusion:
The potential energy when the spring is compressed by x0 is:
38kx20
The potential energy (PE) stored in a spring is given by the formula:
U=12kx2
where k is the spring constant and x is the displacement from the mean position.
We are told that the potential energy is zero when the spring is stretched by x02. Let's denote this position as point A.
UA=0whenx=x02
Since the potential energy is defined to be zero at point A, we can assume that point A is the new mean position for our calculations.
Now, we need to find the potential energy when the spring is compressed by x0. Let's denote this position as point B.
UB=12kx2wherex=−x0
(negative because it is compressed).
Substituting x=−x0 into the potential energy formula:
UB=12k(−x0)2=12kx20
Since we defined the potential energy at point A (stretched by x02) to be zero, we need to calculate the potential energy at point A:
UA=12k(x02)2=12kx204=18kx20
The potential energy at point B (compressed by x0) is:
UB=12kx20
Thus, the potential energy when compressed by x0 is:
UB−UA=12kx20−18kx20
Finding a common denominator:
UB−UA=48kx20−18kx20=38kx20
The potential energy when the spring is compressed by x0 is:
38kx20
Similar Questions
Knowledge Check
The potential energy of a spring is the minimum when it is
AcompressedBextendedCat its natural lengthDat its natural length but is kept at a height above the groundA long spring is stretched by 2 cm and the potential energy is V. IF the spring is stretched by 19 cm, its potential energy will be
A361V/4BV/5C5 VD25 VA long spring is stretched by 2 cm, its potential energy is U. IF the spring is stretched by 10 cm, the potential energy stored in it will be
AU/25BU/5C5 UD25 U
The potential energy of a long spring when stretched by 2 cm is U. If the spring is stretched by 8 cm the potential energy stored in it is:-
View SolutionIn figure, k=100N/m,M=1kg and F=10N
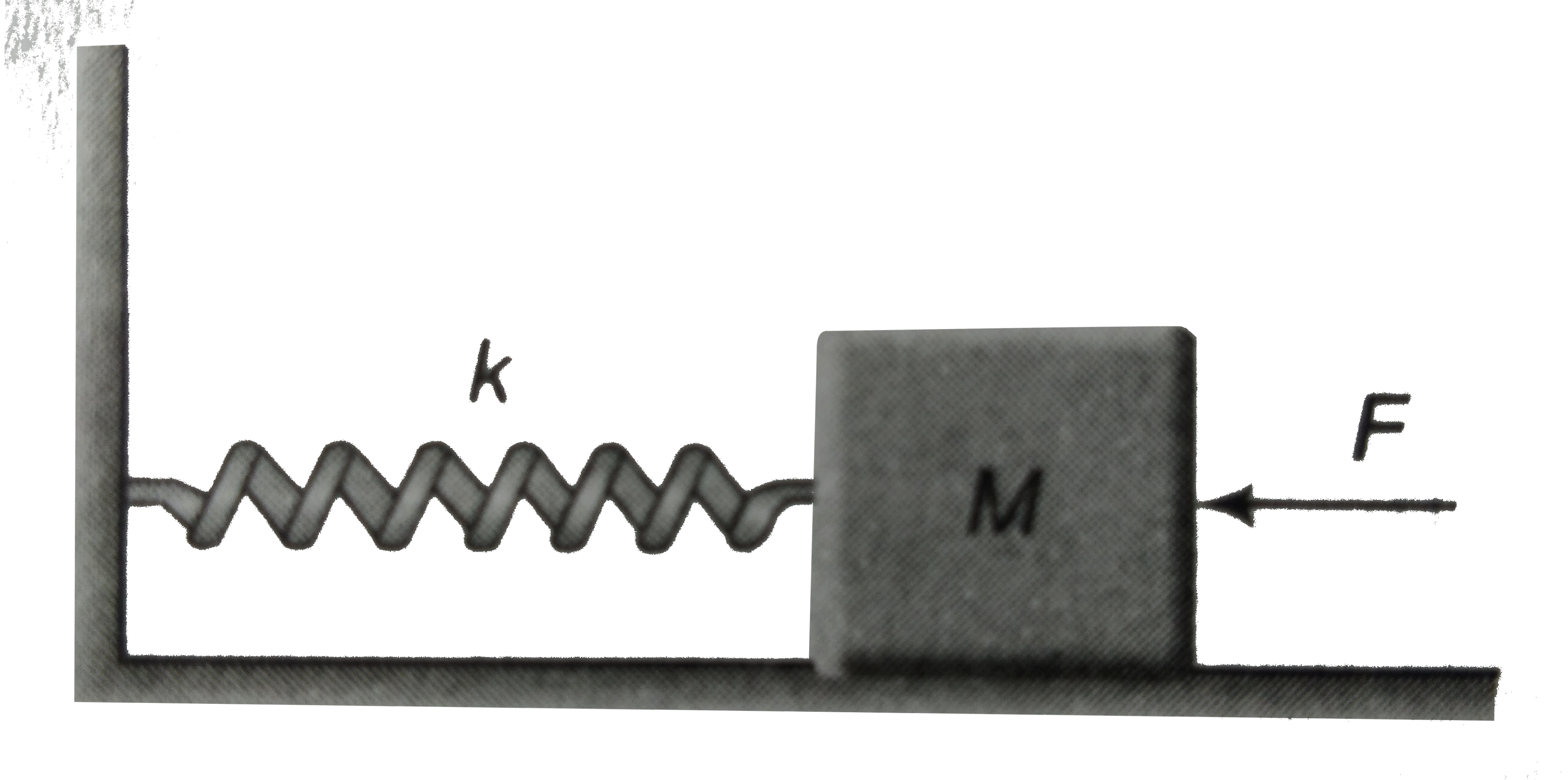
(a) Find the compression of the spring in the equilibrium position
(b) A sharp blow by some external agent imparts a speed of 2m/s to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at this instant.
(c) Find the time period of the resulting simple harmonic motion.
(d) Find the amplitude.
(e) Write the potential energy of the spring when the block is at the left estreme.
(f) Write the potential energy of the spring when the block is at the right extreme.
The answers of (b), (e) and (f) are different. Explain why this does not violate the principle of conservation of energy ?
View SolutionA long elastic spring is stretched by 2cm and its potential energy is U. If the spring is stretched by 10cm, the PE will be
View SolutionIf the total energy of a simple harmonic oscillator is E, then its potential energy, when it is halfway to its endpoint will be
View SolutionWhen a long spring is stretched by 2 cm, its potential energy is V. If the spring is stretched by 10 cm, the potential energy in it will be
View Solution
