Two point white dots are 1mm apart on a black paper. They are viewed by eye of pupil diameter 3mm. Approximately, what is the maximum distance at which these dots can be resolved by the eye? [Take wavelelngth of light =500nm]
6 m
3m
1m
5m
The correct Answer is:D
To solve the problem of determining the maximum distance at which two point white dots can be resolved by the human eye, we can use the formula for the resolving power of an optical system. The key steps are as follows:
Step 1: Understand the Parameters
We have:
- Distance between the dots, d=1 mm=1×10−3 m
- Diameter of the pupil, Y=3 mm=3×10−3 m
- Wavelength of light, λ=500 nm=500×10−9 m
Step 2: Use the Resolving Power Formula
The resolving power of the eye can be expressed as:
yd≥1.22λD
Where:
- y is the diameter of the pupil,
- d is the distance between the two dots,
- D is the maximum distance at which the dots can be resolved.
Rearranging the formula to find D:
D≤d⋅Y1.22λ
Step 3: Substitute the Values
Substituting the known values into the equation:
D≤(1×10−3 m)⋅(3×10−3 m)1.22⋅(500×10−9 m)
Step 4: Calculate the Denominator
Calculating the denominator:
1.22⋅(500×10−9)=6.1×10−7 m
Step 5: Calculate the Numerator
Calculating the numerator:
(1×10−3)⋅(3×10−3)=3×10−6 m2
Step 6: Final Calculation
Now, substituting back into the equation for D:
D≤3×10−66.1×10−7≈4.92 m
Step 7: Conclusion
Thus, the maximum distance D at which the two dots can be resolved by the eye is approximately:
D≈5 m
We have:
- Distance between the dots, d=1 mm=1×10−3 m
- Diameter of the pupil, Y=3 mm=3×10−3 m
- Wavelength of light, λ=500 nm=500×10−9 m
The resolving power of the eye can be expressed as:
yd≥1.22λD
Where:
- y is the diameter of the pupil,
- d is the distance between the two dots,
- D is the maximum distance at which the dots can be resolved.
D≤d⋅Y1.22λ
Substituting the known values into the equation:
D≤(1×10−3 m)⋅(3×10−3 m)1.22⋅(500×10−9 m)
Calculating the denominator:
1.22⋅(500×10−9)=6.1×10−7 m
Calculating the numerator:
(1×10−3)⋅(3×10−3)=3×10−6 m2
Now, substituting back into the equation for D:
D≤3×10−66.1×10−7≈4.92 m
Thus, the maximum distance D at which the two dots can be resolved by the eye is approximately:
D≈5 m
Similar Questions
The diameter of human eye lens is 2mm. What should be the minimum separation between two points situated at 50m from eye, to resolve tham. Take wavelength of light =5000Å.
View SolutionThe diameter of human eye lens is 2mm. What should be the minimum separation between two points situated at 50m from eye, to resolve tham. Take wavelength of light =5000Å.
View Solution
Knowledge Check
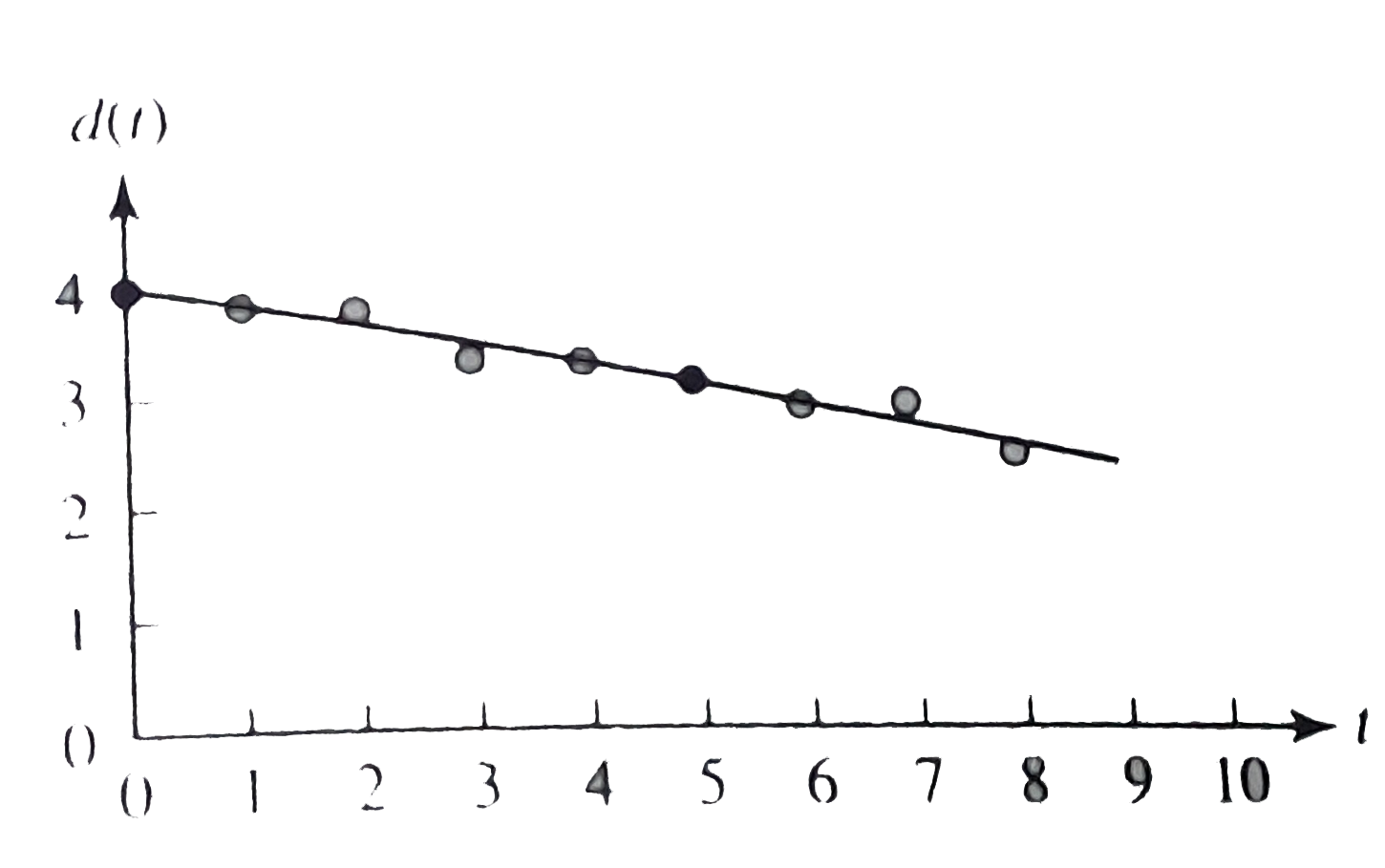
Joe bikes 4 kilometers to school . Because of the traffic and road conditions along the way , his speed varies. The dots on the graph above show his distance from the school at various times , starting at home at t=0 (black dot). After 5 minutes Joe is 3.2 kilometers from the school (second black dot). The data show that his speed is almost constant , and his distance from the school can be approximated by a straight line . The graph of the function that models Joe's distance from school as a function of time , in minutes , is shown as a solid line. Which of the following equations best represents this function ?Ad(t)=-0.5 t+4Bd(t)=-0.16 t +4Cd(t)=-6.25 t+4Dd(t)=0.16 t +4
For what distance is ray optics a good approximation when the aperture is 3 mm wide and the wavelength is 500 nm ?
View SolutionA telescope of aperture diameter 5m is used to observe the moon from the earth. Distance between the moon and earth is 4×105 km. Determine the minimum distance between two points on the moon's surface which can be resolved using this telescope. (Wave length of light is 5893A∘.
View SolutionA telescope of aperture diameter 5m is used to observe the moon from the earth. Distance between the moon and earth is 4×105 km. Determine the minimum distance between two points on the moon's surface which can be resolved using this telescope. (Wave length of light is 5893A∘.
View SolutionIn Young's double slit experiment ,momochromatic light of wavelength 630nm illuminates the pair of slits and produces an interface pattern pattern in which two consecutive bright frings are separated by 8.1mm. Another source of monochromatic light produce the interface pattern in which the two consecutive bright fringes are seperated by 7.2mm, find the wavelength of light from the second sources . What is the effect to on the interference fringes if the monochromatic sources is replaced by a source of white light ?
View SolutionTwo point sources distant 0.1m away viewed by a telescope. The objective is covered by a screen having a hole of 1mm width. If the wavelength fo the light used is 6550A0, then the maximum distance at which the two sources are seen just resolved, will be nearly
View SolutionAssuming human pupil to have a radius of 0.25 cm and a comfortable viewing distance of 25 cm, the minimum separation between two objects than human eye can resolve at 500nm wavelength is :
View SolutionA normal human cye can see an object making an angle of 1.8∘ at the eye. What is the

approximate height of object which can be seen by an eye placed at a distance of 1 m from the object.
View Solution
