For a particle executing SHM, the kinetic energy (K) is given by K=K0cos2ωt. The equation for its displacement is
(k0mω2)1/2sinωt
(2k0mω2)1/2sinωt
(2ω2mk0)1/2sinωt
(2k0mω)1/2sinωt
The correct Answer is:B
To solve the problem, we need to find the equation for the displacement of a particle executing Simple Harmonic Motion (SHM) given that its kinetic energy K is expressed as K=K0cos2(ωt).
1. Assume the Displacement Equation:
We start by assuming the standard form of the displacement in SHM:
y(t)=Asin(ωt)
where A is the amplitude.
2. Find the Velocity:
To find the velocity, we differentiate the displacement with respect to time t:
v(t)=dydt=ddt(Asin(ωt))=Aωcos(ωt)
3. Express Kinetic Energy:
The kinetic energy K of the particle can be expressed in terms of its velocity:
K=12mv2
Substituting the expression for v(t):
K=12m(Aωcos(ωt))2=12mA2ω2cos2(ωt)
4. Compare with Given Kinetic Energy:
We are given that:
K=K0cos2(ωt)
By comparing the two expressions for kinetic energy, we have:
12mA2ω2cos2(ωt)=K0cos2(ωt)
This implies:
12mA2ω2=K0
5. Solve for Amplitude A:
Rearranging the equation gives:
A2=2K0mω2
Taking the square root:
A=√2K0mω2
6. Substitute Amplitude into Displacement Equation:
Now substitute the value of A back into the displacement equation:
y(t)=Asin(ωt)=√2K0mω2sin(ωt)
7. Final Displacement Equation:
Therefore, the equation for the displacement of the particle is:
y(t)=√2K0mω2sin(ωt)
We start by assuming the standard form of the displacement in SHM:
y(t)=Asin(ωt)
where A is the amplitude.
To find the velocity, we differentiate the displacement with respect to time t:
v(t)=dydt=ddt(Asin(ωt))=Aωcos(ωt)
The kinetic energy K of the particle can be expressed in terms of its velocity:
K=12mv2
Substituting the expression for v(t):
K=12m(Aωcos(ωt))2=12mA2ω2cos2(ωt)
We are given that:
K=K0cos2(ωt)
By comparing the two expressions for kinetic energy, we have:
12mA2ω2cos2(ωt)=K0cos2(ωt)
This implies:
12mA2ω2=K0
Rearranging the equation gives:
A2=2K0mω2
Taking the square root:
A=√2K0mω2
Now substitute the value of A back into the displacement equation:
y(t)=Asin(ωt)=√2K0mω2sin(ωt)
Therefore, the equation for the displacement of the particle is:
y(t)=√2K0mω2sin(ωt)
Similar Questions
Energy of particle executing SHM depends upon
View SolutionFor a particle executing SHM, the displacement x is given by x=Acosωt. Identify the graph which represents the variation of potential energy (PE) as a function of time t and displacement x.

(a) I,III
(b) II,IV (c ) II,III
(d) I,IVView SolutionThe displacement of a particle executing simple harmonic motion is given by y=A0+Asinωt+Bcosωt. Then the amplitude of its oscillation is given by
View SolutionThe particle executing simple harmonic motion has a kinetic energy K0cos2ωt. The maximum values of the potential energy and the energy are respectively
View SolutionA particle is executing SHM, whose equation is given below
x=Acos(ωt+ϕ). If t=0 , particle is mean position going towards positive x-direction then ϕ is equal toView SolutionA particle executing SHM has potential energy U0sin2ωt. The maximum kinetic energy and total energy respectively are
View SolutionSTATEMENT-1, The total energy of a particle executing S.H.M( of given amplitude) of depends on the mass of particle but does not depend on its displacement from mean position
and
STATEMENT -2 : The total energy of a particle executing S.H.M. ismaximum at the mean position.View SolutionA body executes simple harmonic motion. The potential energy (P.E), the kinetic energy (K.E) and energy (T.E) are measured as a function of displacement x. Which of the following staements is true?
View SolutionA particle executes SHM with an amplitude of 10cm and frequency 2Hz, at t=0, the particle is at point where potential energy and kinetic energy are same. Find the equation of displacement of particle.
View SolutionThe figure shows the displacement-time graph of a particle executing SHM. If the time period of oscillation is 2s, then the equation of motion is given by
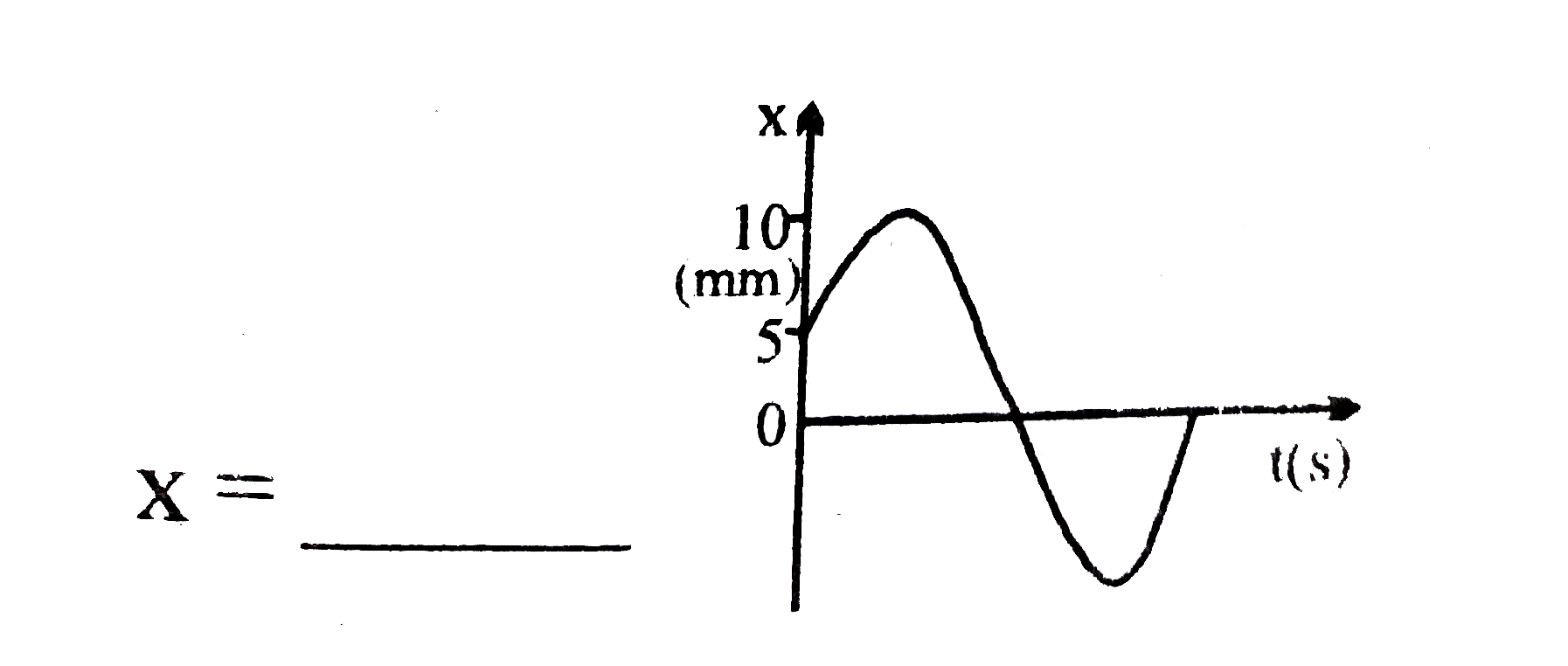 View Solution
View Solution

