A uniform disk of mass 300kg is rotating freely about a vertical axis through its centre with constant angular velocity ω. A boy of mass 30kg starts from the centre and moves along a radius to the edge of the disk. The angular velocity of the disk now is
(a)ω06
(b)ω05
(c)4ω05
(d)5ω06
The correct Answer is:D
To solve the problem, we need to apply the principle of conservation of angular momentum. Here's a step-by-step breakdown of the solution:
Step 1: Understand the System
We have a uniform disk with a mass of 300 kg rotating about a vertical axis with an initial angular velocity ω0. A boy with a mass of 30 kg starts from the center of the disk and moves to its edge.
Step 2: Write the Expression for Angular Momentum
The angular momentum L of a rotating system is given by the product of its moment of inertia I and its angular velocity ω:
L=I⋅ω
Step 3: Calculate Initial Angular Momentum
Initially, the boy is at the center of the disk, so his contribution to the moment of inertia is zero. The moment of inertia of the disk is given by:
Idisk=12MR2
where M=300kg and R is the radius of the disk.
Thus, the initial angular momentum Li is:
Li=Idisk⋅ω0=(12⋅300⋅R2)⋅ω0=150R2ω0
Step 4: Calculate Final Angular Momentum
When the boy moves to the edge of the disk, his contribution to the moment of inertia becomes significant. The moment of inertia of the boy when he is at the edge is:
Iboy=mboy⋅R2=30⋅R2
Thus, the total moment of inertia when the boy is at the edge is:
If=Idisk+Iboy=12⋅300⋅R2+30⋅R2=150R2+30R2=180R2
The final angular momentum Lf is then:
Lf=If⋅ωf=180R2⋅ωf
Step 5: Apply Conservation of Angular Momentum
According to the conservation of angular momentum:
Li=Lf
Substituting the expressions we found:
150R2ω0=180R2ωf
Step 6: Solve for Final Angular Velocity
We can cancel R2 from both sides (assuming R≠0):
150ω0=180ωf
Now, solving for ωf:
ωf=150180ω0=56ω0
Conclusion
The final angular velocity of the disk when the boy reaches the edge is:
ωf=56ω0
We have a uniform disk with a mass of 300 kg rotating about a vertical axis with an initial angular velocity ω0. A boy with a mass of 30 kg starts from the center of the disk and moves to its edge.
The angular momentum L of a rotating system is given by the product of its moment of inertia I and its angular velocity ω:
L=I⋅ω
Initially, the boy is at the center of the disk, so his contribution to the moment of inertia is zero. The moment of inertia of the disk is given by:
Idisk=12MR2
where M=300kg and R is the radius of the disk.
Li=Idisk⋅ω0=(12⋅300⋅R2)⋅ω0=150R2ω0
When the boy moves to the edge of the disk, his contribution to the moment of inertia becomes significant. The moment of inertia of the boy when he is at the edge is:
Iboy=mboy⋅R2=30⋅R2
Thus, the total moment of inertia when the boy is at the edge is:
If=Idisk+Iboy=12⋅300⋅R2+30⋅R2=150R2+30R2=180R2
The final angular momentum Lf is then:
Lf=If⋅ωf=180R2⋅ωf
According to the conservation of angular momentum:
Li=Lf
Substituting the expressions we found:
150R2ω0=180R2ωf
We can cancel R2 from both sides (assuming R≠0):
150ω0=180ωf
Now, solving for ωf:
ωf=150180ω0=56ω0
The final angular velocity of the disk when the boy reaches the edge is:
ωf=56ω0
Similar Questions
A solid sphere of radius r and mass m rotates about an axis passing through its centre with angular velocity ω. Its K.E. Is
View SolutionA ring of radius r is rotating about a vertical axis along its diameter with constant angular velocity ω.A read of mass m remains at rest w.r.t. ring at the position shown in figure. Then w2 is:
_2019_CBT8_E01_015_Q01.png)
View Solution
Knowledge Check
A thin circular ring of mass M and radius r is rotating about its axis with a constant angular velocity ω. Two objects each of mass m are attached gently to the opposite ends of a diameter of the ring. The ring will now rotate with an angular velocity of
Aω(M−2m)(M+2m)BωM(M+2m)CωM(M+m)Dω(M+2m)M
A ring of radius r is rotating about a vertical axis along its diameter with constant angular velocity ω.A read of mass m remains at rest w.r.t. ring at the position shown in figure. Then w2 is:
_2019_CBT8_E01_015_Q01.png)
View SolutionA uniform disc of mass m and radius R rotates about a fixed vertical axis passing through its centre with angular velocity ω. A particle of same mass m and having velocity of 2ωR towards centre of the disc collides with the disc moving horizontally and sticks to its rim. Then
View SolutionA thin circular ring of mass M and radius R is rotating about its axis with a constant angular velocity omega. Four objects each of mass m, are kept gently to the opposite ends of two perpendicular diameters of the ring. The angular velocity of the ring will be
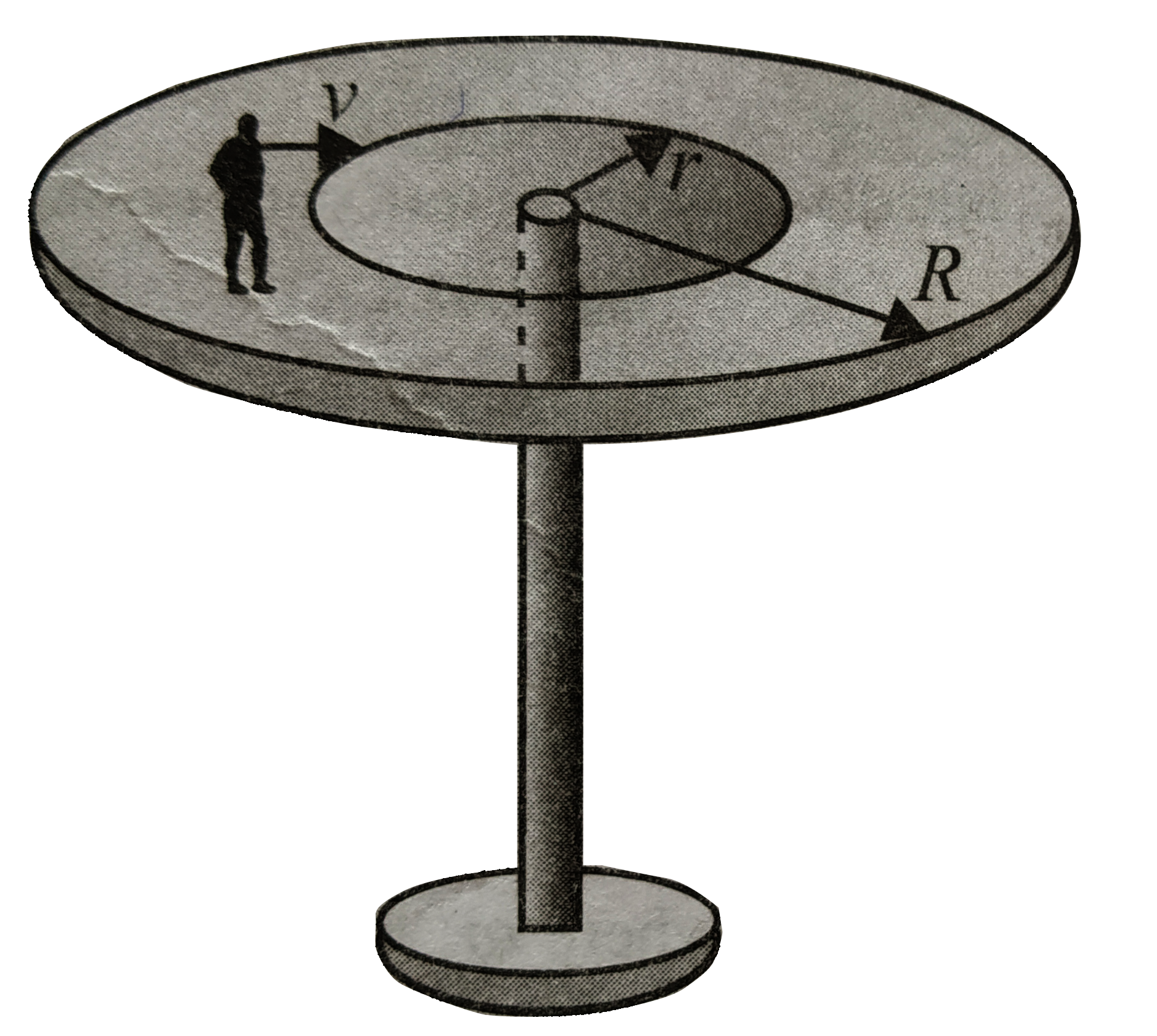
View SolutionA man of mass m stands on a horizontal platform in the shape of a disc of mass m and radius R, pivoted on a vertical axis thorugh its centre about which it can freely rotate. The man starts to move aroung the centre of the disc in a circle of radius r with a velocity v relative to the disc. Calculate the angular velocity of the disc.
View SolutionA thin circular ring of mass M and radius R is rotating about its axis with constant angular velocity ω. The objects each of mass m are attached gently to the ring. The wheel now rotates with an angular velocity.
View SolutionA ring of mass m and radius r rotates about an axis passing through its centre and perpendicular to its plane with angular velocity ω. Its kinetic energy is
View SolutionA thin circular ring of mass M and radius R is rotating in a horizontal plane about an axis vertical to its plane with a constant angular velocity ω. If two objects each of mass m be attached gently to the opposite ends of a diameter of the ring, the ring will then rotate with an angular velocity
View Solution
