For a weak electrolyte α1andα2 are in ratio of 1:2, for a given concentration ka1=2×10−4. What will be value of ka2?
8×10−4
2×10−4
4×10−4
1×10−4
The correct Answer is:A
To solve the problem, we need to find the value of ka2 given the following information:
1. The ratio of the dissociation constants α1 and α2 is 1:2.
2. The value of ka1 is 2×10−4.
Step 1: Write down the given ratios and values.
- We have α1α2=12.
- We know ka1=2×10−4.
Step 2: Use the relationship between dissociation constants.
- The relationship between the dissociation constants and the degree of dissociation is given by:
α1α2=√ka1ka2
Step 3: Substitute the known values into the equation.
- Plugging in the values we have:
12=√2×10−4ka2
Step 4: Square both sides to eliminate the square root.
- Squaring both sides gives:
(12)2=2×10−4ka2
14=2×10−4ka2
Step 5: Rearrange the equation to solve for ka2.
- Rearranging gives:
ka2=2×10−4×4
Step 6: Calculate ka2.
- Performing the multiplication:
ka2=8×10−4
Final Answer:
The value of ka2 is 8×10−4.
---
2. The value of ka1 is 2×10−4.
- We have α1α2=12.
- We know ka1=2×10−4.
- The relationship between the dissociation constants and the degree of dissociation is given by:
α1α2=√ka1ka2
- Plugging in the values we have:
12=√2×10−4ka2
- Squaring both sides gives:
(12)2=2×10−4ka2
14=2×10−4ka2
- Rearranging gives:
ka2=2×10−4×4
- Performing the multiplication:
ka2=8×10−4
The value of ka2 is 8×10−4.
Similar Questions
The degree of dissociation of weak electrolyde is inversely proportional to the square root fo concentration. It is called Ostwald's dilution law.
α=√Kac As the tempertaure increases, degree of dissociation will increase.
α1α2=√Ka1Ka2 if concentration is same.
α1α2=√c2c1 if acid is same.
a1 and a2 are in ratio of 1:2,Ka1=2×10−4. What will be Ka2?
View SolutionIf a1anda2 aare two non- collineaar unit vectors and if |a1+a2|=√3, ,then value of (a1−a2).(2a1−a2) is
View SolutionIf a1anda2 are two non- collinear unit vectors and if |a1+a2|=√3, ,then value of (a1−a2).(2a1−a2) is
View SolutionThe degree of dissociation of a weak monoprotic acid of concentration 1.2×10−3M having Ka=1.0×10−4 is
View SolutionTwo blocks are arranged as shown in figure. Find the ratio of a1/a2. (a1 is acceleration of m1 and a2 that of m2)
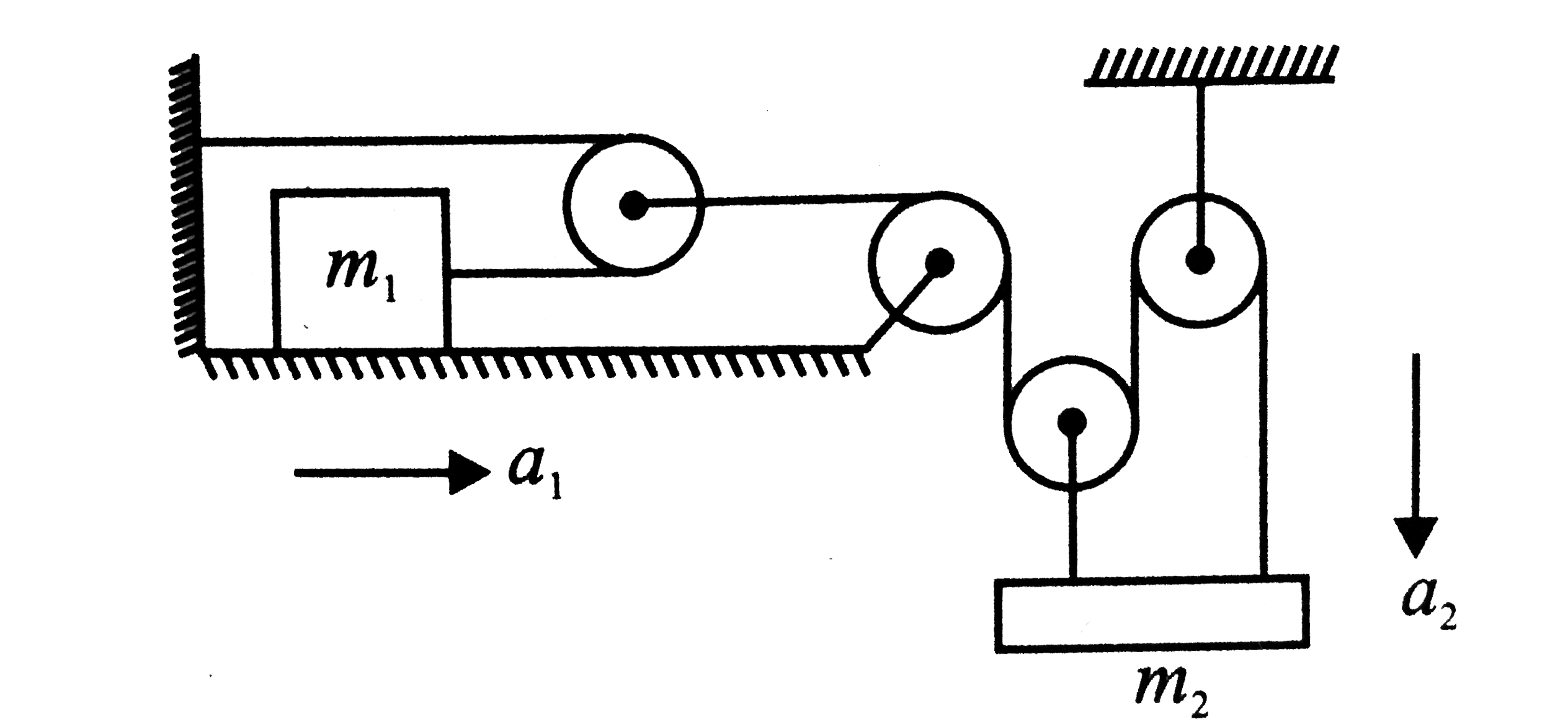
View SolutionIf α and β are roots of the equation x2−3x+1=0 and an=αn+βn−1 then find the value of a5−a1a3−a1
View SolutionFor a 'C'M concentrated solution of a weak electrolyte 'A_(x)B_(y) 'alpha' degree of dissociation) is
View SolutionIf αandβ are the roots of equation x2−3x+1=0andan=αn+βn,n∈N then value of a7+a5a6=
View SolutionThe degree of dissociation of weak electrolyde is inversely proportional to the square root fo concentration. It is called Ostwald's dilution law.
α=√Kac As the tempertaure increases, degree of dissociation will increase.
α1α2=√Ka1Ka2 if concentration is same.
α1α2=√c2c1 if acid is same.
pH of 0.005MHCOOH[Ka=2×10−4] is equal to
View SolutionThe degree of dissociation of weak electrolyde is inversely proportional to the square root fo concentration. It is called Ostwald's dilution law.
α=√Kac As the tempertaure increases, degree of dissociation will increase.
α1α2=√Ka1Ka2 if concentration is same.
α1α2=√c2c1 if acid is same.
0.01MCH3COOH has 4.24% degree of dissociation, the degree of dissociation of 0.1MCH3COOH will be
View Solution
