The work done by all the forces (external and internal) on a system equals the change in
(a)total energy
(b)kinetic energy
(c)potential energy
(d)none of these
The correct Answer is:B
To solve the question, we need to apply the work-energy theorem, which relates the work done by all forces acting on a system to the change in kinetic energy of that system.
1. Understanding the Work-Energy Theorem:
The work-energy theorem states that the total work done by all forces (both external and internal) on a system is equal to the change in kinetic energy of that system.
W=ΔKE
2. Defining Work Done (W):
Work done on a system can be expressed as the difference between the final kinetic energy and the initial kinetic energy.
W=KEfinal−KEinitial
3. Kinetic Energy Formula:
The kinetic energy (KE) of an object is given by the formula:
KE=12mv2
where m is the mass of the object and v is its velocity.
4. Expressing Change in Kinetic Energy:
The change in kinetic energy (ΔKE) can be expressed as:
ΔKE=KEfinal−KEinitial=12mv2final−12mv2initial
5. Conclusion:
According to the work-energy theorem, the work done by all forces on a system equals the change in kinetic energy. Therefore, the correct answer to the question is:
B. Kinetic Energy
The work-energy theorem states that the total work done by all forces (both external and internal) on a system is equal to the change in kinetic energy of that system.
Work done on a system can be expressed as the difference between the final kinetic energy and the initial kinetic energy.
The kinetic energy (KE) of an object is given by the formula:
The change in kinetic energy (ΔKE) can be expressed as:
According to the work-energy theorem, the work done by all forces on a system equals the change in kinetic energy. Therefore, the correct answer to the question is:
Similar Questions
Knowledge Check
The negative of the work done by the conserative internal forces on a system equals the change iln
Atotal energyBkinetic energyCpotential energyDnone of these
S1: If the internal forces within a system are conservative, then the work done by the external forces on the system is equal to the change in mechanical energy of the system.
S2: The potential energy of a particle moving along x− axis in a conservative force field is U=2x2−5x+1 is S.I. units. No other forces are acting on it. It has a stable equalibrium position at one point on x− axis.
S3:Internal forces can perform net work on a rigid body.
S4: Internal forces can perform net work on a non− rigid body.
View SolutionStatement−1: The work done by all forces on a system equals to the change in kinetic energy of that system . This statement is true even if nonconservative forces act on the system.
Statement −2: The total work done by internal forces may be positive.
View SolutionIf no external force acts on a system
View SolutionIf no external force acts on a system
View SolutionSTATEMENT-1: Work done by the net force acting on a particle is equal to the change in its kinetic energy.
STATEMENT-2: Change in potential energy is always equal to the work done by external forces.
STATEMENT-3: Work energy theorem is not applicable, if the work is done by the non-conservative forces
View SolutionIs net work done by all the external force of a system is independent of choice of reference frame.
View SolutionAsseration : Displacement time graph of a particle moving in a straight line is shown in figure. Work done by all the forces between time interval t1 and t2 is definitely zero.
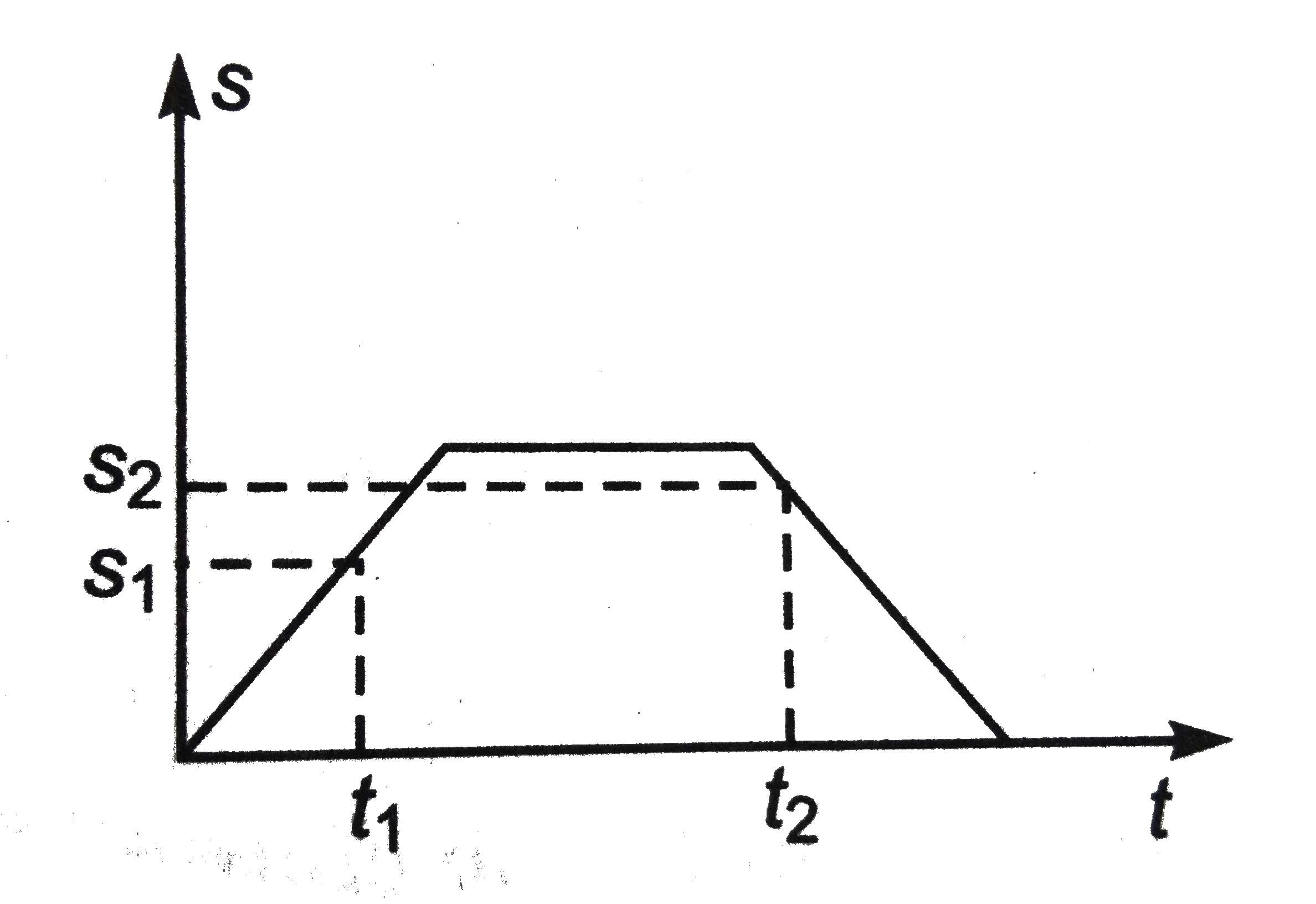 ,
,
Reason : Work done by all the forces is equal to change in kinetic energy.
View Solution
