If the first excitation energy of a hydrogen - like atom is 27.3 eV , then ionization energy of this atom will be
The correct Answer is:36.4
To find the ionization energy of a hydrogen-like atom given that its first excitation energy is 27.3 eV, we can follow these steps:
1. Understanding Excitation Energy: The first excitation energy refers to the energy required to move an electron from the ground state (n=1) to the first excited state (n=2). For hydrogen-like atoms, the energy levels can be described by the formula:
En=−13.6Z2n2 eV
where Z is the atomic number.
2. Calculating the Energy Levels:
- The energy of the ground state (n=1) is:
E1=−13.6Z212=−13.6Z2 eV
- The energy of the first excited state (n=2) is:
E2=−13.6Z222=−13.6Z24 eV
3. Finding the Excitation Energy: The excitation energy from n=1 to n=2 is given by:
Eexcitation=E2−E1=(−13.6Z24)−(−13.6Z2)
Simplifying this gives:
Eexcitation=−13.6Z24+13.6Z2=13.6Z2(1−14)=13.6Z2⋅34
Thus,
Eexcitation=3⋅13.6Z24=10.2Z2 eV
4. Setting Up the Equation: We know from the problem statement that the first excitation energy is 27.3 eV:
10.2Z2=27.3
5. Solving for Z:
Z2=27.310.2=2.676⟹Z=√2.676≈1.64
6. Calculating Ionization Energy: The ionization energy Eionization for hydrogen-like atoms is given by:
Eionization=13.6Z2 eV
Substituting Z2:
Eionization=13.6⋅2.676≈36.4 eV
Final Answer:
The ionization energy of the hydrogen-like atom is 36.4 eV.
En=−13.6Z2n2 eV
where Z is the atomic number.
- The energy of the ground state (n=1) is:
E1=−13.6Z212=−13.6Z2 eV
- The energy of the first excited state (n=2) is:
E2=−13.6Z222=−13.6Z24 eV
Eexcitation=E2−E1=(−13.6Z24)−(−13.6Z2)
Simplifying this gives:
Eexcitation=−13.6Z24+13.6Z2=13.6Z2(1−14)=13.6Z2⋅34
Thus,
Eexcitation=3⋅13.6Z24=10.2Z2 eV
10.2Z2=27.3
Z2=27.310.2=2.676⟹Z=√2.676≈1.64
Eionization=13.6Z2 eV
Substituting Z2:
Eionization=13.6⋅2.676≈36.4 eV
The ionization energy of the hydrogen-like atom is 36.4 eV.
Similar Questions
If first excitation potential of a hydrogen-like atom is V electron volt, then the ionization energy of this atom will be:
View SolutionExcitations energy of hydrogen atom is 13.6eV match the following
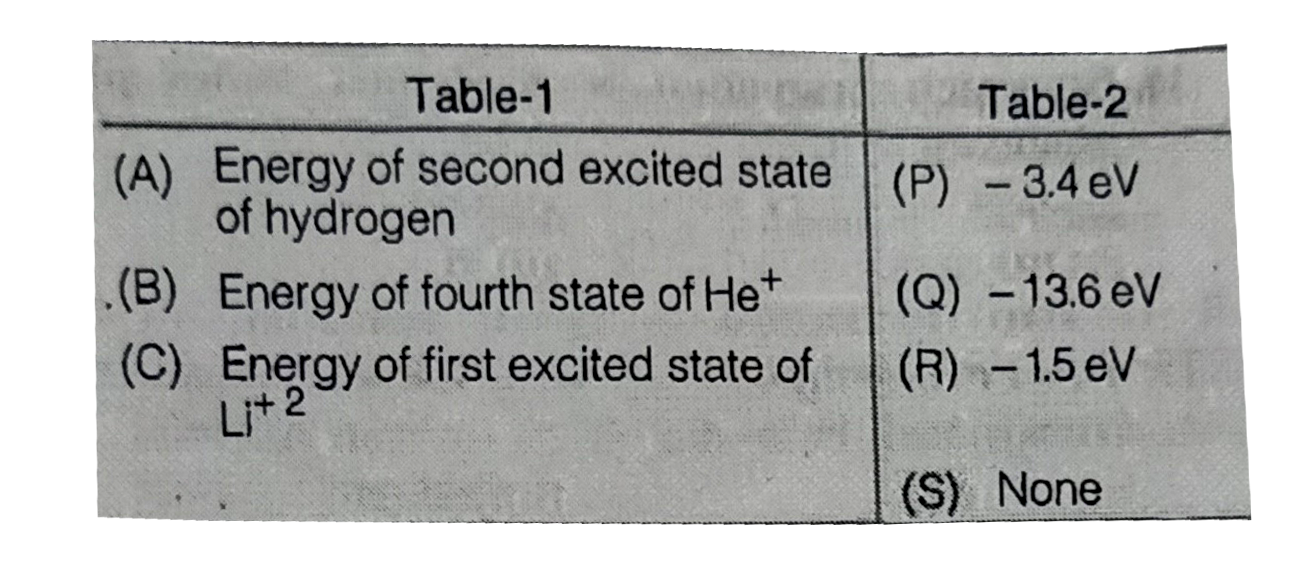 View Solution
View SolutionThe first ionization energy of hydrogen is 2.179×10−18 J
The second ionization energy of helium atom will beView SolutionIf the energy in the first excited state in hydrogen atom is 23.8eV then the potential energy of a hydrogen atom in the ground state can be assumed to be
View SolutionThe ionization energy of a hydrogen atom is εion=13.6eV. Yet the ionization of hydrogen atoms is observed at temperatures for which the average kinetic energy is much less. How can this fact be explained?
View SolutionThe total energy of an electron in the first excited state of the hydrogen atom is about -3.4 eV.
What is the potential energy of the electron in this state ?View SolutionThe first excitation potential of a hypothetical hydrogen- like atom is 15V. Find the third excitation potential of the atom.
View SolutionThe ionization energy of H-atom is 13.6eV. Calculate the is ionization energy of Li+2ion-
View SolutionThe total energy of an electron in the first excited state of hydrogen is about −3.4eV. Its kinetic energy in this state is:
View SolutionA stationary hydrogen atom in the first excited state emits a photon. If the mass of the hydrogen atom is m and its ionization energy is E, then the recoil velocity acquired by the atom is [speed of light = c]
View Solution

