The equilibrium constant of the following isomerisation reaction at 400 K and 298 K are 2.07 and 3.42 respectively. cis− butenek1⇐⇒k−1trans - butene
Which of the following is/are correct?
I. The reaction is exothermic
II. The reaction is endothermic
III. At 400 K 50% of cis - butene and 50% of trans - butene are present of equilibrium
IV. Both at 298 K and 400 K, k1=k−1.
I and IV
II and IV
I and III
I only
The correct Answer is:D
To solve the problem, we need to analyze the given information about the isomerization reaction between cis-butene and trans-butene at two different temperatures (400 K and 298 K) and determine the validity of the statements provided.
Step 1: Understand the Equilibrium Constants
The equilibrium constants (K) for the reaction are given as:
- At 298 K, K1 = 3.42
- At 400 K, K2 = 2.07
Step 2: Determine the Nature of the Reaction
To determine whether the reaction is exothermic or endothermic, we can use the van 't Hoff equation, which relates the change in the equilibrium constant with temperature to the enthalpy change (ΔH) of the reaction:
ln(K2K1)=−ΔHR(1T2−1T1)
Where:
- K1=3.42 (at 298 K)
- K2=2.07 (at 400 K)
- R is the gas constant (approximately 8.314 J/mol·K)
- T1=298 K
- T2=400 K
Step 3: Calculate the Natural Logarithm of the Ratio of Equilibrium Constants
Calculate ln(K2K1):
ln(2.073.42)=ln(0.605)≈−0.494
Step 4: Calculate the Temperature Difference
Calculate 1T2−1T1:
1400−1298≈0.0025−0.003356≈−0.000856
Step 5: Substitute into the van 't Hoff Equation
Substituting into the van 't Hoff equation:
−0.494=−ΔH8.314×(−0.000856)
Rearranging gives:
ΔH=0.494×8.3140.000856≈4,800 J/mol≈4.8 kJ/mol
Since ΔH is positive, the reaction is endothermic.
Step 6: Analyze the Equilibrium Concentrations
At equilibrium, the relationship between the concentrations of cis-butene and trans-butene can be expressed as:
K=(trans-butene)(cis-butene)=2.07 at 400K
Let x be the concentration of trans-butene and A be the initial concentration of cis-butene. Then:
K=xA−x=2.07
Cross-multiplying gives:
2.07(A−x)=x
Rearranging gives:
2.07A−2.07x=x⟹2.07A=3.07x⟹x=2.07A3.07
Calculating the fraction of trans-butene:
xA=2.073.07≈0.674 (or 67.4% trans-butene)
This means that at 400 K, we do not have 50% of each isomer.
Step 7: Evaluate Each Statement
1. The reaction is exothermic: False (it is endothermic).
2. The reaction is endothermic: True.
3. At 400 K, 50% of cis-butene and 50% of trans-butene are present at equilibrium: False (we found 67% trans-butene).
4. Both at 298 K and 400 K, k1=k−1: False (the rate constants are not equal as the equilibrium constants differ).
Conclusion
The correct statement is:
- II. The reaction is endothermic.
The equilibrium constants (K) for the reaction are given as:
- At 298 K, K1 = 3.42
- At 400 K, K2 = 2.07
To determine whether the reaction is exothermic or endothermic, we can use the van 't Hoff equation, which relates the change in the equilibrium constant with temperature to the enthalpy change (ΔH) of the reaction:
- K1=3.42 (at 298 K)
- K2=2.07 (at 400 K)
- R is the gas constant (approximately 8.314 J/mol·K)
- T1=298 K
- T2=400 K
Calculate ln(K2K1):
Calculate 1T2−1T1:
Substituting into the van 't Hoff equation:
At equilibrium, the relationship between the concentrations of cis-butene and trans-butene can be expressed as:
1. The reaction is exothermic: False (it is endothermic).
2. The reaction is endothermic: True.
3. At 400 K, 50% of cis-butene and 50% of trans-butene are present at equilibrium: False (we found 67% trans-butene).
4. Both at 298 K and 400 K, k1=k−1: False (the rate constants are not equal as the equilibrium constants differ).
The correct statement is:
- II. The reaction is endothermic.
Similar Questions
Knowledge Check
Cis-2-butene and trans-2-butene are.
Ageometrical isomersBdiastereomersCenantiomersDposition isomers
The equilibrium constant of the reaction :
Zn(s)+2Ag+(aq)→Zn(aq)+2Ag(s),E∘=1.50V at
298 K is
View SolutionReaction of trans-2-butene with Br2 in presence of CCl4 gives
View SolutionFor the following isomerisation reaction
cis-butene-2⇔trans−butene-2 Kp=1.732
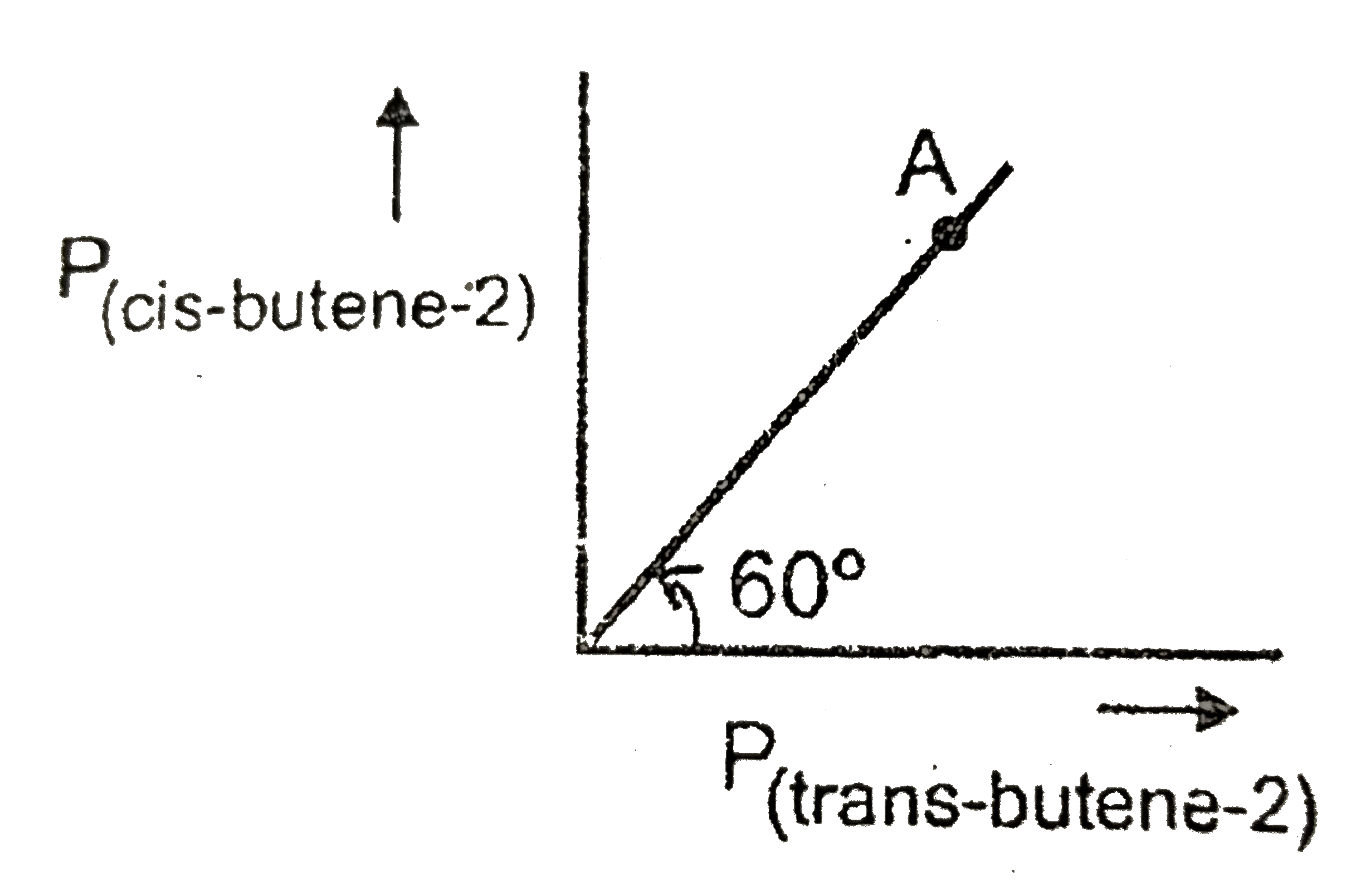
Which of the following statement is true at point A?
View SolutionK2HgI4 is 50% ionised in aqueous solution. Which of the following are correct ?
1. n=7
2. n=3
3. i=2
4. i=4
View SolutionA chemical reaction is spontaneous at 298K but non spontaneous
at 350K . Which one of the following is true
for the reaction ?
View SolutionThe equilibrium constant of a reaction at 298 K is 5×10−3 and at 1000 K is 2×10−5 What is the sign of △H for the reaction.
View SolutionThe addition of Br2 to trans-2-butene produces
View Solution
