Two bodies of same mass tied with an inelastic string of length l lie together. One of them is projected vertically upwards with velocity √6gl. Find the maximum height up to which the centre of mass system of the two masses rises.
3l4
l2
3l2
l
The correct Answer is:D
To solve the problem, we will follow these steps:
Step 1: Understand the Initial Conditions
We have two bodies of the same mass tied together with an inelastic string of length l. One of the bodies is projected vertically upwards with an initial velocity of v0=√6gl.
Step 2: Determine the Velocity of the First Body When the String Becomes Taut
As the first body rises, it will continue to move upward until the string becomes taut. At this point, the second body is still at rest. We can use the kinematic equation to find the velocity of the first body when the string becomes taut.
Using the equation:
v2=u2+2as
where:
- v = final velocity (when the string becomes taut)
- u=√6gl = initial velocity
- a=−g (acceleration due to gravity, acting downwards)
- s=l (the distance the first body rises until the string becomes taut)
Substituting the values:
v2=(√6gl)2−2g(l)
v2=6gl−2gl=4gl
v=√4gl=2√gl
Step 3: Apply Conservation of Momentum
When the string becomes taut, both bodies will start moving together with a common velocity v2. By conservation of momentum:
mv1=(m+m)v2
where v1=2√gl (velocity of the first body just before the string becomes taut).
Substituting the values:
m(2√gl)=2mv2
2√gl=2v2
v2=√gl
Step 4: Find the Maximum Height of the Center of Mass
Now that we have the common velocity v2=√gl, we can find the maximum height the center of mass rises. The center of mass of the two bodies is initially at a height of l2 (since both bodies are at the same height).
Using the kinematic equation again to find the height h the center of mass rises from its initial position:
v2=u2+2as
where:
- v=0 (final velocity at the maximum height)
- u=√gl (initial velocity of the center of mass)
- a=−g (acceleration due to gravity)
- s=h (height the center of mass rises)
Substituting the values:
0=(√gl)2−2gh
0=gl−2gh
2gh=gl
h=l2
Step 5: Calculate the Total Height of the Center of Mass
The total height Hmax of the center of mass from the ground is:
Hmax=l2+l2=l
Final Answer
The maximum height up to which the center of mass system of the two masses rises is l.
---
We have two bodies of the same mass tied together with an inelastic string of length l. One of the bodies is projected vertically upwards with an initial velocity of v0=√6gl.
As the first body rises, it will continue to move upward until the string becomes taut. At this point, the second body is still at rest. We can use the kinematic equation to find the velocity of the first body when the string becomes taut.
v2=u2+2as
where:
- v = final velocity (when the string becomes taut)
- u=√6gl = initial velocity
- a=−g (acceleration due to gravity, acting downwards)
- s=l (the distance the first body rises until the string becomes taut)
v2=(√6gl)2−2g(l)
v2=6gl−2gl=4gl
v=√4gl=2√gl
When the string becomes taut, both bodies will start moving together with a common velocity v2. By conservation of momentum:
mv1=(m+m)v2
where v1=2√gl (velocity of the first body just before the string becomes taut).
m(2√gl)=2mv2
2√gl=2v2
v2=√gl
Now that we have the common velocity v2=√gl, we can find the maximum height the center of mass rises. The center of mass of the two bodies is initially at a height of l2 (since both bodies are at the same height).
v2=u2+2as
where:
- v=0 (final velocity at the maximum height)
- u=√gl (initial velocity of the center of mass)
- a=−g (acceleration due to gravity)
- s=h (height the center of mass rises)
0=(√gl)2−2gh
0=gl−2gh
2gh=gl
h=l2
The total height Hmax of the center of mass from the ground is:
Hmax=l2+l2=l
The maximum height up to which the center of mass system of the two masses rises is l.
Similar Questions
Two particles A and B of equal masses lie close together on a horizontal table and are connected by a light inextensible string of length l. A is projected vertically upwards with a velocity √10gl. Find the velocity with which it reaches the table again.
View SolutionIf two particles of masses m1 and m2 are projected vertically upwards with speed v1 and v2, then the acceleration of the centre of mass of the system is
View SolutionTwo bodies are thrown vertically upwards with their initial velocity in the ratio 2:3. Then the ratio of the maximum height attained by them is
View SolutionTwo particles of equal mass are projected simultaneously with speeds 20m/s end 10√3m/s as shown in figure. Find the maximum height reached by the centre of mass of the particles
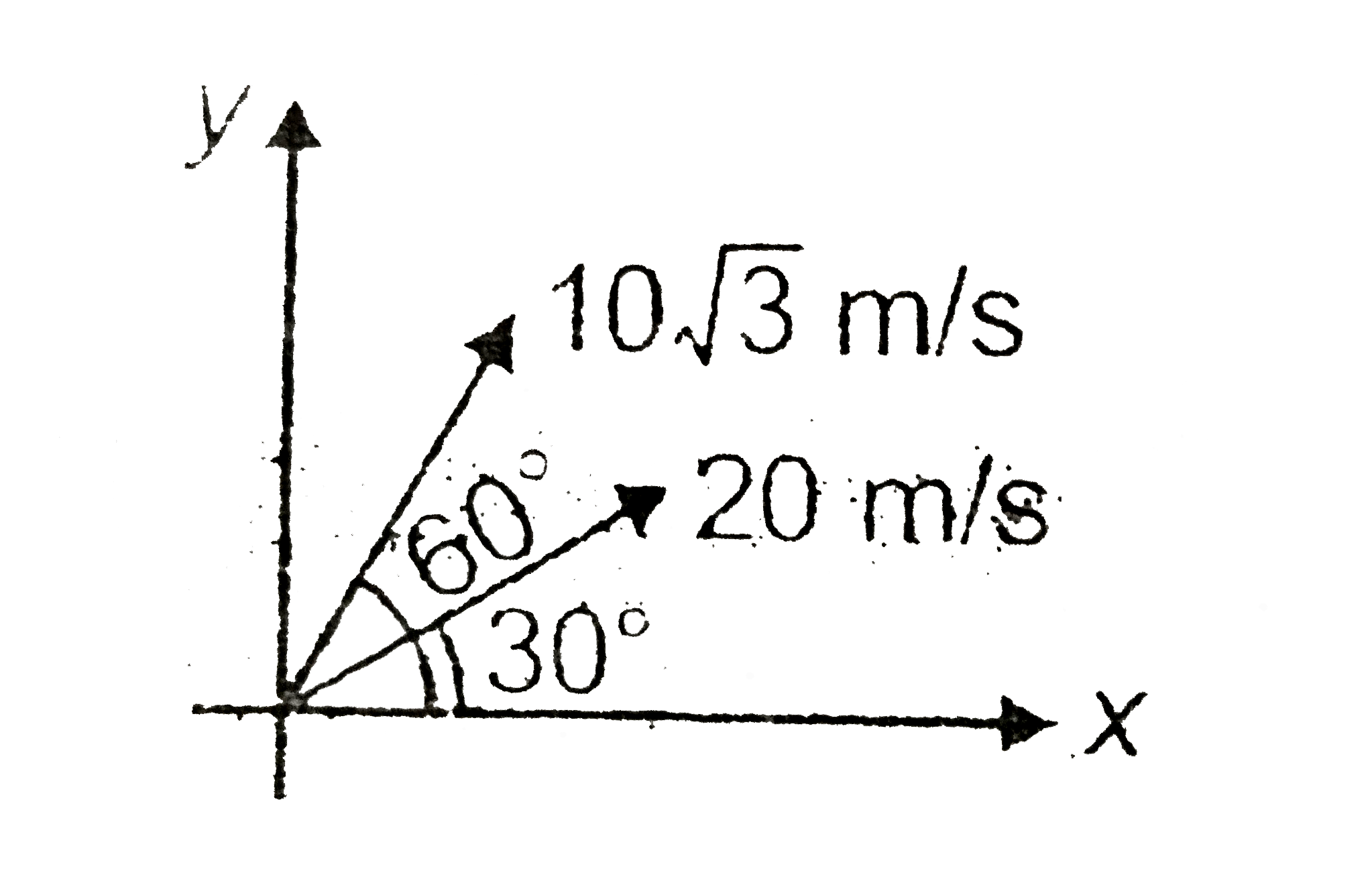
View SolutionA particle is suspended by a light vertical inelastic string of length 1 from a fixed support. At its equilbrium position it is projected horizontally with a speed √6gl. Find the ratio of the tension in the string in its horizontal position to that in the string when the particle is vertically above the point of support.
View SolutionA body is projected vertically upwards with a speed of √GMR ( M is mass and R is radius of earth ) . The body will attain a height of
View SolutionWith what velocity a body of mass m be projected vertically upwards so that it may be able to reach a height nR above earth's surface
View SolutionTwo particles of a mass 2m and m are tied with an inextensible string the particle of mass m is given a speed V as shown in the figure. Find the speed with which the particle of mass 2m starts moving.

View SolutionTwo particles of a mass 2m and m are tied with an inextensible string the particle of mass m is given a speed V as shown in the figure. Find the speed with which the particle of mass 2m starts moving.

View SolutionA particle in projected with velocity √3gL at point A (lowest point of the circle) in the vertical plane. Find the maximum height about horizontal level of point A if the string slacks at the point B as shown in figure.

View Solution
