In a Young's double slit experiment, the slit separation is and the screen is from the slit. For a monochromatic light of wavelength , the distance of 3rd minima from the central maxima is
0.50 mm
1.25 mm
1.50 mm
1.75 mm
The correct Answer is:B
To find the distance of the 3rd minima from the central maxima in a Young's double slit experiment, we can use the formula for the position of minima, which is given by:
Xn=(2n−1)λD2d
where:
- Xn = distance of the nth minima from the central maxima
- n = order of the minima (for the 3rd minima, n=3)
- λ = wavelength of the light
- D = distance from the slits to the screen
- d = slit separation
Given Data:
- Slit separation, d=1 mm=1×10−3 m
- Distance to the screen, D=1 m
- Wavelength, λ=500 nm=500×10−9 m
Step 1: Substitute the values into the formula
We need to find X3 for the 3rd minima.
X3=(2⋅3−1)⋅λ⋅D2d
Step 2: Calculate (2⋅3−1)
(2⋅3−1)=6−1=5
Step 3: Substitute the values into the formula
Now substituting all the values into the equation:
X3=5⋅(500×10−9)⋅12⋅(1×10−3)
Step 4: Simplify the expression
Calculating the denominator:
2⋅(1×10−3)=2×10−3
Now substituting back:
X3=5⋅500×10−92×10−3
Step 5: Calculate the numerator
5⋅500=2500
Thus,
X3=2500×10−92×10−3
Step 6: Divide the values
X3=25002×10−910−3=1250×10−6 m
Step 7: Convert to millimeters
X3=1.25×10−3 m=1.25 mm
Final Answer
The distance of the 3rd minima from the central maxima is 1.25 mm.
- Xn = distance of the nth minima from the central maxima
- n = order of the minima (for the 3rd minima, n=3)
- λ = wavelength of the light
- D = distance from the slits to the screen
- d = slit separation
- Slit separation, d=1 mm=1×10−3 m
- Distance to the screen, D=1 m
- Wavelength, λ=500 nm=500×10−9 m
We need to find X3 for the 3rd minima.
(2⋅3−1)=6−1=5
Now substituting all the values into the equation:
Calculating the denominator:
5⋅500=2500
Thus,
X3=25002×10−910−3=1250×10−6 m
X3=1.25×10−3 m=1.25 mm
The distance of the 3rd minima from the central maxima is 1.25 mm.
Similar Questions
In Young's double-slit experiment, the slit separation is 0.5 mm and the screen is 0.5 m away from the slit. For a monochromatic light of wavelength 500 nm, the distance of 3rd maxima from the 2nd minima on the other side of central maxima is
View SolutionIn a double slit experiment, the two slits are 1mm apart and the screen is placed 1m away. A monochromatic light of wavelength 500nm is used. What will be the width of each slit for obtaining ten maxima of double slit within the central maxima of single-slit pattern?
View Solution
Knowledge Check
A beam of light consisting of two wavelengths 650nm and 520nm is used to obtain interference fringes in a Young's double slit experiment.
(a) Find the distance of the third bright fringe on the screen from the central maximum for the wavelength 650nm.
(b) What is the least distance from the central maximum where the bright fringes due to both the wavelengths coincide? The distance between the slits is 2mm and the distance between the plane of the slits and screen is 120cm.A1.17 mmB2.52 mmC1.56 mmD3.14 mmIn a Young's double slit esperiment, the angular width of a fringe formed on a distant screen is 1∘ . The slit separation is 0.01 mm. The wavelength of the light is
A0.174 nmB0.174 Ã…C0.174 μmD0.174×10−4m
In Yonung's double-slit experiment, two slits which are separated by 1.2 mm are illuminated with a monochromatic light of wavelength 6000Å The interference pattern is observed on a screen placed at a distance of 1 m from the slits. Find the number of bright fringes formed over 1 cm width on the screen.
View SolutionIn a YDSE, distance between the slits and the screen is 1m, separation between the slits is 1mm and the wavelength of the light used is 5000nm. The distance of 100th maxima from the central maxima is:
View SolutionIn a Young's double slit experiment , the slits are Kept 2mm apart and the screen is positioned 140cm away from the plane of the slits. The slits are illuminatedd with light of wavelength 600nm. Find the distance of the third brite fringe. From the central maximum . in the interface pattern obtained on the screen frings from the central maximum. 3
View SolutionIn the youngs double slit experiment ,the slit sepration is 1mm ,the distance between source and screen is 1m ,wavelength of light used is 6500 angstrom.Find the distance between fifth maxima and third minima in (mm)?
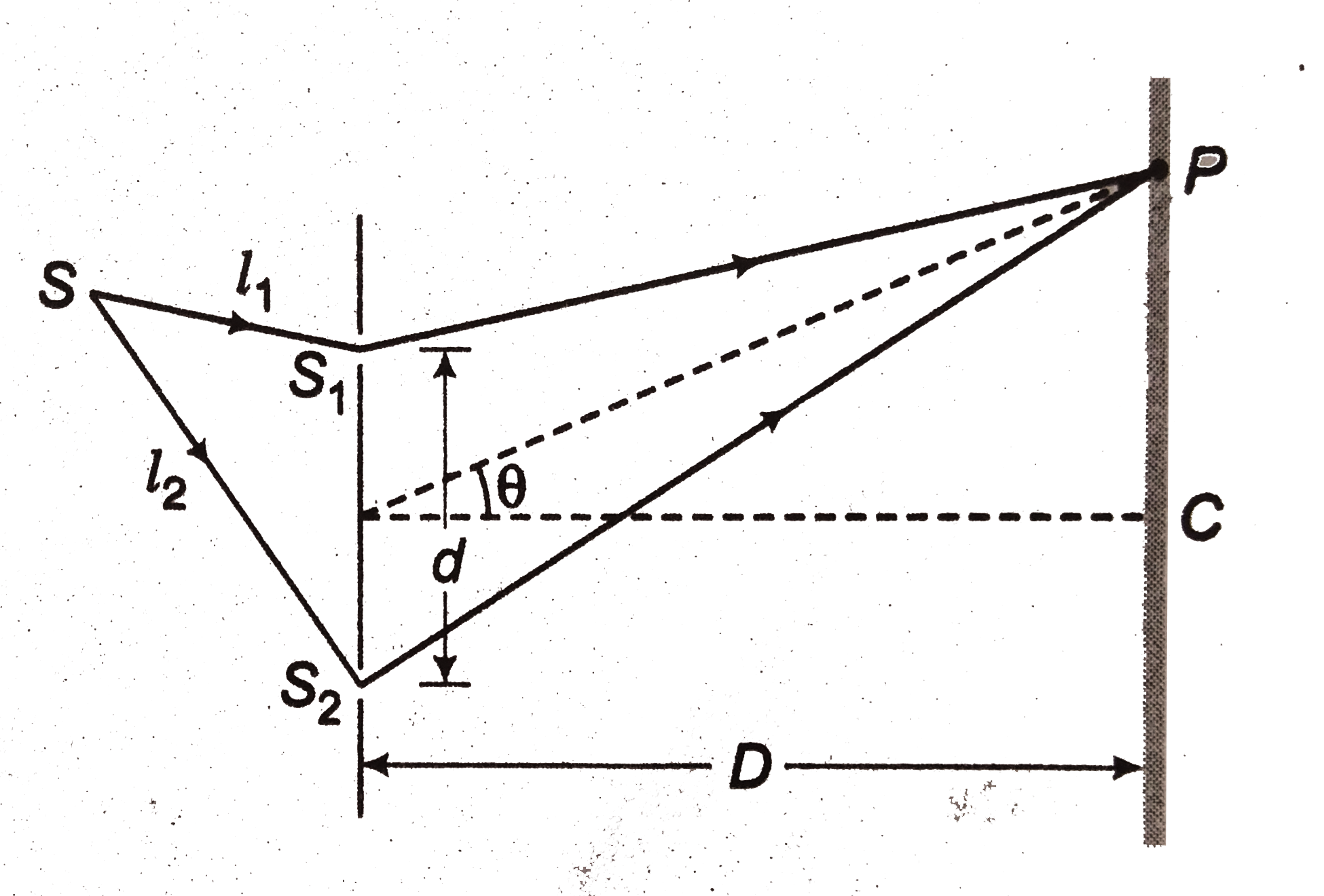
View SolutionIn a Young's double slit experiment, the light sources is at distance l1=20μm and l2=40μm form the main slit. The light of wavelength lambda = 500nm is incident on slits separated at a distance 10μm. A screen is placed at a distance D = 2 away from the slits as shown in figure. Find
(a) the values of θ relative to the central line where maxima appear on the screen?
(b) how many maxima will appear on the screen?
what should be the minimum thickness of a slab of refractive index 1.5 placed on the path of one of the ray so that minima occurs at C ?
 .
.
View SolutionIn Young's double slit experiment , the two slits 0.20 mm apart are illuminated by monochromatic light of wavelength 600 nm . The screen 1.0 m away from the slits .
(a) Find the distance of the second (i) bright fringe , (ii) dark fringe from the central maximum .
(b) How will the fringe pattern change if the screen is moved away from the slits ?
View Solution
