Two points on a travelling wave having frequency 500 Hz and velocity 300ms−1 are 60∘ out of phase, then the minimum distance between the two point is
(a)0.2
(b)0.1
(c)0.5
(d)0.4
The correct Answer is:B
To solve the problem, we need to find the minimum distance between two points on a traveling wave that are 60 degrees out of phase. We are given the frequency and velocity of the wave.
1. Identify Given Values:
- Frequency (f) = 500 Hz
- Velocity (v) = 300 m/s
- Phase difference (Δφ) = 60 degrees
2. Convert Phase Difference to Radians:
- Since calculations in physics are often easier in radians, we convert degrees to radians:
Δϕ=60∘=60×π180=π3 radians
3. Calculate Wavelength (λ):
- The wavelength can be calculated using the formula:
λ=vf
- Substituting the values:
λ=300m/s500Hz=300500=0.6m
4. Relate Phase Difference to Path Difference (Δx):
- The relationship between phase difference and path difference is given by:
Δϕ=2πλΔx
- Rearranging for Δx gives:
Δx=Δϕ⋅λ2π
5. Substituting Values:
- Now substitute Δφ and λ into the equation:
Δx=(π3)⋅(0.6)2π
- Simplifying this:
Δx=0.66=0.1m
6. Conclusion:
- The minimum distance between the two points that are 60 degrees out of phase is:
Δx=0.1m
Final Answer:
The minimum distance between the two points is 0.1 meters.
- Frequency (f) = 500 Hz
- Velocity (v) = 300 m/s
- Phase difference (Δφ) = 60 degrees
- Since calculations in physics are often easier in radians, we convert degrees to radians:
Δϕ=60∘=60×π180=π3 radians
- The wavelength can be calculated using the formula:
λ=vf
- Substituting the values:
λ=300m/s500Hz=300500=0.6m
- The relationship between phase difference and path difference is given by:
Δϕ=2πλΔx
- Rearranging for Δx gives:
Δx=Δϕ⋅λ2π
- Now substitute Δφ and λ into the equation:
Δx=(π3)⋅(0.6)2π
- Simplifying this:
Δx=0.66=0.1m
- The minimum distance between the two points that are 60 degrees out of phase is:
Δx=0.1m
The minimum distance between the two points is 0.1 meters.
Similar Questions
A wave of frequency 500 Hz has velocity 360 m/sec. The distance between two nearest points 60∘ out of phase, is
View SolutionConsider a wave with frequency 300 Hz and speed 350ms−1. How far apart are two points 60° out of phase?
View SolutionIf a sound wave of frequency 500 Hz and velocity 350 m/s. Then the distance between the two particles of a phase difference of 60∘ will be nearly
View SolutionThe phase velocity of a wave of frequency 500 Hz is 400 m/s (i) How far apart are two points 61∘ out of phase? (ii) Calculate the phase difference between two displacements atá certain point at times 10−3 apart
View SolutionThe frequency of a transverse wave is 800Hz and its velocity is 200m/s. (i) How far apart are two points 30∘ out of phase? (ii) What is the phase difference between two displacements at a certain point at times 10−3 s apart.
View SolutionTwo point sound sources A and B each to power 25πw and frequency 850Hz are 1m apart. The sources are in phase
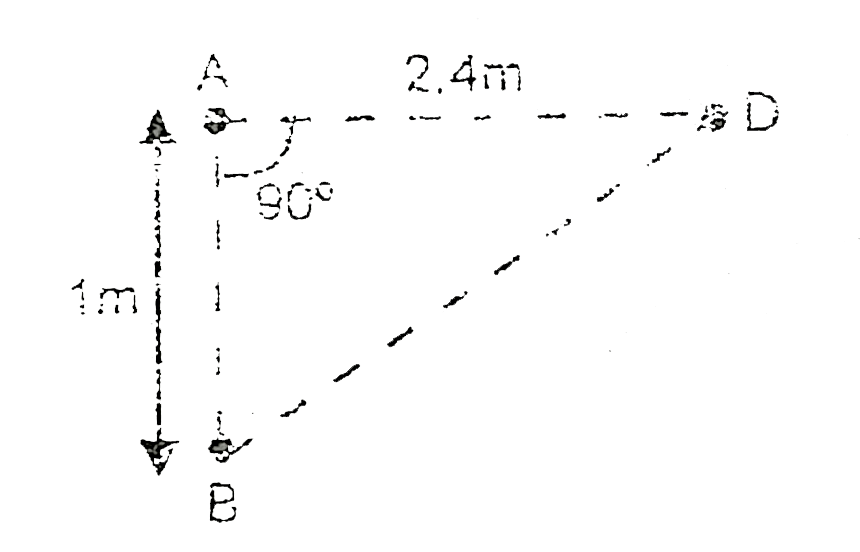
Determine the phase difference between the waves emitting from A and B received by detector D as shown in figure. Velocity of sound =340m/s.
View SolutionThere are two waves having wavelength 100 cm and 101 cm and same velocity 303ms−1. The beat frequency is
View SolutionA progressive wave of frequency 550 Hz is travelling with a velocity of 360 ms How far apart are the two points 60∘ out of phase ?
View SolutionA sinusoidal wave travels along a taut string of linear mass density 0.1g/cm. The particles oscillate along y− direction and wave moves in the positive x− direction . The amplitude and frequency of oscillation are 2mm and 50Hz respectively. The minimum distance between two particles oscillating in the same phase is4m.
The amount of energy transferred ( in Joules ) through any point of the string in 5 seconds is
View SolutionWhat is the minimum distance between two points in a wave having a phase difference 2π ?
View Solution
