Two particles P1 and P2 are performing SHM along the same line about the same mean position , initial they are at their extreme position. If the time period of each particle is 12 sec and the difference of their amplitude is 12cm then find the minimum time after which the separation between the particle becomes 6cm.
The correct Answer is:2
To solve the problem, we will follow these steps:
Step 1: Understand the setup
We have two particles P1 and P2 performing Simple Harmonic Motion (SHM) along the same line about the same mean position. Both particles start at their extreme positions, and the difference in their amplitudes is given as 12cm.
Step 2: Define the parameters
Let:
- A1 = Amplitude of particle P1
- A2 = Amplitude of particle P2
- Given that A2−A1=12cm
- Time period T=12s
The angular frequency ω can be calculated as:
ω=2πT=2π12=π6rad/s
Step 3: Write the equations of motion
The equations of motion for the two particles can be written as:
- For particle P1:
x1=A1cos(ωt)
- For particle P2:
x2=A2cos(ωt)
Step 4: Calculate the separation
The separation S between the two particles is given by:
S=|x2−x1|=|A2cos(ωt)−A1cos(ωt)|=|(A2−A1)cos(ωt)|
Substituting A2−A1=12cm:
S=|12cos(ωt)|
Step 5: Set the separation equal to 6 cm
We need to find the time when the separation is 6cm:
|12cos(ωt)|=6
This simplifies to:
cos(ωt)=612=12
Step 6: Solve for ωt
The cosine function gives us:
cos(ωt)=12⟹ωt=π3 or ωt=5π3 (but we need the first occurrence)
Step 7: Substitute ω and solve for t
Substituting ω=π6:
π6t=π3
Solving for t:
t=π/3π/6=13×6=2s
Conclusion
The minimum time after which the separation between the particles becomes 6cm is 2s.
---
We have two particles P1 and P2 performing Simple Harmonic Motion (SHM) along the same line about the same mean position. Both particles start at their extreme positions, and the difference in their amplitudes is given as 12cm.
Let:
- A1 = Amplitude of particle P1
- A2 = Amplitude of particle P2
- Given that A2−A1=12cm
- Time period T=12s
ω=2πT=2π12=π6rad/s
The equations of motion for the two particles can be written as:
- For particle P1:
x1=A1cos(ωt)
- For particle P2:
x2=A2cos(ωt)
The separation S between the two particles is given by:
S=|x2−x1|=|A2cos(ωt)−A1cos(ωt)|=|(A2−A1)cos(ωt)|
Substituting A2−A1=12cm:
S=|12cos(ωt)|
We need to find the time when the separation is 6cm:
|12cos(ωt)|=6
This simplifies to:
cos(ωt)=612=12
The cosine function gives us:
cos(ωt)=12⟹ωt=π3 or ωt=5π3 (but we need the first occurrence)
Substituting ω=π6:
π6t=π3
Solving for t:
t=π/3π/6=13×6=2s
The minimum time after which the separation between the particles becomes 6cm is 2s.
Similar Questions
The amplitude of a executing SHM is 4cm At the mean position the speed of the particle is 16cm/s The distance of the particle from the mean position at which the speed the particle becomes 8√3cm/s will be
View SolutionA particle performing SHM with amplitude 10cm. At What distance from mean position the kinetic energy of the particle is thrice of its potential energy ?
View SolutionTwo particle A and B execute SHM along the same line with the same amplitude a, same frequency and same equlibrium position O. If the phase difference between them is ϕ=2sin−1(0.9) then find maxium distance between two.
View SolutionTwo particles are separated at a horizontal distance x as shown in (Fig. 5.57). They are projected at the same time as shown in the figure with different initial speed. Find the time after which the horizontal distance between the particles becomes zero.
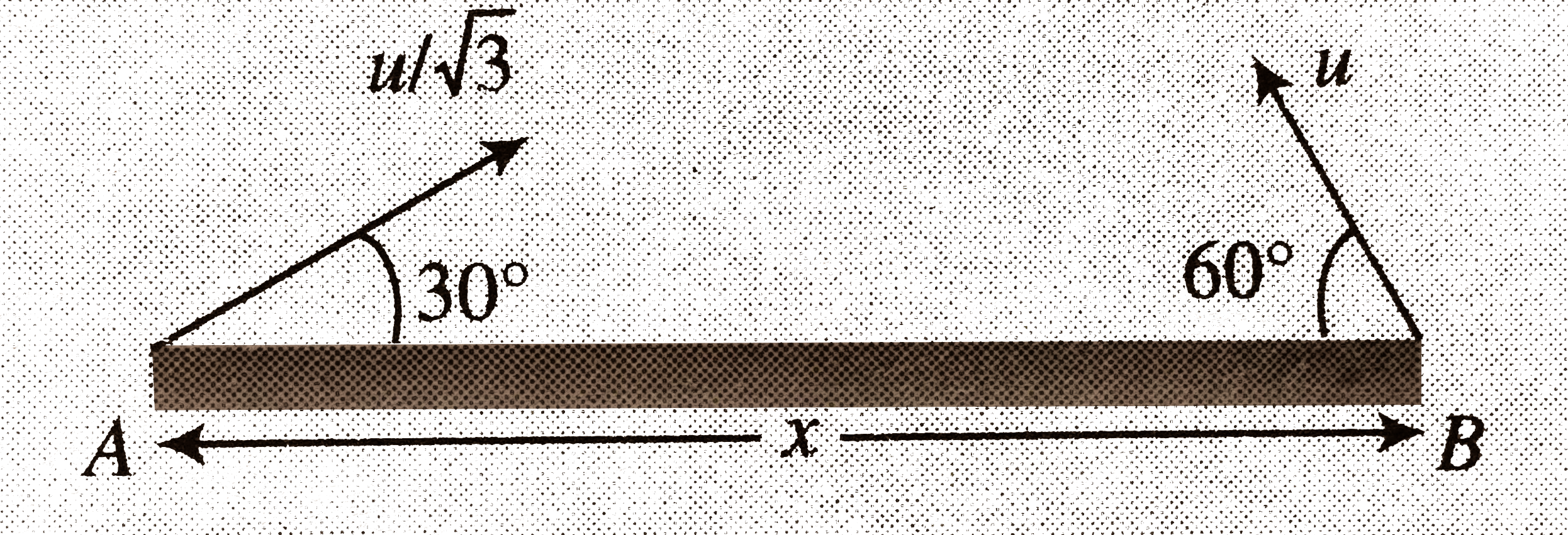 .
.
View SolutionThe time period of a particle executing SHM is T. After a time T/6 after it passes its mean position, then att=0 its :
View SolutionThe kinetic energy of a particle executing shm is 32 J when it passes through the mean position. If the mass of the particle is.4 kg and the amplitude is one metre, find its time period.
View SolutionA particle executing SHM of amplitude 4 cm and T=4s .The time taken by it to move from positive extreme position to half the amplitude is
View SolutionTwo particles are in SHM with the same amplitude and frequency along the same line and about the same point. If the maximum separation between them is √3 times their amplitude, the phase difference between them is 2πn. Find value of n
View SolutionA particle starts from a point P at a distance of A/2 from the mean position O and travels towards left as shown in the figure. If the time period of SHM, executed about O is T and amplitude A then the equation of the motion of particle is

View SolutionTwo particles A and B oscillates in SHM having same amplitude and frequencies fA and fB respectively. At t=0 the particle A is at positive extreme position while the particle B is at a distance half of the amplitude on the positive side of the mean position and is moving away from the mean position.
View Solution
