An object of mass 8 kg starts to move from rest under the action of a variable force F=3xN where x is the distance (in m) covered by the object. If initially, the position of the object is x = 2 m, then find its speed (in m s−1) when it crosses x = 10 m.
The correct Answer is:6
To solve the problem step-by-step, we will follow these steps:
Step 1: Identify the given information
- Mass of the object, m=8kg
- Force acting on the object, F=3xN
- Initial position, xi=2m
- Final position, xf=10m
- Initial velocity, vi=0m/s
Step 2: Relate force to acceleration
From Newton's second law, we know that:
F=ma
Thus, we can express acceleration a as:
a=Fm=3x8
Step 3: Use the relationship between acceleration, velocity, and position
Using the relationship:
a=vdvdx
we can substitute for a:
3x8=vdvdx
Step 4: Rearranging and integrating
Rearranging gives:
vdv=3x8dx
Now, we will integrate both sides. The left side will be integrated from 0 to v and the right side from 2 to 10:
∫v0vdv=∫1023x8dx
Step 5: Perform the integration
The left side integrates to:
v22∣∣∣v0=v22
The right side integrates as follows:
38∫102xdx=38(x22)102=38(1022−222)=38(1002−42)=38(50−2)=38×48=18
Step 6: Set the integrals equal to each other
Now we have:
v22=18
Multiplying both sides by 2 gives:
v2=36
Step 7: Solve for v
Taking the square root:
v=6m/s
Final Answer
The speed of the object when it crosses x=10m is:
6m/s
- Mass of the object, m=8kg
- Force acting on the object, F=3xN
- Initial position, xi=2m
- Final position, xf=10m
- Initial velocity, vi=0m/s
From Newton's second law, we know that:
F=ma
Thus, we can express acceleration a as:
a=Fm=3x8
Using the relationship:
a=vdvdx
we can substitute for a:
3x8=vdvdx
Rearranging gives:
vdv=3x8dx
Now, we will integrate both sides. The left side will be integrated from 0 to v and the right side from 2 to 10:
∫v0vdv=∫1023x8dx
The left side integrates to:
v22∣∣∣v0=v22
The right side integrates as follows:
38∫102xdx=38(x22)102=38(1022−222)=38(1002−42)=38(50−2)=38×48=18
Now we have:
v22=18
Multiplying both sides by 2 gives:
v2=36
Taking the square root:
v=6m/s
The speed of the object when it crosses x=10m is:
6m/s
Similar Questions
An object starts from rest and is acted upon by a variable force F as shown in figure. If F0 is the initial value of the force, then the position of the object, where it again comes of rest will be :-
View SolutionAn object of mass 2 kg at rest at origin starts moving under the action of a force
→F=(3t2ˆi+4tˆj)N The velocity of the object at t = 2 s will be -
View Solution
Knowledge Check
A force F acting on an object varies with distance x as shown in the figure. The work done by the force in moving the object from x=0 to x = 20 m is
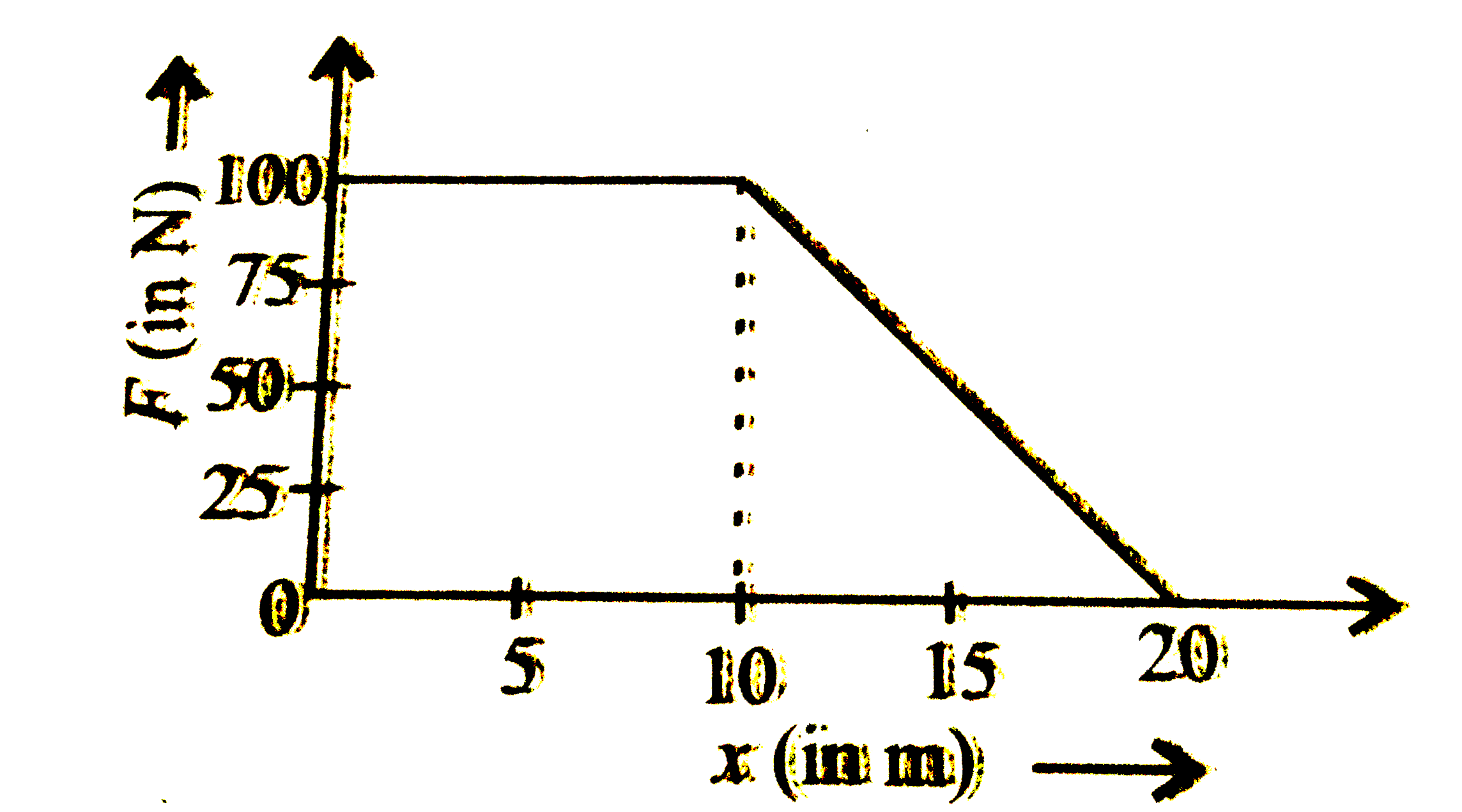 A500 JB1000 JC1500 JD2000 J
A500 JB1000 JC1500 JD2000 J
A ball of mass is thrown vertically upwards by applying a force by hand. If the hand moves while applying the force and the ball goes up to height further, find the magnitude of the force. (Take g=10ms−2)
View SolutionAn object of mass m, initially at rest under the action of a constant force F attains a velocity v in time t. Then, the average power supplied to mass is
View SolutionA body starts from rest with a uniform acceleration of 2 m s−1 . Find the distance covered by the body in 2 s.
View SolutionAn object of mass m moves with constant speed in a circular path of radius r under the action of a force of constant magnitude F. the kinetic energy of object is
View SolutionA particle of mass 2kg starts to move at position x=0 and time t=0 under the action of force F=(10+4x)N along the x− axis on a frictionless horizontal track . Find the power delivered by the force in watts at the instant the particle has moved by distance 5m.
View SolutionAn object of mass 5 kg falls from rest through a vertical distance of 20m and attains a velocity of 10 m/s. How much work is done by the resistance of the air on the object?
(g=10m/s2).
View SolutionAn object of mass 5 kg falls from rest through a vertical distance of 20m and attains a velocity of 10 m/s. How much work is done by the resistance of the air on the object?
(g=10m/s2).
View Solution
