Interference fringes are produced on a screen by using two light sources of intensities / and 9/. The phase difference between the beams π2 is at point P and πat point Q on the screen. The difference between the resultant intensities at point P and Q is
(a)2I
(b)4I
(c)6I
(d)8I
The correct Answer is:C
To solve the problem of finding the difference between the resultant intensities at points P and Q, we can follow these steps:
Step 1: Identify the given values
We have two light sources with intensities:
- I1=I
- I2=9I
The phase differences at points P and Q are:
- At point P: ϕP=π2
- At point Q: ϕQ=π
Step 2: Calculate the resultant intensity at point P
The formula for the resultant intensity IP when two waves interfere is given by:
IP=I1+I2+2√I1I2cos(ϕP)
Substituting the values:
IP=I+9I+2√I⋅9Icos(π2)
Since cos(π2)=0:
IP=I+9I+0=10I
Step 3: Calculate the resultant intensity at point Q
Using the same formula for point Q:
IQ=I1+I2+2√I1I2cos(ϕQ)
Substituting the values:
IQ=I+9I+2√I⋅9Icos(π)
Since cos(π)=−1:
IQ=I+9I+2√I⋅9I(−1)
=10I−2√9I2
=10I−6I=4I
Step 4: Find the difference between the resultant intensities
Now, we calculate the difference between the intensities at points P and Q:
ΔI=IP−IQ=10I−4I=6I
Final Answer
The difference between the resultant intensities at point P and Q is:
ΔI=6I
We have two light sources with intensities:
- I1=I
- I2=9I
- At point P: ϕP=π2
- At point Q: ϕQ=π
The formula for the resultant intensity IP when two waves interfere is given by:
IP=I1+I2+2√I1I2cos(ϕP)
IP=I+9I+2√I⋅9Icos(π2)
IP=I+9I+0=10I
Using the same formula for point Q:
IQ=I1+I2+2√I1I2cos(ϕQ)
IQ=I+9I+2√I⋅9Icos(π)
IQ=I+9I+2√I⋅9I(−1)
=10I−2√9I2
=10I−6I=4I
Now, we calculate the difference between the intensities at points P and Q:
ΔI=IP−IQ=10I−4I=6I
The difference between the resultant intensities at point P and Q is:
ΔI=6I
Similar Questions
Two beams of ligth having intensities I and 4I interface to produce a fringe pattern on a screen. The phase difference between the beams is π2 at point A and π at point B. Then the difference between the resultant intensities at A and B is
View SolutionTwo beams of light having intensities I and 4I interferer to produce a fringe pattern on a screen .the phase difference between the beam is π/2 at a point A and 2π at a point B.Then find out the difference between the resultant intensitites at A and B.
View Solution
Knowledge Check
Two beam of light having intensities I and 4I interfere to produce a fringe pattern on a screen. The phase difference between the beams is π2 at point A and π at point B. Then the difference between resultant intensities at A and B is : (2001,2M)
A2IB4IC5ID7I
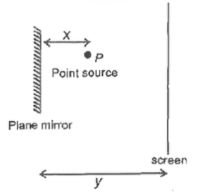
Shape of interference fringes formed on the screen due to point source P, in the case shown here
View SolutionTwo light sources with intensity I0 each interfere in a medium where phase difference between them us π2. Resultant intensity at the point would be.
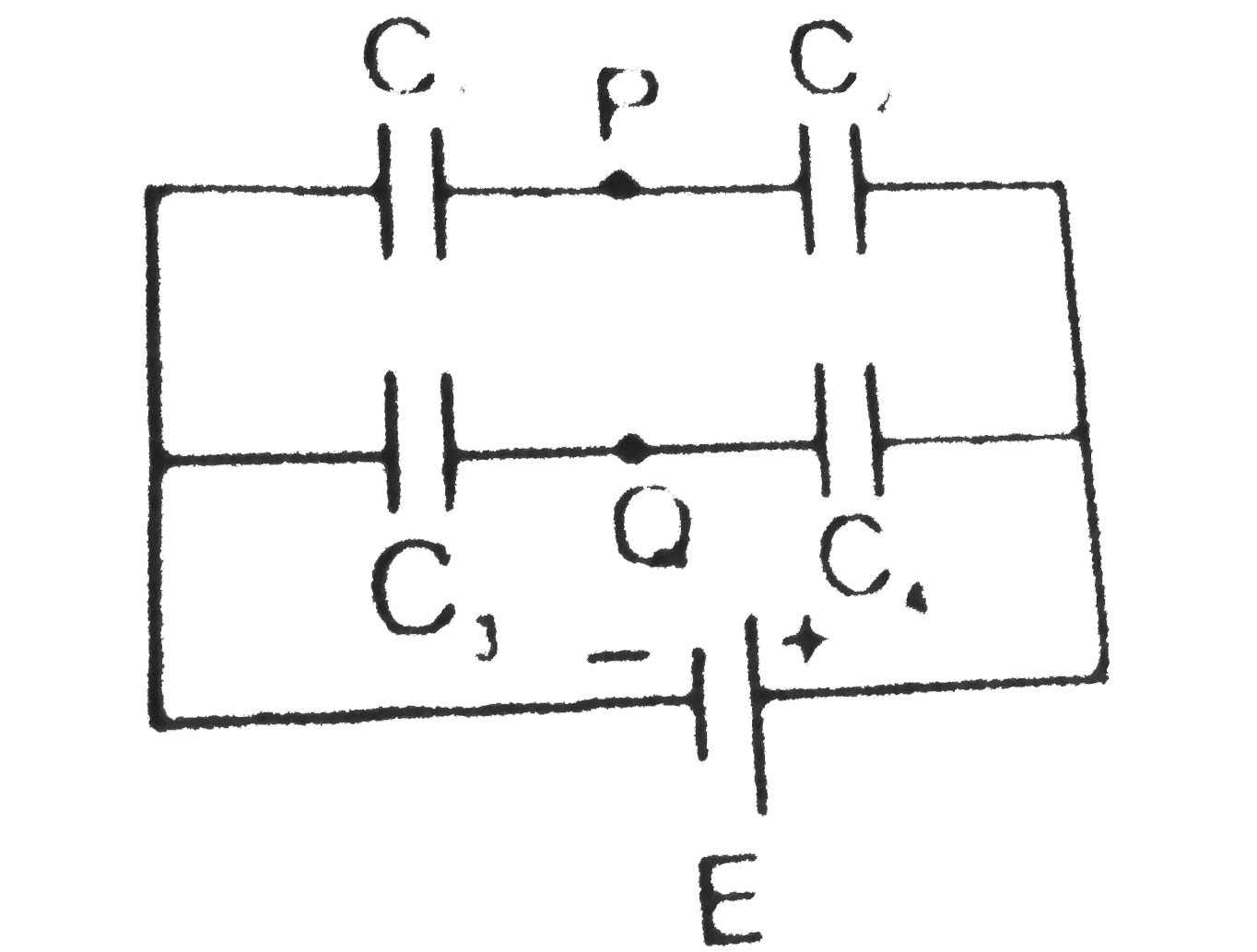
View SolutionThe potential difference between the points P and Q in the adjoining circuit will be-
View SolutionTwo waves having intensity Iand9I produce interference . If the resultant intensity at a point is 7I, what is the phase difference between the two waves ?
View SolutionWhen two waves of intensities l1andl2 coming from coherent sources interfere at a point P, where phase difference is ϕ, then resultant intensity (lres) at point P would be
View SolutionTwo coherent light beams of intensities I and 4I produce interference pattern. The intensity at a point where the phase difference is zero, will b:
View SolutionTwo coherent sources each emitting light of intensity I0 Interfere, in a medium at a point, where phase different between them is 2π3. Then, the resultant intensity at that point would be.
View Solution