particles
particles are helium nuclei, so will form helium gas by trapping electrons )
The radioactive disintegration follows first order kinetics Starting with 1 mol of A in a 1- litre closed flask at pressure developed after tw0 half- lives is approximately.
24 atm
65 atm
61.5 atm
12 atm
The correct Answer is:C
To solve the problem, we will follow these steps:
Step 1: Understand the Reaction
The reaction given is:
A(g)→B(g)+α particles
where α particles are helium nuclei. The reaction follows first-order kinetics.
Step 2: Determine the Half-Life
For a first-order reaction, the half-life (t1/2) is constant and does not depend on the initial concentration. The half-life can be expressed as:
t1/2=0.693k
where k is the rate constant.
Step 3: Calculate Moles After Two Half-Lives
Starting with 1 mole of A:
- After the first half-life, the amount of A remaining is:
A1=12 moles of A
- After the second half-life, the amount of A remaining is:
A2=A12=14 moles of A
Thus, the amount of A that has reacted after two half-lives:
Reacted A=1−A2=1−14=34 moles of A
Step 4: Calculate Moles of Products Formed
From the stoichiometry of the reaction:
- For every mole of A that reacts, it produces:
- 1 mole of B
- 2 moles of α particles (since each α particle is a helium nucleus, we consider 2 moles of helium gas).
Thus, after the reaction:
- Moles of B formed:
Moles of B=34 moles
- Moles of α particles (which will form helium gas):
Moles of α=2×34=32 moles
Step 5: Calculate Total Moles in the System
Now, we can calculate the total moles in the system after two half-lives:
- Remaining moles of A:
Remaining A=14 moles
- Moles of B formed:
Moles of B=34 moles
- Moles of α (helium) formed:
Moles of α=32 moles
Total moles:
Ntotal=14+34+32=14+34+64=104=2.5 moles
Step 6: Calculate Pressure Using Ideal Gas Law
Using the ideal gas law:
PV=nRT
Where:
- n=2.5 moles
- R=0.0821 L atm/(K mol)
- T=27∘C=300 K
- V=1 L
Rearranging for pressure P:
P=nRTV=2.5×0.0821×3001
Calculating:
P=61.575 atm
Step 7: Final Answer
The pressure developed after two half-lives is approximately:
P≈61.5 atm
The reaction given is:
A(g)→B(g)+α particles
where α particles are helium nuclei. The reaction follows first-order kinetics.
For a first-order reaction, the half-life (t1/2) is constant and does not depend on the initial concentration. The half-life can be expressed as:
t1/2=0.693k
where k is the rate constant.
Starting with 1 mole of A:
- After the first half-life, the amount of A remaining is:
A1=12 moles of A
- After the second half-life, the amount of A remaining is:
A2=A12=14 moles of A
Reacted A=1−A2=1−14=34 moles of A
From the stoichiometry of the reaction:
- For every mole of A that reacts, it produces:
- 1 mole of B
- 2 moles of α particles (since each α particle is a helium nucleus, we consider 2 moles of helium gas).
- Moles of B formed:
Moles of B=34 moles
- Moles of α particles (which will form helium gas):
Moles of α=2×34=32 moles
Now, we can calculate the total moles in the system after two half-lives:
- Remaining moles of A:
Remaining A=14 moles
- Moles of B formed:
Moles of B=34 moles
- Moles of α (helium) formed:
Moles of α=32 moles
Ntotal=14+34+32=14+34+64=104=2.5 moles
Using the ideal gas law:
PV=nRT
Where:
- n=2.5 moles
- R=0.0821 L atm/(K mol)
- T=27∘C=300 K
- V=1 L
P=nRTV=2.5×0.0821×3001
P=61.575 atm
The pressure developed after two half-lives is approximately:
P≈61.5 atm
Similar Questions
Rutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
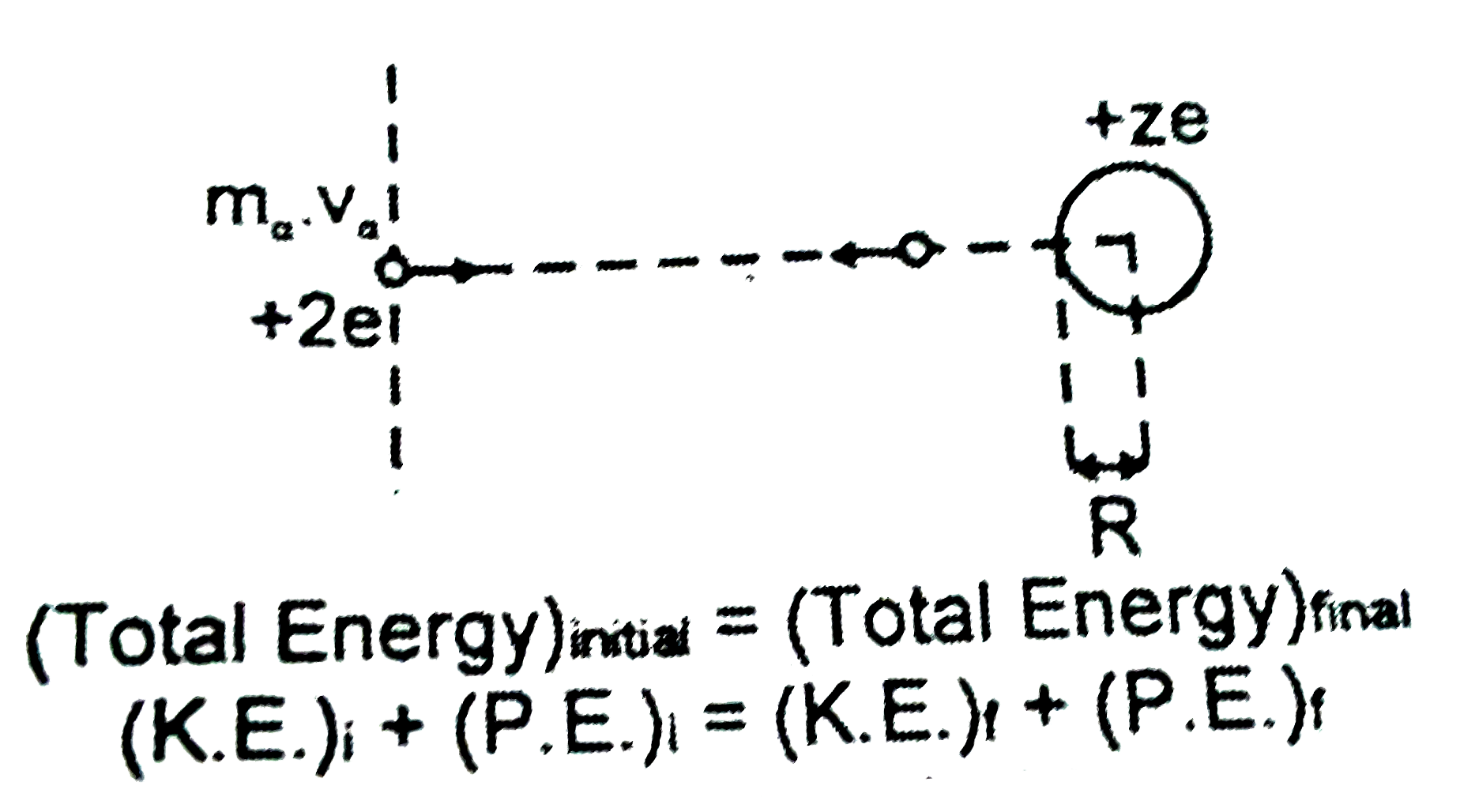
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
Radius of a particular nucleus is calculated by the projection of α-particle from infinity at a particular speed. Let this radius is the true radius . If the radius calculation for the same nucleus is made by another α-particle with half of the earlier speed, then the percentage error involved in the radius calculation is :
View SolutionRutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
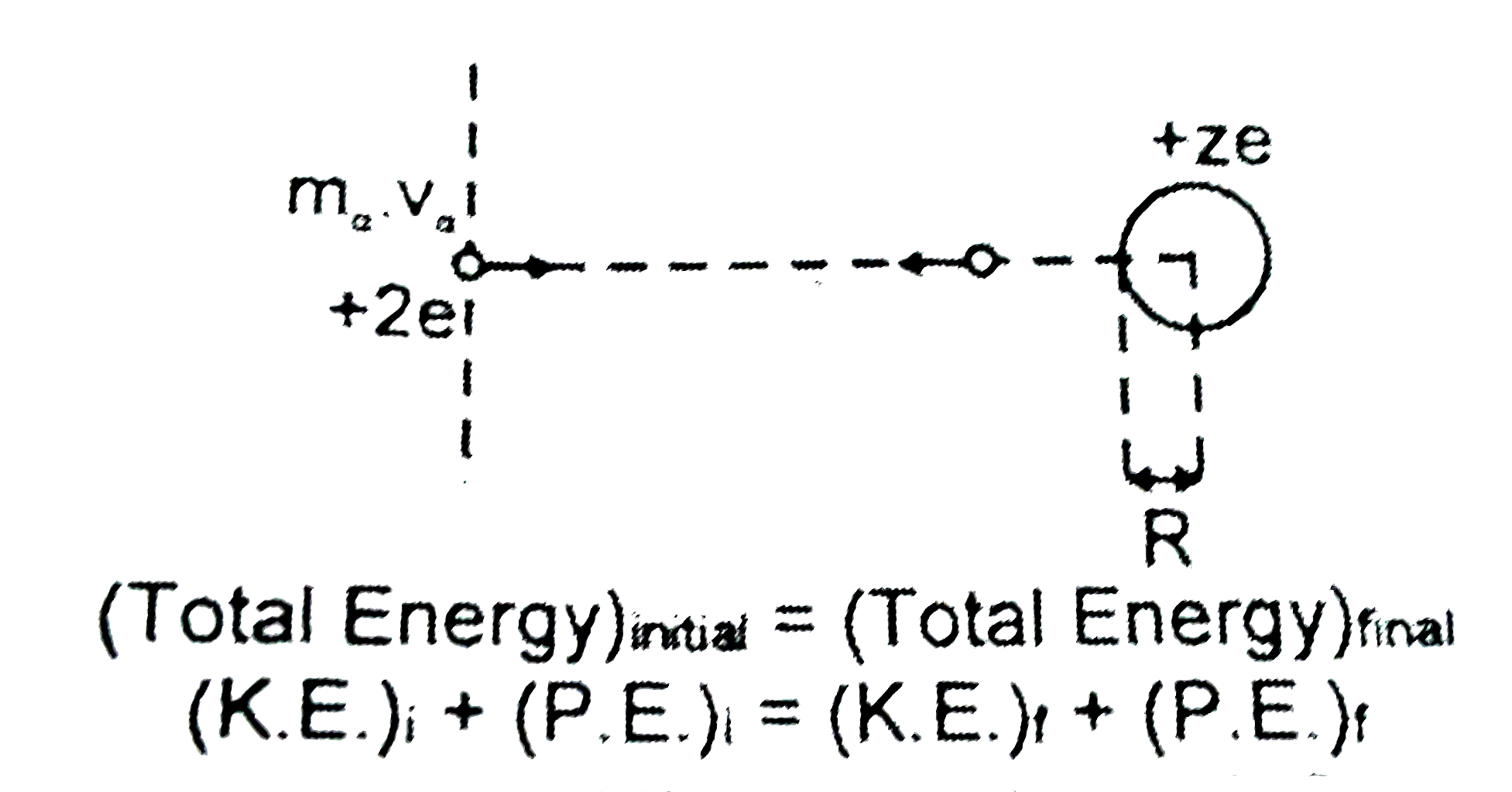
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
An α-particle with initial speed v0 is projected from infinity and it approaches up to r0 distance from a nuclie. Then, the initial speed of α-particle, which approaches upto 2r0 distance from the nucleus is :
View SolutionThe system shown in the figure is in equilibrium, where A and B are isomeric liquids and form an ideal solution at TK. Standard vapour pressures of A and B are P0A and P0B, respectively, at TK. We collect the vapour of A and B in two containers of volume V, first container is maintained at 2TK and second container is maintained at 3T/2. At the temperature greater than TK, both A and B exist in only gaseous form.
We assume than collected gases behave ideally at 2TK and there may take place an isomerisation reaction in which A gets converted into B by first-order kinetics reaction given as:
Ak−−→B, where k is a rate constant.
In container (II) at the given temperature 3T/2, A and B are ideal in nature and non reacting in nature. A small pin hole is made into container. We can determine the initial rate of effusion of both gases in vacuum by the expression

r=K.P√M0
where P= pressure differences between system and surrounding
K= positive constant
M0= molecular weight of the gas
Vapours of A and B are passed into a container of volume 8.21L, maintained at 2TK, where T=50K and after 5min, moles of B=8/3. The pressure developed into the cotainer after two half lives is
View SolutionA compound A dissociate by two parallel first order paths at certain temperature
A(g)k1(min−1)→2B(g)k1=6.93×10−3min−1
A(g)k2(min−1)→C(g)k2=6.93×10−3min−1
If reaction started with pure 'A' with 1 mole of A in 1 litre closed container with initial pressure (in atm) developed in container after 50 minutes from start of experiment?
View SolutionDuring alpha-decay , a nucleus decays by emitting an α-particle ( a helium nucleus .2He4) according to the equation
.AZX→.A−4Z−2Y+.42He+Q
In this process, the energy released Q is shared by the emitted α-particle and daughter nucleus in the form of kinetic energy .
The energy Q is divided in a definite ratio among the α-particle and the daughter nucleus .
A nucleus that decays spontaneously by emitting an electron or a positron is said to undergo β-decay .This process also involves a release of definite energy . Initially, the β-decay was represented as .AZX→.AZ+1Y+e−(electron)+Q
According to this reaction, the energy released during each decay must be divided in definite ratio by the emitted e' (β-particle) and the daughter nucleus. While , in alpha decay, it has been found that every emitted α-particle has the same sharply defined kinetic energy. It is not so in case of β-decay . The energy of emitted electrons or positrons is found to vary between zero to a certain maximum value. Wolfgang Pauli first suggested the existence of neutrinoes in 1930. He suggested that during β-decay, a third particle is also emitted. It shares energy with the emitted β particles and thus accounts for the energy distribution.
The beta particles (positron) are emitted with different kinetic energies because
View SolutionDuring alpha-decay , a nucleus decays by emitting an α-particle ( a helium nucleus .2He4) according to the equation
.AZX→.A−4Z−2Y+.42He+Q
In this process, the energy released Q is shared by the emitted α-particle and daughter nucleus in the form of kinetic energy .
The energy Q is divided in a definite ratio among the α-particle and the daughter nucleus .
A nucleus that decays spontaneously by emitting an electron or a positron is said to undergo β-decay .This process also involves a release of definite energy . Initially, the β-decay was represented as .AZX→.AZ+1Y+e−(electron)+Q
According to this reaction, the energy released during each decay must be divided in definite ratio by the emitted e' (β-particle) and the daughter nucleus. While , in alpha decay, it has been found that every emitted α-particle has the same sharply defined kinetic energy. It is not so in case of β-decay . The energy of emitted electrons or positrons is found to vary between zero to a certain maximum value. Wolfgang Pauli first suggested the existence of neutrinoes in 1930. He suggested that during β-decay, a third particle is also emitted. It shares energy with the emitted β particles and thus accounts for the energy distribution.
During β+ decay (positron emission) a proton in the nucleus is converted into a neutron, positron and neutrino. The reaction is correctly represented as
View SolutionDuring alpha-decay , a nucleus decays by emitting an α-particle ( a helium nucleus .2He4) according to the equation
.AZX→.A−4Z−2Y+.42He+Q
In this process, the energy released Q is shared by the emitted α-particle and daughter nucleus in the form of kinetic energy .
The energy Q is divided in a definite ratio among the α-particle and the daughter nucleus .
A nucleus that decays spontaneously by emitting an electron or a positron is said to undergo β-decay .This process also involves a release of definite energy . Initially, the β-decay was represented as .AZX→.AZ+1Y+e−(electron)+Q
According to this reaction, the energy released during each decay must be divided in definite ratio by the emitted e' (β-particle) and the daughter nucleus. While , in alpha decay, it has been found that every emitted α-particle has the same sharply defined kinetic energy. It is not so in case of β-decay . The energy of emitted electrons or positrons is found to vary between zero to a certain maximum value. Wolfgang Pauli first suggested the existence of neutrinoes in 1930. He suggested that during β-decay, a third particle is also emitted. It shares energy with the emitted β particles and thus accounts for the energy distribution.
When a nucleus of mass number A at rest decays emitting an α-particle , the daugther nucleus recoils with energy K . What is the Q value of the reaction ?
View SolutionCalculate the total pressure in a 10 litre cylinder which contains 0.4g of helium, 1.6g of oxygen and 1.4g of nitrogen at 27∘C. Also calculate the partial pressure of helium gas in the cylinder. Assume ideal behaviour of gases. Given R=0.082 litre atm K−1mol−1.
View SolutionFollowing are two first order reaction with their half times given at 25∘C.
At1/2=30min−−−−−−−→ Products
Bt1/2=40min−−−−−−−→ Products
The temperature coefficients of their reactions rates are 3 and 2, respectively, beween 25∘C and 35∘C. IF the above two resctions are carried out taking 0.4M of each reactant but at different temperatures: 25∘C for the first order reaction and 35∘C for the second order reaction, find the ratio of the concentrations of A and B after an hour.
View SolutionX-ray are emitted by a tube containing the electron gt Noibum (Z=41) as anticathode and the Kα X-ray an allowed to be incident on an unknown gas containing hydrogen- like atom ions. It is found that the X-ray cause the emission of photoelectrons with an energy of 2.7keV from these ions. Find
a. the minimum voltage at which the X-ray tube should be opreated so that the momentum of the emitted photoelectrons is doubled.
b. the approximate value of Z for the target of the anticathode after the momentum has been doubled.
c. the approximate value of Z the atomic number of the atom of the gas.
d. the number of the ion produced in the gas caused by X-ray , if the inetensity of X-ray is 100mWm−2 and 1% of the X-rays cause ionization.
View Solution
