A wave is represente by y=Asin2(kx−ωt+ϕ). The amplitude and wavelength of wave is given by
2A,2πk
A,2πk
A2,2πk
A2,πk
The correct Answer is:D
To solve the problem, we need to analyze the wave function given by:
y=Asin2(kx−ωt+ϕ)
Step 1: Rewrite the wave equation using trigonometric identities
We can use the trigonometric identity for sin2 to rewrite the wave equation. The identity states:
sin2θ=1−cos(2θ)2
Applying this identity to our wave function:
y=Asin2(kx−ωt+ϕ)=A⋅1−cos(2(kx−ωt+ϕ))2
This simplifies to:
y=A2(1−cos(2kx−2ωt+2ϕ))
Step 2: Identify the amplitude of the wave
From the rewritten equation, we can see that the amplitude of the wave is the coefficient of the cosine term. The term A2 indicates that the amplitude of the wave is:
Amplitude=A2
Step 3: Determine the wavelength of the wave
The general form of a wave is given by:
y=Acos(kx−ωt)
From this, we know that the wavelength λ is related to the wave number k by:
λ=2πk
In our case, the wave number k remains unchanged in the transformation we performed, so we can conclude that the wavelength is:
Wavelength=2πk
Final Answer
Thus, the amplitude and wavelength of the wave are:
- Amplitude = A2
- Wavelength = 2πk
The correct option is C: Amplitude = A2 and Wavelength = 2πk.
---
- Wavelength = 2πk
Similar Questions
Knowledge Check
A transverse wave is represented by y=Asin(ωt−kx). For what value of the wavelength is the wave velocity equal to the maximum particle velocity?
AπA2BπAC2πADAA travelling wave represented by
y=Asin(ωt−kx)
is superimposed on another wave represented by
y=Asin(ωt+kx). The resultant isAstanding wave having nodes at x=(n+12)λ2, n=0,1,2,….Bsatnding wave having nodes at x=nλ2,n=0,1,2..Cwave travelling along +x directionDwave travelling along-x direction
The displacement of a progressive wave is represented by y=Asin(ωt−kx), where x is distance and t is time. Write the dimensional formula of (i) ω and (ii) k.
View SolutionA progressive wave travelling along the positive x-direction is represented by y(x,t)=Asin(kx−ωt+ϕ). Its snapshot at t=0 is given in the figure.
For this wave, the phase is:
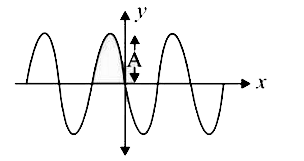
View SolutionA progressive wave travelling along the positive x-direction is represented by y(x,t)=Asin(kx−ωt+ϕ). Its snapshot at t=0 is given in the figure.
For this wave, the phase is:

View SolutionIn a stationary wave represented by y=4sin(ωt)cos(kx) cm, the amplitude of the component progressive wave is
View SolutionThe equation of a standing wave in a string fixed at both its ends is given as y=2A sin kx cos ωt. The amplitude and frequency of a particle vibrating at the point of string midway between a node and an antinode is
View SolutionTwo waves represented by y=asin(ωt−kx) and y=acos(ωt−kx) are superposed. The resultant wave will have an amplitude.
View Solution
