Two plane mirrors are placed at some angle. There are five images formed when an object is placed symmetrically between them . Find the angle between the mirrors.
The correct Answer is:A
To solve the problem of finding the angle between two plane mirrors that form five images when an object is placed symmetrically between them, we can follow these steps:
1. Understand the relationship between the number of images and the angle:
The formula to find the number of images (N) formed by two plane mirrors placed at an angle (θ) is given by:
N=360∘θ−1
2. Set up the equation with the given number of images:
According to the problem, the number of images formed (N) is 5. Therefore, we can write:
5=360∘θ−1
3. Rearrange the equation:
To isolate θ, we first add 1 to both sides:
5+1=360∘θ
This simplifies to:
6=360∘θ
4. Solve for θ:
Now, we can solve for θ by multiplying both sides by θ and then dividing by 6:
θ=360∘6
5. Calculate the angle:
Performing the division gives:
θ=60∘
6. Conclusion:
The angle between the two mirrors is 60∘.
Final Answer:
The angle between the mirrors is 60∘.
---
The formula to find the number of images (N) formed by two plane mirrors placed at an angle (θ) is given by:
N=360∘θ−1
According to the problem, the number of images formed (N) is 5. Therefore, we can write:
5=360∘θ−1
To isolate θ, we first add 1 to both sides:
5+1=360∘θ
This simplifies to:
6=360∘θ
Now, we can solve for θ by multiplying both sides by θ and then dividing by 6:
θ=360∘6
Performing the division gives:
θ=60∘
The angle between the two mirrors is 60∘.
The angle between the mirrors is 60∘.
Similar Questions
Knowledge Check
Two plane mirrors are placed parallel to each other at a distance L apart. A point object O placed between them, at a distance L/3 from one mirror. Both mirrors form multiple image. The distance between any two images cannot be
A(a)3L/2B(b)2L/2C(c)2LD(d)None of these
Two plane mirrors are placed making an angle θ in between them. Write an expression for the number of images formed of an object placed in between the mirrors. State the condition, if any.
View SolutionIf two mirrors are inclined at some angle and an object is placed between the mirrors and there are 7 images formed for an object, then the angle between the mirrors is
View SolutionNumber of images of an object kept symmetrically between two mirrors inclined at angle 72∘, would be
View SolutionNumber of images of an object kept symmetrically between two mirrors inclined at angle 72∘, would be
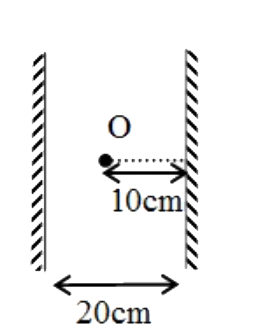
View SolutionTwo plane mirrors are kept parallel at 20 cm from each other. A point object O is placed exactly in between them. Calculate distance between second images formed by two mirros.
View SolutionTwo plane mirrors are kept such that angle between their reflecting surfaces is 40∘. A point object is kept symmetrically with respect to the mirrors. The total number of images formed by one mirror is :
View SolutionIf two mirrors are inclined at some angle θ. An object is placed between the mirrors and there are 5 images formed for an object, then θ is may be
View Solution
