A nuclear reactor delivers a power of 109 W. What is the amount of fuel consumed by the reactor in one hour?
6.610−5g
0.96g
4×10−2g
0.8g
The correct Answer is:C
To solve the problem of determining the amount of fuel consumed by a nuclear reactor delivering a power of 109 W in one hour, we can follow these steps:
Step 1: Understand the relationship between power, energy, and time.
The energy consumed by the reactor can be calculated using the formula:
E=P×t
where:
- E is the energy consumed (in joules),
- P is the power (in watts),
- t is the time (in seconds).
Step 2: Convert time from hours to seconds.
Since the power is given in watts (joules per second), we need to convert the time from hours to seconds.
1 hour = 3600 seconds.
Step 3: Calculate the energy consumed in one hour.
Substituting the values into the energy formula:
E=109W×3600s
Calculating this gives:
E=109×3600=3.6×1012J
Step 4: Relate energy to mass using Einstein's equation.
According to Einstein's mass-energy equivalence principle, the energy can also be expressed in terms of mass:
E=mc2
where:
- m is the mass (in kilograms),
- c is the speed of light (c≈3×108m/s).
Step 5: Rearrange the equation to find mass.
We can rearrange the equation to find the mass:
m=Ec2
Step 6: Substitute the values into the mass equation.
Substituting the values we have:
m=3.6×1012J(3×108m/s)2
Calculating c2:
c2=(3×108)2=9×1016m2/s2
Now substituting this back:
m=3.6×10129×1016=4×10−5kg
Step 7: Convert mass from kilograms to grams.
Since 1 kg = 1000 grams, we convert the mass:
m=4×10−5kg×1000g/kg=4×10−2g
Final Answer:
The amount of fuel consumed by the reactor in one hour is 4×10−2grams.
---
The energy consumed by the reactor can be calculated using the formula:
E=P×t
where:
- E is the energy consumed (in joules),
- P is the power (in watts),
- t is the time (in seconds).
Since the power is given in watts (joules per second), we need to convert the time from hours to seconds.
1 hour = 3600 seconds.
Substituting the values into the energy formula:
E=109W×3600s
Calculating this gives:
E=109×3600=3.6×1012J
According to Einstein's mass-energy equivalence principle, the energy can also be expressed in terms of mass:
E=mc2
where:
- m is the mass (in kilograms),
- c is the speed of light (c≈3×108m/s).
We can rearrange the equation to find the mass:
m=Ec2
Substituting the values we have:
m=3.6×1012J(3×108m/s)2
Calculating c2:
c2=(3×108)2=9×1016m2/s2
Now substituting this back:
m=3.6×10129×1016=4×10−5kg
Since 1 kg = 1000 grams, we convert the mass:
m=4×10−5kg×1000g/kg=4×10−2g
The amount of fuel consumed by the reactor in one hour is 4×10−2grams.
Similar Questions
What is the role of heavy water in a nuclear reactor?
View SolutionA nuclear reactor using .235U generates 250MW of electric power. The efficiency of the reactor (i.e., efficiency of conversion of thermal energy into electrical energy) is 25%. What is the amount of .235U used in the reactor per year? The thermal energy released per fission of .235U is 200MeV.
View SolutionIf the cell of emf 5 volt shown in the figure gives a power of 10W. Q, find the powers consumed by the resistors 2Ω and 1Ω
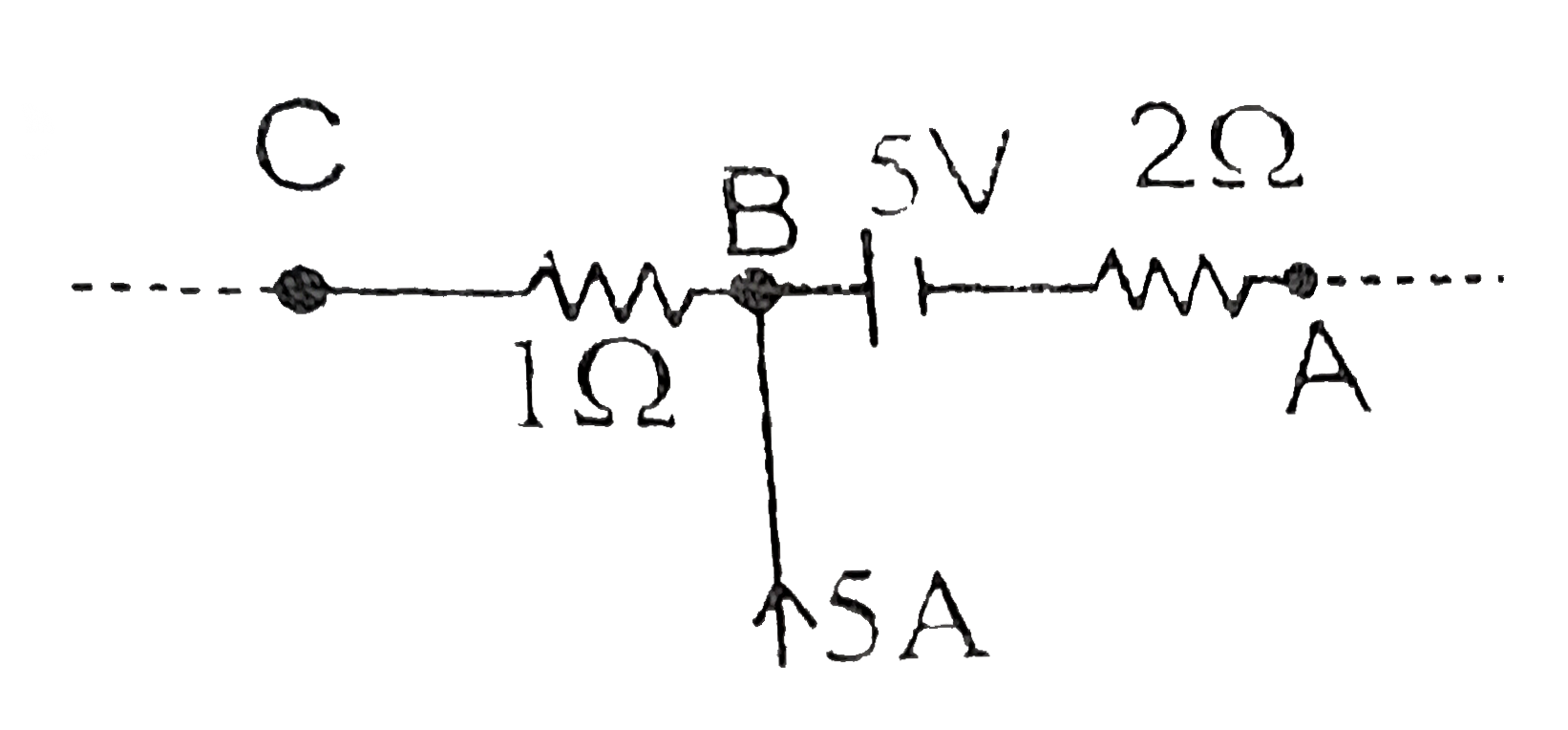
View SolutionIf the cell of emf 5 volt shown in the figure gives a power of 10W. Q, find the powers consumed by the resistors 2Ω and 1Ω

View SolutionA nuclear reactor has a power of 16 kW. If the energy released per fission is 200 MeV, the number of fissions per second is
View SolutionWhat is the amount of power delivered by the ac source in the circuit shown (in watts)

View SolutionA nuclear power reactor generates electric power of 100 MW. How many number of fissions occur per second if nuclear fuel used in the reactor is uranium ?
View SolutionWhat is nuclear reactor? Discuss the role of heavy water as moderator
View SolutionIn a uranium reactor whose thermal power is P=100MW, if the average number of neutrons liberated in each nuclear spitting is 2.5. Each splitting is assumed to release an energy E=200MeV. The number of neutrons generated per unit time is-
View SolutionA nuclear explosion is designed to deliver 1MW of heat energy, how many fission events must be required in a second to attain this power level. If this explosion is designed with a nuclear fuel consisting of uranium 235 to run a reactor at this power level for one year, then calculate the amount of fuel needed. You can assume that the calculate the amount of energy released per fission event is 200MeV.
View Solution
