A point on the periphery of a rotating disc has its acceleration vector making angle of 30∘ with the velocity . The ratio (ac/at(ac is centripetal acceleration and at is tangential acceleration) equals
sin30∘
cos30∘
tan30∘
none of these
The correct Answer is:C
To solve the problem, we need to find the ratio of centripetal acceleration ac to tangential acceleration at given that the acceleration vector makes an angle of 30∘ with the velocity vector.
1. Understanding the Acceleration Components:
- The total acceleration →a of a point on the rotating disc can be resolved into two components:
- Tangential acceleration at (acts along the direction of velocity).
- Centripetal acceleration ac (acts towards the center of the circular path).
2. Visualizing the Problem:
- Draw a diagram where the velocity vector →v is directed tangentially to the circular path.
- The acceleration vector →a makes an angle of 30∘ with the velocity vector →v.
3. Using Trigonometric Relationships:
- From the triangle formed by the vectors →a, →at, and →ac, we can use the cosine of the angle to relate the components:
cos(30∘)=aca
- And the sine of the angle to relate the tangential acceleration:
sin(30∘)=ata
4. Calculating the Ratios:
- We know that:
cos(30∘)=√32andsin(30∘)=12
- Therefore, we can express ac and at in terms of a:
ac=a⋅cos(30∘)=a⋅√32
at=a⋅sin(30∘)=a⋅12
5. Finding the Ratio:
- Now, we can find the ratio acat:
acat=a⋅√32a⋅12=√3212=√3
6. Final Result:
- Thus, the ratio of centripetal acceleration to tangential acceleration is:
acat=√3
- The total acceleration →a of a point on the rotating disc can be resolved into two components:
- Tangential acceleration at (acts along the direction of velocity).
- Centripetal acceleration ac (acts towards the center of the circular path).
- Draw a diagram where the velocity vector →v is directed tangentially to the circular path.
- The acceleration vector →a makes an angle of 30∘ with the velocity vector →v.
- From the triangle formed by the vectors →a, →at, and →ac, we can use the cosine of the angle to relate the components:
cos(30∘)=aca
- And the sine of the angle to relate the tangential acceleration:
sin(30∘)=ata
- We know that:
cos(30∘)=√32andsin(30∘)=12
- Therefore, we can express ac and at in terms of a:
ac=a⋅cos(30∘)=a⋅√32
at=a⋅sin(30∘)=a⋅12
- Now, we can find the ratio acat:
acat=a⋅√32a⋅12=√3212=√3
- Thus, the ratio of centripetal acceleration to tangential acceleration is:
acat=√3
Similar Questions
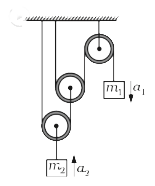
Find the relation between acceleration a1 and a2
View SolutionA particle is moving with a constant angular acceleration of 4rad/s2 in a circular path. At time t=0 , particle was at rest. Find the time at which the magnitudes of centripetal acceleration and tangential acceleration are equal.
View SolutionA point starts from rest and moves along a circular path with a constant tangential acceleration. After one rotation, the ratio of its radial acceleration to its tangential acceleration will be equal to
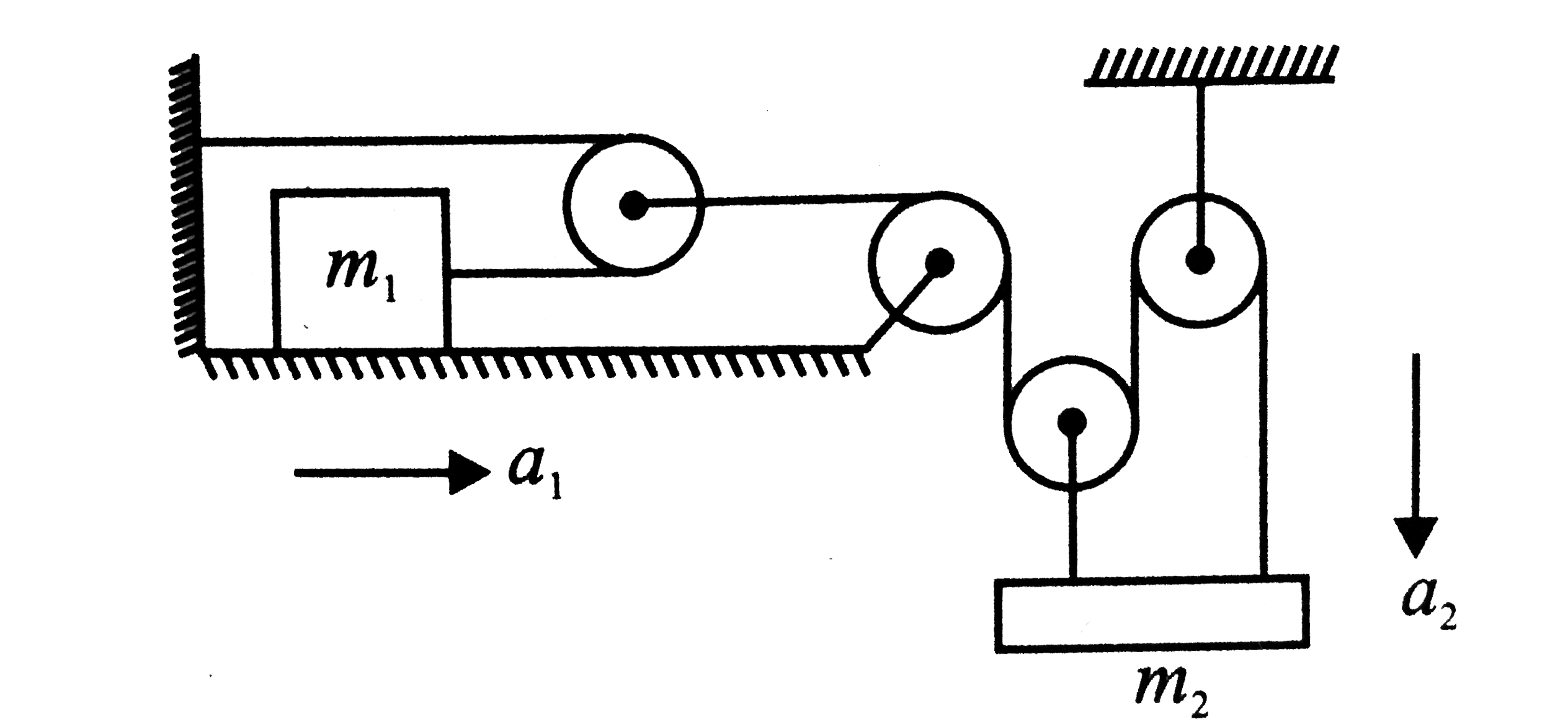
View SolutionTwo blocks are arranged as shown in figure. Find the ratio of a1/a2. (a1 is acceleration of m1 and a2 that of m2)
View SolutionA point at the periphery of a disc rotating about a fixed axis moves so that its speed varies with time as v=2t2m/s The tangential acceleration of the point at t= 1s is
View SolutionA particle is projected at t=0 with velocity u at angle θ with the horizontal. Then the ratio of the tangential acceleration and the radius of curvature at the point of projection is :
View SolutionAssertion:- A particle is moving in a circle with constant tangential acceleration such that its speed v is increasing. Angle made by resultant acceleration of the particle with tangential acceleration increases with time.
Reason:- Tangential acceleration =∣∣∣d→vdt∣∣∣ and centripetal acceleration =v2R
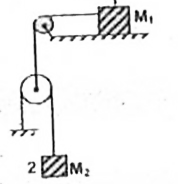
View SolutionIn given system a1anda2 are the accelerations of blocks 1 and 2. Then constraint relation is
View SolutionA particle moves in a circle with constant tangential acceleration starting from rest. At t = 1/2 s radial acceleration has a value 25% of tangential acceleration. The value of tangential acceleration will be correctly represented by
View SolutionCalculate the relative acceleration of A w.r.t. B if B is moving with acceleration a0 towards right.

View Solution
