A cylindrical wire of radius R has current density varying with distance r form its axis as J(x)=J0(1−r2R2) . The total current through the wire is
πJ0R22
2πJ0R23
4πJ0R23
none of these
The correct Answer is:A
To find the total current through a cylindrical wire with a given current density that varies with distance from the axis, we can follow these steps:
1. Understand the Current Density Function:
The current density J(r) is given by:
J(r)=J0(1−r2R2)
where J0 is a constant, r is the distance from the axis of the cylinder, and R is the radius of the cylinder.
2. Determine the Differential Area:
For a cylindrical shell at a distance r from the axis with thickness dr, the differential area dA can be expressed as:
dA=2πrdr
3. Calculate the Differential Current:
The differential current dI through this differential area can be calculated using the formula:
dI=J(r)⋅dA
Substituting the expressions for J(r) and dA:
dI=J0(1−r2R2)⋅(2πrdr)
4. Integrate to Find Total Current:
To find the total current I, integrate dI from r=0 to r=R:
I=∫R0dI=∫R0J0(1−r2R2)(2πrdr)
This can be separated into two integrals:
I=2πJ0∫R0(r−r3R2)dr
5. Evaluate the Integrals:
Calculate the two integrals:
- The first integral:
∫R0rdr=(r22)R0=R22
- The second integral:
∫R0r3dr=(r44)R0=R44
Substitute these results back into the expression for I:
I=2πJ0(R22−1R2⋅R44)
Simplifying this gives:
I=2πJ0(R22−R24)=2πJ0(2R24−R24)=2πJ0⋅R24=πJ0R22
6. Final Result:
The total current through the wire is:
I=πJ0R22
The current density J(r) is given by:
J(r)=J0(1−r2R2)
where J0 is a constant, r is the distance from the axis of the cylinder, and R is the radius of the cylinder.
For a cylindrical shell at a distance r from the axis with thickness dr, the differential area dA can be expressed as:
dA=2πrdr
The differential current dI through this differential area can be calculated using the formula:
dI=J(r)⋅dA
Substituting the expressions for J(r) and dA:
dI=J0(1−r2R2)⋅(2πrdr)
To find the total current I, integrate dI from r=0 to r=R:
I=∫R0dI=∫R0J0(1−r2R2)(2πrdr)
This can be separated into two integrals:
I=2πJ0∫R0(r−r3R2)dr
Calculate the two integrals:
- The first integral:
∫R0rdr=(r22)R0=R22
- The second integral:
∫R0r3dr=(r44)R0=R44
I=2πJ0(R22−1R2⋅R44)
Simplifying this gives:
I=2πJ0(R22−R24)=2πJ0(2R24−R24)=2πJ0⋅R24=πJ0R22
The total current through the wire is:
I=πJ0R22
Similar Questions
An infinite cylindrical wire of radius R and having current density varying with its radius r as, J=J0[1−(r/R)]. Then answer the following questions.
Graph between the magnetic field and radius is
View SolutionThe current density is a solid cylindrical wire a radius R, as a function of radial distance r is given by J(r)=J0(1−rR) . The total current in the radial regon r = 0 to r=R4 will be :
View SolutionA current is flowing through a cylinderical conductor of radius R, such that current density at any cross-section is given by J=J0(1−rR), where r is radial distance from axis of the cylinder .Calculate the total current through the cross-section of the conductor.
View SolutionThe mass density of a planet of radius R varie with the distance r form its centre as ρ(r)=p0(1−r2R2). Then the graviational field is maximum at :
View SolutionThe current density across a cylindrical conductor of radius R varies in magnitude according to the equation J=J0(1−rR) where r is the distance from the central axis. Thus, the current density is a maximum J0 at that axis (r = 0) and decreases linearly to zero at the surface (r = R). Calculate the current in terms of J0 and the conductor 's cross - sectional area A=πR2.
View SolutionThe magnetic field at point P in the hollow cylindrical wire carrying a current i shown in the figure at a distance r from axis (a<r<b) is given by (Taking current density to be uniform across its cross-section)
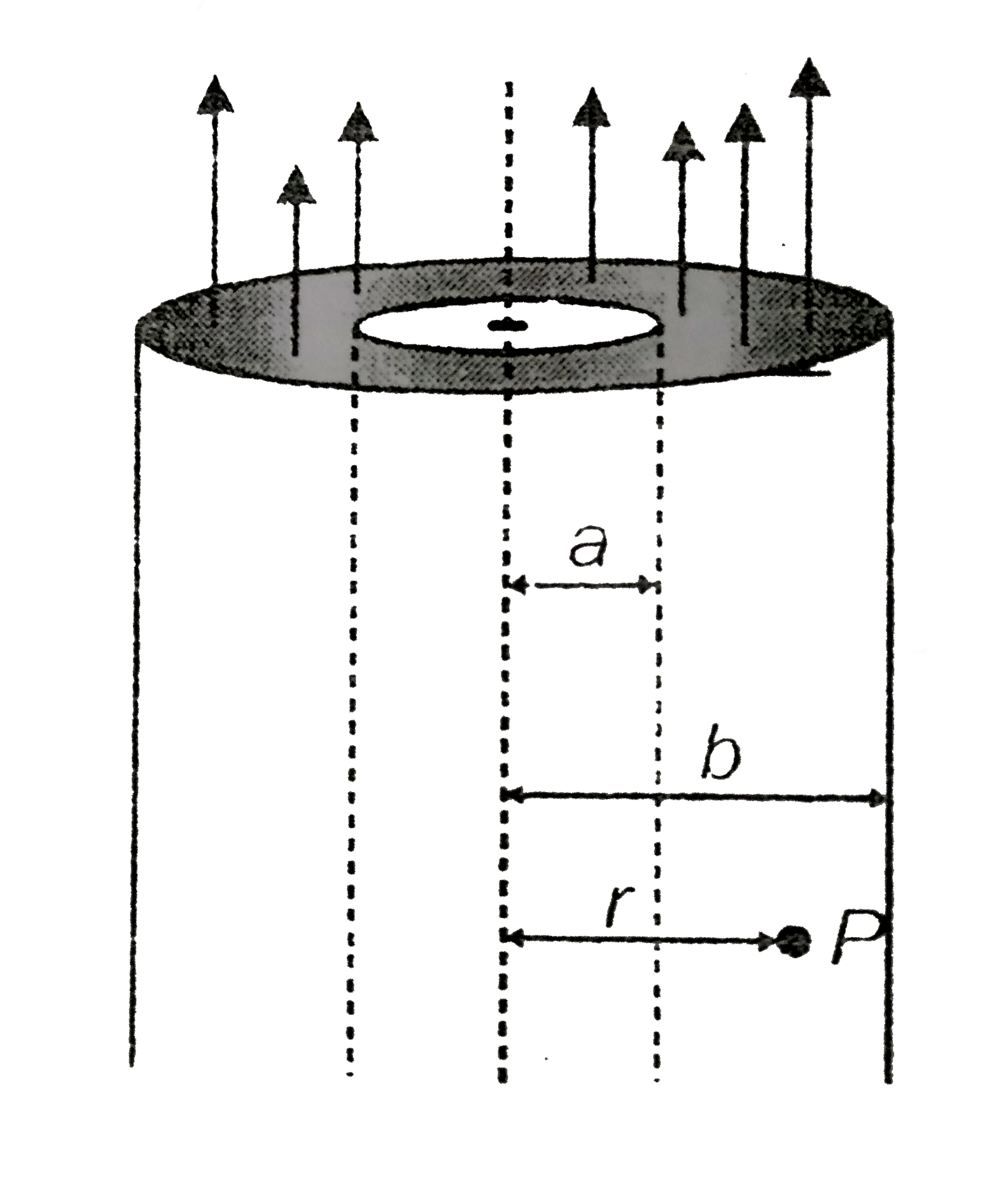
View SolutionAn infinitely long cylinderical wire of radius R is carrying a current with current density j=αr3 (where α is constant and r is the distance from the axis of the wire). If the magnetic fixed at r=R2 is B1 and at r=2R is B2 then the ratio B2B1 is
View SolutionConsider a long cylindrical wire carrying current along the axis of wire. The current distributed non-uniformly on cross section. The magnetic field B inside the wire varies with distance r from the axis as B=kr4 where k is a cosntant. The current density J varies with distance r as J=crn then the value of n is (where c is another constant).
View SolutionConsider a long cylindrical wire carrying current along the axis of wire. The current distributed non-uniformly on cross section. The magnetic field B inside the wire varies with distance r from the axis as B=kr4 where k is a cosntant. The current density J varies with distance r as J=crn then the value of n is (where c is another constant).
View SolutionElectric current is passing unfiormly through a solid cylinderical wire of radius R. The current I passing through circular cross-section of radius r measured from the axis of the cylinder (r<R) varies as shown in
View Solution
