Two satellite A and B , ratio of masses 3:1 are in circular orbits of radii r and 4 r . Then ratio of total mechanical energy of A to B is
(a)13
(b)3
(c)34
(d)12
The correct Answer is:D
To solve the problem of finding the ratio of total mechanical energy of satellites A and B, we can follow these steps:
Step 1: Understand the formula for total mechanical energy of a satellite
The total mechanical energy (E) of a satellite in a circular orbit is given by the formula:
E=−GMm2r
where:
- G is the gravitational constant,
- M is the mass of the planet,
- m is the mass of the satellite,
- r is the radius of the orbit.
Step 2: Define the variables for satellites A and B
Let:
- Mass of satellite A, m1=3m (since the ratio of masses is 3:1),
- Mass of satellite B, m2=m,
- Radius of orbit for satellite A, r1=r,
- Radius of orbit for satellite B, r2=4r.
Step 3: Calculate the total mechanical energy for each satellite
Using the formula for total mechanical energy:
For satellite A:
EA=−GMm12r1=−GM(3m)2r=−3GMm2r
For satellite B:
EB=−GMm22r2=−GMm2(4r)=−GMm8r
Step 4: Find the ratio of total mechanical energy of A to B
Now, we need to find the ratio EAEB:
EAEB=−3GMm2r−GMm8r=3GMm2r⋅8rGMm
Step 5: Simplify the ratio
Cancelling out the common terms G,M,m, and r:
EAEB=3⋅82=242=12
Conclusion
The ratio of total mechanical energy of satellite A to satellite B is:
EAEB=12:1
Final Answer
Thus, the ratio of total mechanical energy of A to B is 12:1.
---
The total mechanical energy (E) of a satellite in a circular orbit is given by the formula:
E=−GMm2r
where:
- G is the gravitational constant,
- M is the mass of the planet,
- m is the mass of the satellite,
- r is the radius of the orbit.
Let:
- Mass of satellite A, m1=3m (since the ratio of masses is 3:1),
- Mass of satellite B, m2=m,
- Radius of orbit for satellite A, r1=r,
- Radius of orbit for satellite B, r2=4r.
Using the formula for total mechanical energy:
EA=−GMm12r1=−GM(3m)2r=−3GMm2r
EB=−GMm22r2=−GMm2(4r)=−GMm8r
Now, we need to find the ratio EAEB:
EAEB=−3GMm2r−GMm8r=3GMm2r⋅8rGMm
Cancelling out the common terms G,M,m, and r:
EAEB=3⋅82=242=12
The ratio of total mechanical energy of satellite A to satellite B is:
EAEB=12:1
Thus, the ratio of total mechanical energy of A to B is 12:1.
Similar Questions
Two satellites P and Q ratio of masses 3:1 are in circular orbits of radii rand8r. Then ratio of total mechanical energy of A to B is
View SolutionTwo satellite A and B, ratio of masses 3:1 are in circular orbits of radii rand4r. Then ratio mechanical energy of A and B is
View SolutionFor a satellite moving in a circular orbit around the earth, the ratio of its total mechanical energy to kinetic energy
View SolutionTwo satellites , A and B , have masses m and 2m respectively . A is in a circular orbit of radius R , and B is in a circular orbit of radius 2R around the earth . The ratio of their energies , KAKB is :
View SolutionTwo satellites, A and B, have masses m and 2m respectively. A is in a circular orbit of radius R, and B is in a circular orbit of radius 2R around the earth. The ratio of their kinetic energies, TA/TB, is :
View SolutionTwo satellites A and B go around a planet in circular orbits of radii 4 R and R respectively. If the speed of the satellite A is 3 V, then the speed of the satellite B will be
View SolutionConsider two satellites A and B of equal mass, moving in the same circular orbit of radius r around the earth but in the opposite sense and therefore a collision occurs.
(a) Find the total mechanical energy EA+EB of the two satellite-plus-earth system before collision.
(b) If the collision is completely inelastic, find the total mechanical energy immediately after collision. Describe the subsequent motion of the combined satellite.
View Solution_E01_109_Q01.png)
Consider two satellites A and B of equal mass m, moving in the same circular orbit of radius r around the earth E but in opposite sense of rotation and therefore on a collision course (see figure)
(i). In terms of G,Me m and r find the total mechanical energy EA+EB of the two satellite plus earth system before collision
(ii). If the collision is completely inelastic so that wreckage remains as one piece of tangled material (mass=2m) find the total mechanical energy immediately after collision.
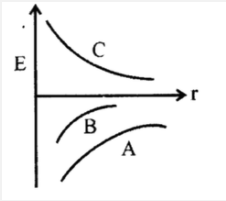
View SolutionAn artificial satellite is made to move in circular orbits of different radii around the earth. The variations of its K.E., P.E. and total energy (E) in different orbits is shown in the figure by different curves. Then for the satellites
View SolutionTwo satellites P and Q go round a planet in circular orbits of radii 9R and R respectively. If the speed of the satellite P is 4V, then speed of satellite Q will be
View Solution
