A bullet of mass m leaves a gun of mass M kept on a smooth horizontal surface . If the speed of the bullet relative to the gun is v , the magnitude of recoil speed of the gun will be
mMv
mM+mv
mM−mv
Mmv
The correct Answer is:B
To solve the problem, we will use the principle of conservation of momentum and the relationship between the velocities of the bullet and the gun. Here are the steps to derive the magnitude of the recoil speed of the gun:
1. Understand the Given Information:
- Mass of the bullet = m
- Mass of the gun = M
- Speed of the bullet relative to the gun = v
2. Define the Directions:
- Assume the positive direction is the direction in which the bullet moves when fired.
- The gun will recoil in the opposite direction, which we will consider as negative.
3. Establish the Relationship of Velocities:
- Let Vg be the recoil speed of the gun (which will be negative).
- Let Vb be the speed of the bullet after it leaves the gun.
- According to the relative speed, we have:
v=Vb−Vg
- Since the gun recoils backward, we can express Vg as negative:
v=Vb−(−Vg)⟹v=Vb+Vg
4. Use Conservation of Momentum:
- Initially, the system (gun + bullet) is at rest, so the initial momentum is 0.
- After firing, the final momentum must also equal 0:
Final Momentum=Momentum of the bullet+Momentum of the gun
0=mVb−MVg
- Rearranging gives:
mVb=MVg⟹Vg=mMVb
5. Substitute for Vb:
- From the earlier relationship v=Vb+Vg, substitute Vg:
v=Vb+mMVb
- Factor out Vb:
v=Vb(1+mM)
- Solve for Vb:
Vb=v1+mM=vMM+m
6. Find the Recoil Speed of the Gun:
- Substitute Vb back into the equation for Vg:
Vg=mMVb=mM(vMM+m)
- Simplifying gives:
Vg=mvM+m
7. Magnitude of the Recoil Speed:
- The magnitude of the recoil speed of the gun is:
|Vg|=mvM+m
Final Answer:
The magnitude of the recoil speed of the gun is:
mvM+m
- Mass of the bullet = m
- Mass of the gun = M
- Speed of the bullet relative to the gun = v
- Assume the positive direction is the direction in which the bullet moves when fired.
- The gun will recoil in the opposite direction, which we will consider as negative.
- Let Vg be the recoil speed of the gun (which will be negative).
- Let Vb be the speed of the bullet after it leaves the gun.
- According to the relative speed, we have:
v=Vb−Vg
- Since the gun recoils backward, we can express Vg as negative:
v=Vb−(−Vg)⟹v=Vb+Vg
- Initially, the system (gun + bullet) is at rest, so the initial momentum is 0.
- After firing, the final momentum must also equal 0:
Final Momentum=Momentum of the bullet+Momentum of the gun
0=mVb−MVg
- Rearranging gives:
mVb=MVg⟹Vg=mMVb
- From the earlier relationship v=Vb+Vg, substitute Vg:
v=Vb+mMVb
- Factor out Vb:
v=Vb(1+mM)
- Solve for Vb:
Vb=v1+mM=vMM+m
- Substitute Vb back into the equation for Vg:
Vg=mMVb=mM(vMM+m)
- Simplifying gives:
Vg=mvM+m
- The magnitude of the recoil speed of the gun is:
|Vg|=mvM+m
The magnitude of the recoil speed of the gun is:
mvM+m
Similar Questions
A bullet of mass m embeds itself in a block of mass M resting on a smooth horizontal surface, attached to a spring of force constant k. If the initial speed of the bullet is v0 along horizontal, find (a) the maximum compression of the spring and (b) the time for the bullet - block system to come to rest.
View SolutionA man of mass m is standing on a plank of equal mass m resting on a smooth horizontal surface. The man starts moving on the plank with speed u relative to the plank. The speed of the man relative to the ground is

View SolutionA gun of mass M(including the carriage) fires a shot of mass m. The gun along with the carriage is kept on a smooth horizontal surface. The muzzle speed of the bullet vr is constant. Find
(a) The elevation of the gun with horizontal at which maximum range of bullet with respect to the ground is obtained.
(b) The maximum range of the bullet.
View SolutionA shell of mass 0.020 kg is fired by a gun of mass 100 kg. If the speed of the shell is 80ms, the recoil speed of the gun would be
View SolutionA shell of mass 200g is fired by a gun of mass 100kg. If the muzzle speed of the shell is 80ms−1, then the recoil speed of the gun is
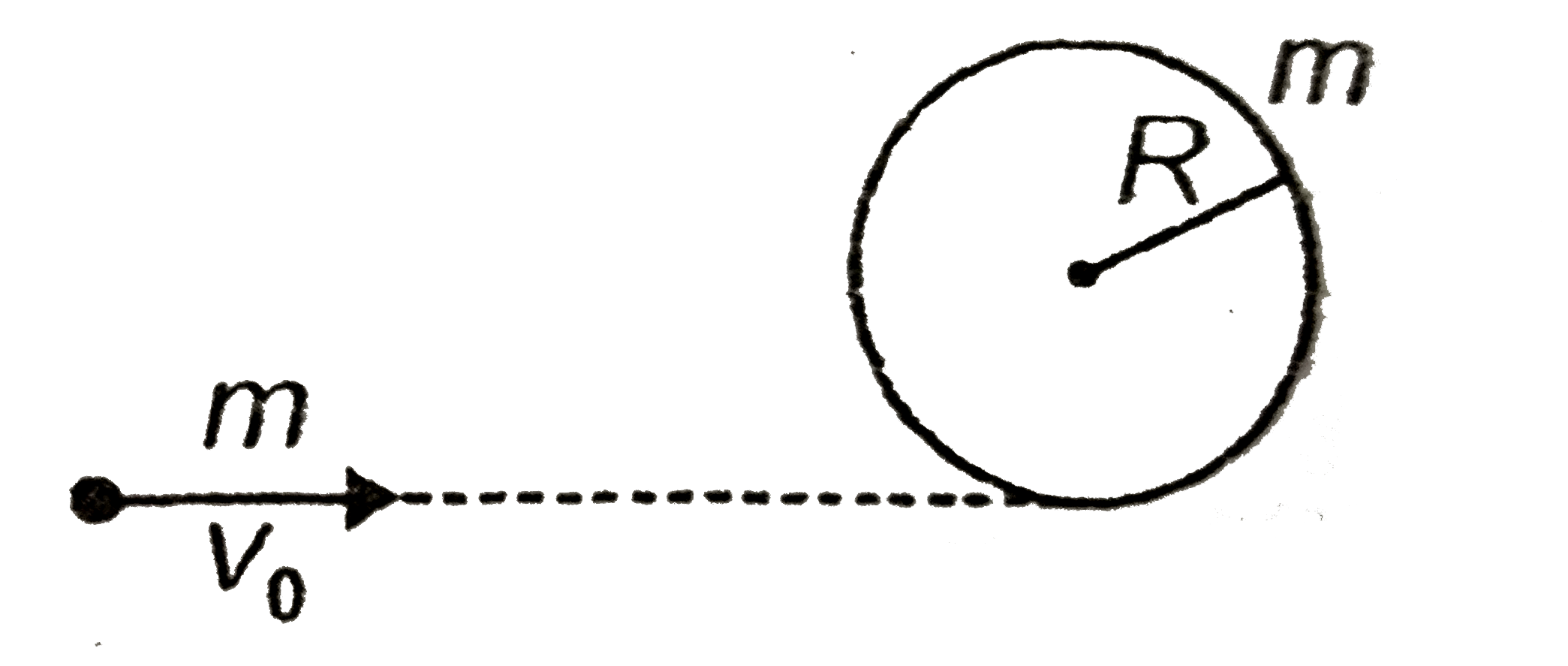
View SolutionA disc of mass m and radius R is kept on a smooth horizontal surface with its plane parallel to the surface. A particle of same mass m travelling with speed v0 collides with the stationery disc and gets embedded into it as shown in the figure. Then
View SolutionA shell of mass 0.02 kg is fird by a gun of mass 10 kg. If the muzzle speed of the shell is 600 ms−1, what is the recoil speed of the gun?
View SolutionA bullet mass 10gm is fired from a gun of mass 1 kg . If the recoil velocity is 5 m/s, the velocity of the muzzle is
View SolutionA bullet mass 10gm is fired from a gun of mass 1 kg . If the recoil velocity is 5 m/s, the velocity of the muzzle is
View SolutionA bullet mass 10gm is fired from a gun of mass 1 kg . If the recoil velocity is 5 m/s, the velocity of the muzzle is
View Solution
