A body of mass m is placed at the centre of the spherical shell of radius R and mass M. The gravitation potential on the surface of the shell is
−GR(M+m)
−GR(M−m)
−GR(mMM+m)
−GR(mMM−m)
The correct Answer is:A
To find the gravitational potential on the surface of a spherical shell with a mass M and radius R when a body of mass m is placed at the center, we can follow these steps:
Step 1: Understand Gravitational Potential
Gravitational potential V at a distance r from a mass M is given by the formula:
V=−GMr
where G is the gravitational constant.
Step 2: Consider the Spherical Shell
For a spherical shell of mass M, the gravitational potential inside the shell (at the center) is constant and equal to the potential on the surface of the shell. This is a key property of spherical shells in gravitational fields.
Step 3: Calculate the Potential on the Surface
At the surface of the shell (where r=R), the potential due to the mass M of the shell is:
V=−GMR
Step 4: Add the Contribution from the Mass m
Since the mass m is at the center, it does not contribute to the potential at the surface of the shell. The potential due to mass m at the surface of the shell is zero because the gravitational potential is defined relative to the mass creating it.
Step 5: Final Expression for Potential
Thus, the total gravitational potential V at the surface of the shell is simply:
V=−GMR
Conclusion
The gravitational potential on the surface of the shell is:
V=−GMR
Gravitational potential V at a distance r from a mass M is given by the formula:
V=−GMr
where G is the gravitational constant.
For a spherical shell of mass M, the gravitational potential inside the shell (at the center) is constant and equal to the potential on the surface of the shell. This is a key property of spherical shells in gravitational fields.
At the surface of the shell (where r=R), the potential due to the mass M of the shell is:
V=−GMR
Since the mass m is at the center, it does not contribute to the potential at the surface of the shell. The potential due to mass m at the surface of the shell is zero because the gravitational potential is defined relative to the mass creating it.
Thus, the total gravitational potential V at the surface of the shell is simply:
V=−GMR
The gravitational potential on the surface of the shell is:
V=−GMR
Similar Questions
A particle of mass M is placed at the centre of a uniform spherical shell of equal mass and radius a. Find the gravitational potential at a point P at a distance a2 from the centre.
View SolutionA particle of mass M is placed at the centre of a uniform spherical shell of equal mass and radius a. Find the gravitational potential at a point P at a distance a2 from the centre.
View Solution
Knowledge Check
A particle of mass m is placed at the centre of a unifrom spherical shell of mass 3 m and radius R The gravitational potential on the surface of the shell is .
AGMRB−GMRC−4GMRD−2GMRA point mass m is placed inside a spherical shell of radius R and mass M at a distance R2 form the centre of the shell. The gravitational force exerted by the shell on the point mass is
AGMmR2B2GMmR2CzeroD4MmR2A particle of mass M is placed at the centre of a spherical shell of same mass and radius a. What will be the magnitude of the gravitational potential at a point situated at a/2 distance from the centre ?
A−3GMRB−2GMRC−GMRD−4GMR
A particle of mass M is placed at the centre of a uniform spherical shell of equal mass and radius a. Find the gravitational potential at a point P at a distance a2 from the center.
View SolutionA point charge q is placed at a distance of r from the centre O of an uncharged spherical shell of inner radius R and outer radius 2R. The electric potential at the centre of the shell will be

View SolutionA spherical conductor having charge q and radius r is placed at the centre of a spherical shell of radius R and having charge Q (R > r). The potential difference between the two is
View SolutionA block of mass m is lying at a distance r from a spherical shell of mass m and radius r, then :
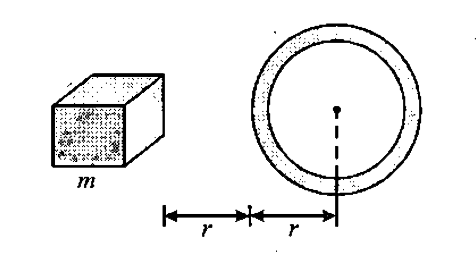
A. only gravitational field inside the shell is zero
B. gravitational field and gravitational potential both are zero inside the shell
C. gravitational potential as well as gravitational field inside the shell are not zero .
D. can't be ascertained
View SolutionA hollow spherical shell is compressed to half its radius. The gravitational potential at the centre
View Solution
