A particle of mass 1 kg is moving in SHM with path length 0.01 m and a frequency of 50 Hz. The maximum force in newton, acting on the particle is
150π2
200π2
100π2
50π2
The correct Answer is:C
Step by step video & image solution for A particle of mass 1 kg is moving in SHM with path length 0.01 m and a frequency of 50 Hz. The maximum force in newton, acting on the particle is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
A particle of mass 10g is undergoing SHM of amplitude 10cm and period 0.1s. The maximum value of force on particle is about
View SolutionA particle of mass 0.10 kg executes SHM with an amplitude 0.05 m and frequency 20 vib/s. Its energy of oscillation is
View SolutionA particle of mass 2 kg is moving in circular path with constant speed 20 m/s. The magnitude of change in velocity when particle travels from A to P will be
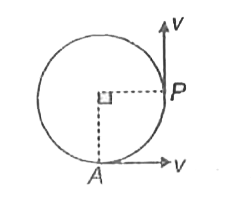 View Solution
View SolutionA particle executing S.H.M. having amplitude 0.01 m and frequency 60 Hz. Determine maximum acceleration of particle.
View SolutionA particle of mass m is rotating in a plane in circular path of radius r. Its angular momentum is L. The centripetal force acting on the particle is
View SolutionA particle of mass m=3 kg is moving on a straight line in a conservative force field in which the potential energy associated with the particle is given by U(y)=2y2−30y+450. At t=0, particle is at y=8m and moving towards positive y- axis with 1m/s
View SolutionThe breaking tension of a string is 10 N. A particle of mass 0.1 kg tied to it is rotated along a horizontal circle of radius 0.5 meter. The maximum speed with which the particle can be rotated without breaking the string is
View SolutionA particle of mass m executing SHM with angular frequency ω0 and amplitude A0, The graph of velocity of the particle with the displacement of the particle from mean position will be(ω0=1)
View SolutionThe amplitude of a particle executing simple harmonic motion with a frequency of 60 Hz is 0.01 m. Determine the maximum value of the acceleration of the particle.
View SolutionThe force of a particle of mass 1 kg is depends on displacement as F = —4x then the frequency of S.H.M. is
View Solution
