Two convex lenses of power 2 D and 3 D are separated by a distance 13m. The power of the optical system formed is
(a)+2D
(b)−2D
(c)−3D
(d)+3D
The correct Answer is:D
To solve the problem of finding the equivalent power of two convex lenses separated by a distance, we can follow these steps:
1. Identify the Given Values:
- Power of the first lens, P1=2D
- Power of the second lens, P2=3D
- Distance between the lenses, d=13m
2. Use the Formula for Equivalent Power:
The formula for the equivalent power Peq of two lenses separated by a distance d is given by:
Peq=P1+P2−d⋅P1⋅P2f1⋅f2
However, since we are using powers directly, we can simplify it to:
Peq=P1+P2−d⋅P1⋅P2
3. Substitute the Values:
Substitute the known values into the equation:
Peq=2+3−(13⋅2⋅3)
4. Calculate the Product Term:
Calculate 13⋅2⋅3:
13⋅2⋅3=63=2
5. Complete the Calculation:
Now substitute this back into the equation:
Peq=2+3−2=3
6. Final Result:
Therefore, the equivalent power of the optical system is:
Peq=3D
Conclusion:
The power of the optical system formed by the two lenses is +3D.
---
- Power of the first lens, P1=2D
- Power of the second lens, P2=3D
- Distance between the lenses, d=13m
The formula for the equivalent power Peq of two lenses separated by a distance d is given by:
Peq=P1+P2−d⋅P1⋅P2f1⋅f2
However, since we are using powers directly, we can simplify it to:
Peq=P1+P2−d⋅P1⋅P2
Substitute the known values into the equation:
Peq=2+3−(13⋅2⋅3)
Calculate 13⋅2⋅3:
13⋅2⋅3=63=2
Now substitute this back into the equation:
Peq=2+3−2=3
Therefore, the equivalent power of the optical system is:
Peq=3D
The power of the optical system formed by the two lenses is +3D.
Similar Questions
Two lenses of power 15D and -3D are placed in contact. The focal length of the combination is a
View SolutionTwo thin lenses of power +4 D and -2 D are in contact. What is the focal length of the combination ?
View SolutionTwo lenses of power 6 D and – 2 D are combined to form a single lens. The focal length of this lens will be
View SolutionTwo thin lenses of power +5 D and -2.5 D are in contact. What is the focal length of the combination ?
View SolutionTwo thin lenses of power +6Dand−2D are in contact. What is the focal length of the combination ?
View SolutionTwo lenses of power −15D and +5D are in contact with each other. The focal length of the combination is
View SolutionTwo thin lenses have a combined power of +9D.When they are separated by a distance of 20 cm, then their equivalent power becomes +275 D. Their individual powers (in dioptre) are
View SolutionIf the optic axis of convex and concave lenses are separated by a distance 5mm as shown in the figure. Find the coordinate of the final image formed by the combination if parallel beam of light is incident on lens. Origin is at the optical centre of convex lens
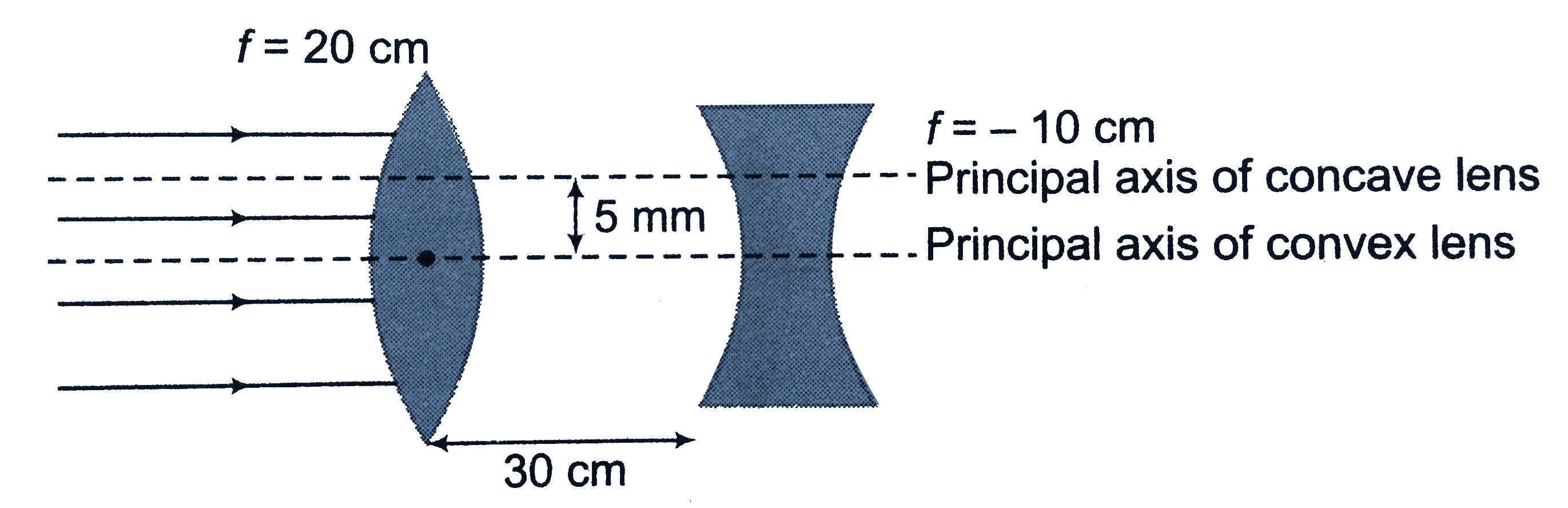
View SolutionA compound microscope consists of two convex lenses of focal length 2 cm and 5 cm. When an object is kept at a distance 2.1 cm from the objective, a virtual and magnified image is formed 25 cm from the eye piece. Calculate the magnifying power of the microscope.
View SolutionTwo thin convex lenses of focal lengths f1 and f2 are separated by a horizontal distance d (whered<f1,d<f2) and their centres are displaced by a vertical separation △ as shown in the fig.
Taking the origin of coordinates O, at the centre of the first lens the x and y coordinates of the focal point of this lens system, for a parallel beam of rays coming form the left, are given by:
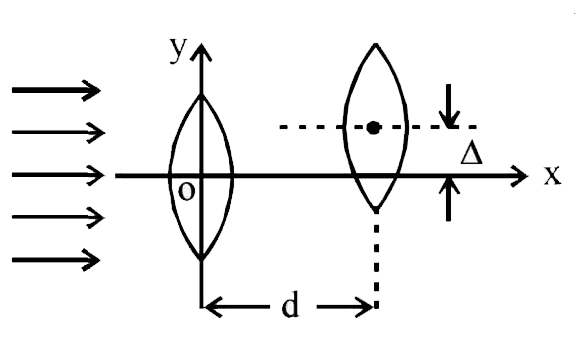
View Solution
