If θ is the polarising angle for two optical media whose critical angles are C1andC2 , then the correct relation is
sinθ=sinC2sinC1
θ=sinC2sinC1
tanθ=sinC1sinC2
sinθ=sinC1sinC2
The correct Answer is:C
To solve the problem, we need to establish the relationship between the polarizing angle (θ) and the critical angles (C1 and C2) for two optical media.
1. Understanding Brewster's Law: According to Brewster's Law, the tangent of the polarizing angle (θ) is given by the ratio of the refractive indices of the two media:
tanθ=n2n1
2. Relating Refractive Index to Critical Angle: The critical angle (C) for total internal reflection can be expressed using Snell's Law. For a medium with refractive index n1 (denser) and air (with refractive index n2=1), the critical angle C1 can be defined as:
n1sinC1=n2sin90∘
Since sin90∘=1, we can simplify this to:
n1=1sinC1
3. Applying the Same for the Second Medium: Similarly, for the second medium with critical angle C2:
n2=1sinC2
4. Substituting the Refractive Indices: Now we can substitute the expressions for n1 and n2 into the equation from Brewster's Law:
tanθ=n2n1=1sinC21sinC1=sinC1sinC2
5. Final Relation: Thus, we arrive at the relationship:
tanθ=sinC1sinC2
Conclusion:
The correct relation between the polarizing angle (θ) and the critical angles (C1 and C2) is:
tanθ=sinC1sinC2
tanθ=n2n1
n1sinC1=n2sin90∘
Since sin90∘=1, we can simplify this to:
n1=1sinC1
n2=1sinC2
tanθ=n2n1=1sinC21sinC1=sinC1sinC2
tanθ=sinC1sinC2
The correct relation between the polarizing angle (θ) and the critical angles (C1 and C2) is:
tanθ=sinC1sinC2
Similar Questions
Knowledge Check
Find the third angle of a triangle, two of whose angles are 65∘and71∘.
A84∘.B54∘.C44∘.D64∘.
If θ1 and θ2 be the apparent angles of dip observed in two vertical planes at right angles to each other, then the true angle of dip θ is given by
View SolutionHow is the critical angle related to the refractive index of a medium ?
View Solution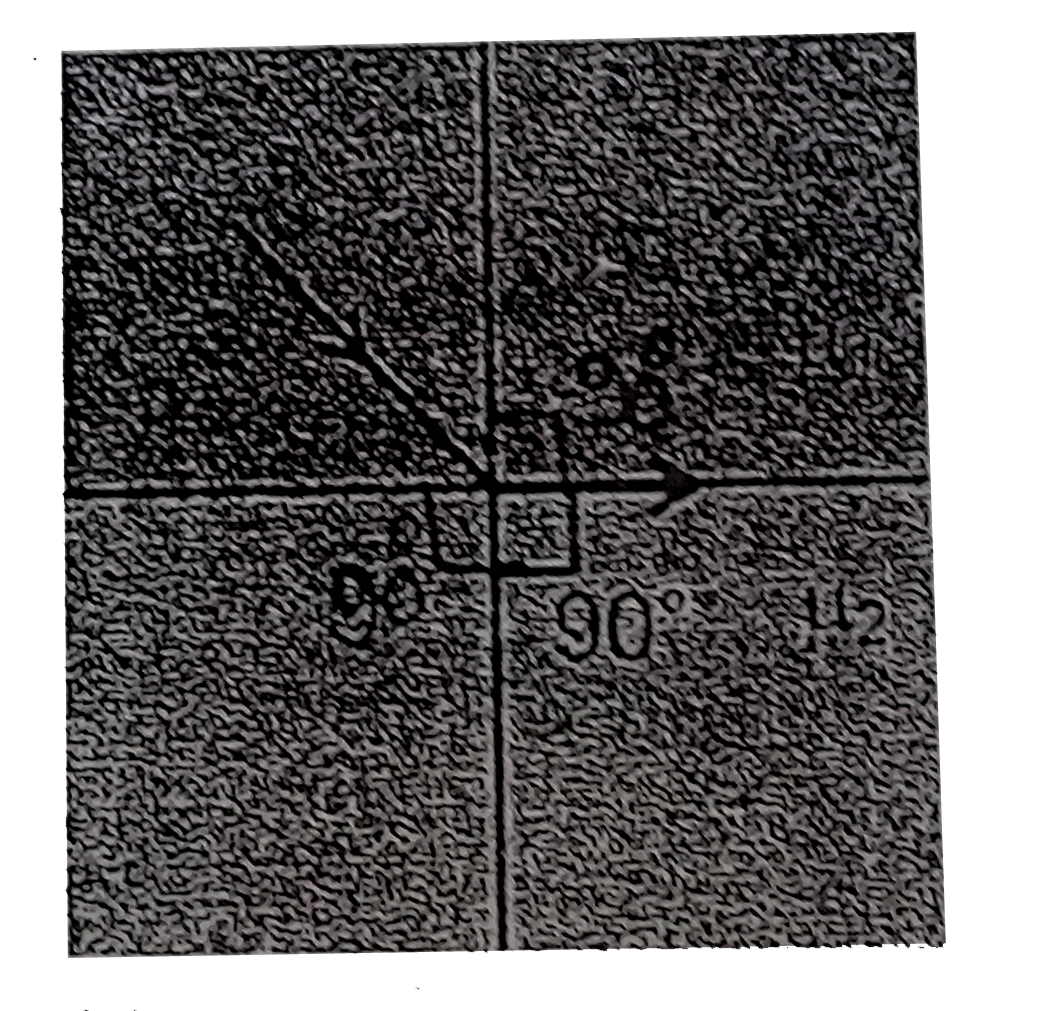
A ray is incident on interface of two media at critical angle as shown in the figure.
View SolutionWhen light travels from glass to air, the incident angle is θ1 and the refracted angle is θ2. The true relation is
View SolutionFind the angles of a triangle whose verties are A(3,2,1),B(35,2) and C(5,−2,3).
View SolutionThe angle of polarisation for any medium is 60∘ , what will be critical angle for this
View SolutionThe angle of polarisation for any medium is 60∘ , what will be critical angle for this
View Solution
