A spring balance and a physical balance are kept in a lift. In these balance equal masses are placed. If now the lift starts moving upward with constant acceleration, then.
Readings of A and B will be different at both the places
Readings of A will be different but those of B will be same at both the places
Readings of A will be same but those of B will be different at both the places
Readings of both A and B are same at both the places
The correct Answer is:B
To solve the problem, we need to analyze the behavior of both the spring balance and the physical balance when the lift is accelerating upward with a constant acceleration.
1. Understanding the Scenario:
- We have two types of balances: a spring balance and a physical balance, both placed in a lift.
- Equal masses (m) are placed on both balances.
2. For the Spring Balance:
- When the lift accelerates upward with an acceleration a, the effective weight acting on the mass in the spring balance changes.
- The gravitational force acting on the mass is mg (where g is the acceleration due to gravity).
- Additionally, there is a pseudo force acting downward due to the lift's acceleration, which is ma.
- Therefore, the total force acting on the mass in the spring balance is:
T=mg+ma=m(g+a)
- This means the reading on the spring balance will increase because the tension in the spring is greater than just the weight of the mass.
3. For the Physical Balance:
- The physical balance measures mass by comparing the weights on both pans.
- When the lift accelerates upward, both pans experience the same upward acceleration, and thus the pseudo forces acting on both sides cancel each other out.
- The effective weight on both sides remains mg because the pseudo forces do not affect the balance between the two sides.
- Therefore, the reading on the physical balance remains unchanged.
4. Conclusion:
- The reading on the spring balance will increase due to the upward acceleration of the lift.
- The reading on the physical balance will remain the same.
- Thus, the readings of the two balances will be different when the lift accelerates upward.
Final Answer:
- The reading of the spring balance (A) will be greater than the reading of the physical balance (B).
- We have two types of balances: a spring balance and a physical balance, both placed in a lift.
- Equal masses (m) are placed on both balances.
- When the lift accelerates upward with an acceleration a, the effective weight acting on the mass in the spring balance changes.
- The gravitational force acting on the mass is mg (where g is the acceleration due to gravity).
- Additionally, there is a pseudo force acting downward due to the lift's acceleration, which is ma.
- Therefore, the total force acting on the mass in the spring balance is:
T=mg+ma=m(g+a)
- This means the reading on the spring balance will increase because the tension in the spring is greater than just the weight of the mass.
- The physical balance measures mass by comparing the weights on both pans.
- When the lift accelerates upward, both pans experience the same upward acceleration, and thus the pseudo forces acting on both sides cancel each other out.
- The effective weight on both sides remains mg because the pseudo forces do not affect the balance between the two sides.
- Therefore, the reading on the physical balance remains unchanged.
- The reading on the spring balance will increase due to the upward acceleration of the lift.
- The reading on the physical balance will remain the same.
- Thus, the readings of the two balances will be different when the lift accelerates upward.
- The reading of the spring balance (A) will be greater than the reading of the physical balance (B).
Similar Questions
A block of mass m is placed on weighing machine and it is also attached with a spring balance. The whole system is placed in a lift as shown in figure. Left is moving with constant acceleration 10m/s2 in upward direction. The reading of the weighing machine is 500 N and the reading of spring balance is 300 N. Find the actual mass of the block in kg. (Takr g=10m/s2)
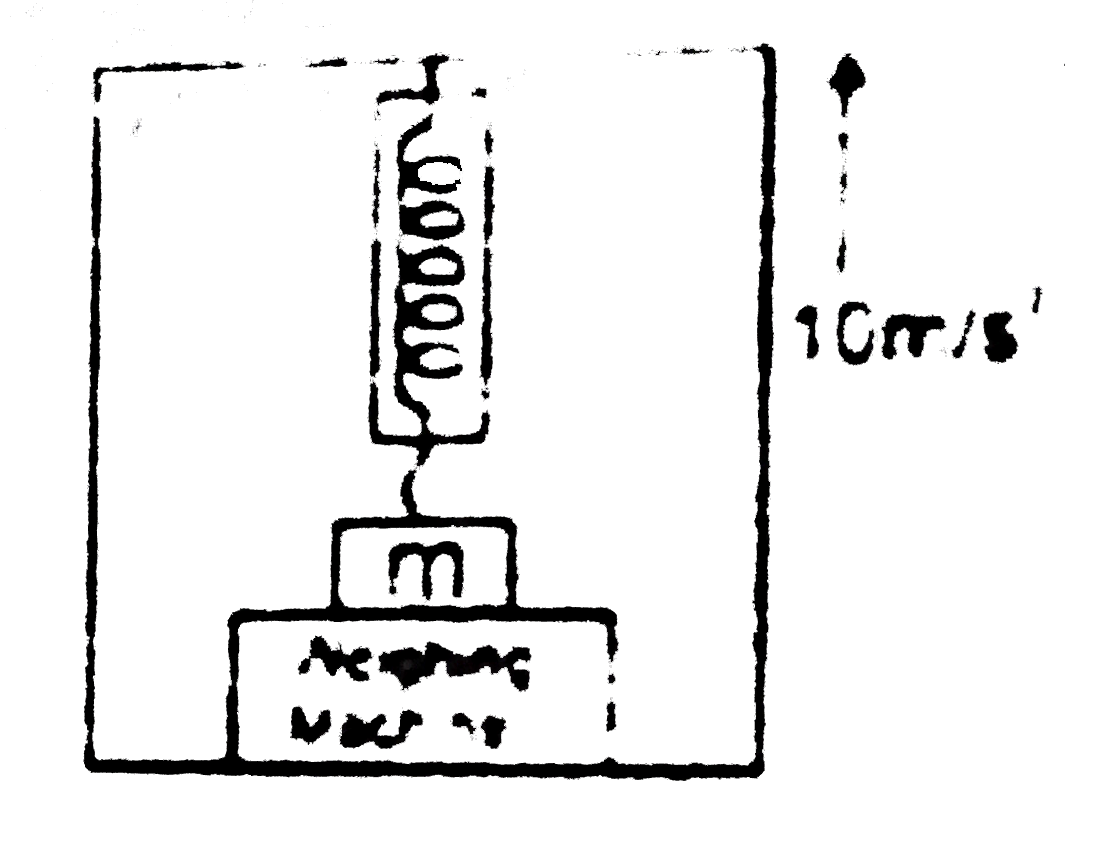
View SolutionA block of wood floats in a bucket of water placed in a lift. Will the block sink more or less if the lift starts accelerating up?
View SolutionA wooden block is floating on water kept in a beaker. 40% of the block is above the water surface. Now the beaker is kept inside a lift that starts going upward with acceleration equal to g/2. The block will then
View SolutionA lift can move upward or downward. A light inextensible string fixed from ceiling of lift when a frictionless pulley and tensions in string T1. Two masses of m1andm2 are connected with inextensible light string and tension in this string T2 as shown in figure. Read the questionbs carefully and answer
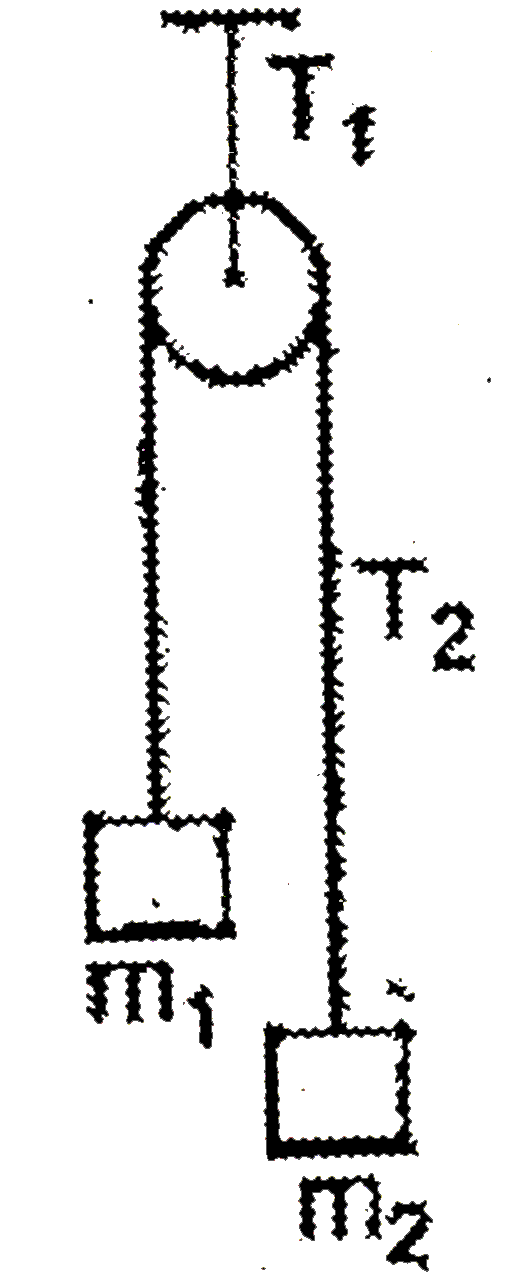
If m1=m2andm1 is moving at a certain instant with velocity v upward with respect to lift and the lift is moving in upward direction with constant acceleration (a<g) then speed of m2 with respect to lift :
View SolutionThe time period of a simple pendulum measured inside a stationary lift is found to be T . If the lift starts accelerating upwards with an acceleration g/3, the time period is
View SolutionA body of mass 50 kg is hung by a spring balance in a lift. Calculate the reading of the balance when :
The lift is ascending with an acceleration of 2m/s2.
View SolutionTime period of a spring pendulum when lift moves downward with constant velocity v is T second. When the lift moves upward with constant acceleration=g3 , the time period will be
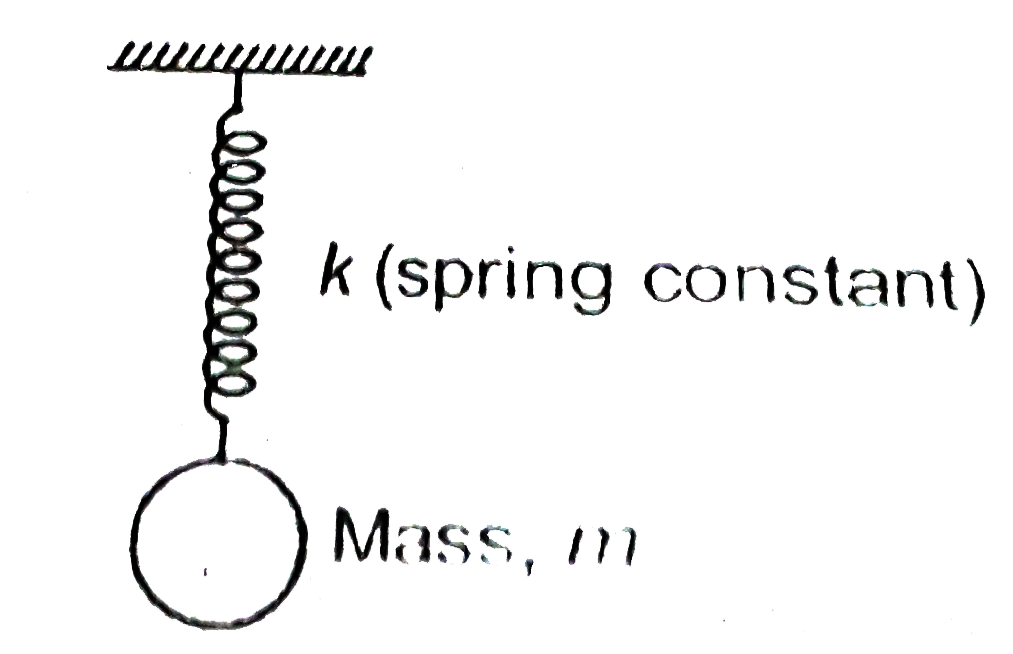
View SolutionThe time period of a simple pendulum inside a stationary lift is √5 s. What will be the time period when the lift moves upward with an acceleration g4?
View SolutionA spring balance is attached to the ceiling of a lift. A man hangs his bag on the spring and the spring reads 49N, when the lift is stationary. If the lift moves downward with an acceleration of 5m/22, the reading of the spring balance will be
View SolutionA spring balance is attached to the ceiling of a lift. A man hangs his bag on the spring and the spring reads 49N, when the lift is stationary. If the lift moves downward with an acceleration of 5m/22, the reading of the spring balance will be
View Solution